A composite tube is made by striking a thin steel tube on a brass tube. If AS and AB are the respective sectional areas of the steel and brass tubes and YS and YB their Young's moduli, then find the Young's modulus of single tube of the same length and total sectional area, which would behave in the same fashion as that of the composite tube.
To find the Young's modulus of a single tube that behaves the same way as a composite tube made of steel and brass, we can follow these steps:
1. Define the Variables:
- Let AS = sectional area of the steel tube
- Let AB = sectional area of the brass tube
- Let YS = Young's modulus of the steel tube
- Let YB = Young's modulus of the brass tube
- Let F = axial force applied to the composite tube
- Let L = original length of the tubes
- Let ΔL = elongation of the composite tube
2. Calculate Stress in Each Tube:
- The stress in the steel tube is given by:
σS=FSAS
- The stress in the brass tube is given by:
σB=FBAB
- The total force F is the sum of the forces in the steel and brass tubes:
F=FS+FB
3. Relate Stress and Strain:
- Since the strain in both tubes is the same (as they are in series), we have:
σSYS=σBYB
- Rearranging gives:
σB=σS⋅YBYS
4. Substitute Stress into Total Force Equation:
- Substitute σB into the total force equation:
F=σSAS+(σS⋅YBYS)AB
- Factor out σS:
F=σS(AS+YBYSAB)
5. Express Stress:
- Thus, we can express σS as:
σS=FAS+YBYSAB
6. Calculate Elongation of Composite Tube:
- The elongation ΔL of the composite tube can be expressed as:
ΔL=σSLYS=FLYS(AS+YBYSAB)
7. Determine the Elongation of a Single Equivalent Tube:
- For a single tube with total area AS+AB and Young's modulus Y, the elongation is:
ΔL=FLY(AS+AB)
8. Set the Two Elongations Equal:
- To find Y, set the elongation of the composite tube equal to that of the single tube:
FLYS(AS+YBYSAB)=FLY(AS+AB)
9. Solve for Young's Modulus Y:
- Cancel FL from both sides:
1YS(AS+YBYSAB)=1Y(AS+AB)
- Rearranging gives:
Y=YS(AS+YBYSAB)AS+AB
10. Final Expression for Young's Modulus:
- Thus, the Young's modulus Y of the single tube is:
Y=YSAS+YBABAS+AB
- Let AS = sectional area of the steel tube
- Let AB = sectional area of the brass tube
- Let YS = Young's modulus of the steel tube
- Let YB = Young's modulus of the brass tube
- Let F = axial force applied to the composite tube
- Let L = original length of the tubes
- Let ΔL = elongation of the composite tube
- The stress in the steel tube is given by:
σS=FSAS
- The stress in the brass tube is given by:
σB=FBAB
- The total force F is the sum of the forces in the steel and brass tubes:
F=FS+FB
- Since the strain in both tubes is the same (as they are in series), we have:
σSYS=σBYB
- Rearranging gives:
σB=σS⋅YBYS
- Substitute σB into the total force equation:
F=σSAS+(σS⋅YBYS)AB
- Factor out σS:
F=σS(AS+YBYSAB)
- Thus, we can express σS as:
σS=FAS+YBYSAB
- The elongation ΔL of the composite tube can be expressed as:
ΔL=σSLYS=FLYS(AS+YBYSAB)
- For a single tube with total area AS+AB and Young's modulus Y, the elongation is:
ΔL=FLY(AS+AB)
- To find Y, set the elongation of the composite tube equal to that of the single tube:
FLYS(AS+YBYSAB)=FLY(AS+AB)
- Cancel FL from both sides:
1YS(AS+YBYSAB)=1Y(AS+AB)
- Rearranging gives:
Y=YS(AS+YBYSAB)AS+AB
- Thus, the Young's modulus Y of the single tube is:
Y=YSAS+YBABAS+AB
Topper's Solved these Questions
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Solved Examples
11 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - Exercise 5.1
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
Similar Questions
Two vertical rods of equal lengths, one of steel and the other of copper, are suspended from the ceiling at a distance l apart and are connected rigidly to a rigid horizontal bar at their lower ends. If AS and AC be their respective cross-sectional areas, and YS and YC,their respective Young's moduli of elasticities, where should a vertical force F be applied to the horizontal bar in order that the bar remains horizontal?
View SolutionThe Young's modulus of steel is twice that of brass. Two wires of the same length and of the same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weight added to the steel and brass wires must be in the ratio of
View Solution
Knowledge Check
A steel wire of length 4.5 m and cross-sectional area 3×10−5m2 stretches by the same amount as a copper wire of length 3.5 m and cross-sectional area of 4x10−5m2 under a given load. The ratio of the Young's modulus of steel to that of copper is
A1.3B1.5C1.7D1.9A liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section A1 and A2 and v1 and v2 respectively. The differnece in the levels of the liquid in the two vertical tubes is h. then
 Av22−v21=2ghBv22+v21=2ghCv22−v21=ghDv22+v21=gh
Av22−v21=2ghBv22+v21=2ghCv22−v21=ghDv22+v21=gh
The water flowing through the same tube having two different cross section areas has different flow rates .
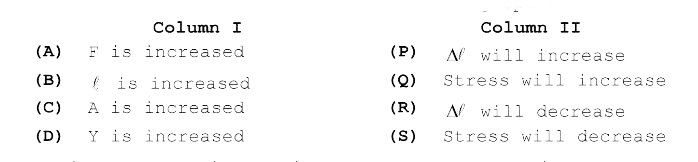
View SolutionA wire of length l, area of cross section A and Young’s modulus of elasticity Y is stretched by a longitudinal force F. The change in length is Δl Match the following two columns.
View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

View SolutionA steel wire of length 4.7 m and cross-section 3.0×102m2 stretches by the same amount as a copper wire of length 3.5 m and cross-section 4.0xx102m2 under a given load. What is the ratio of the Young.s modulus of steel to that of copper?
View SolutionA steel bolt is insertede into a copper tube as shown in the figure. Find the forces induced in the bolt and in the tube when the nut is turned through one revolution. Assume that the length of the tube is l, the pitch of the bolt thread is h and the cross sectional areas of the steel bolt and the copper tube are As and Ac respectively.

View SolutionA smooth uniform, string of natural length l, cross-sectional area A and Young’s modulus Y is pulled along its length by a force F on a horizontal surface. Find the elastic potential energy stored in the string.
View Solution
