The Young's modulus of steel is twice that of brass. Two wires of the same length and of the same area of cross section, one of steel and another of brass are suspended from the same roof. If we want the lower ends of the wires to be at the same level, then the weight added to the steel and brass wires must be in the ratio of
1:2
2:1
4:1
1:1
The correct Answer is:B
To solve the problem, we need to understand the relationship between Young's modulus, stress, and strain in the two wires made of steel and brass.
1. Understanding Young's Modulus:
- Young's modulus (Y) is defined as the ratio of stress (force per unit area) to strain (relative change in length).
- For two materials, we have:
Ys=FsLAΔLs(for steel)
Yb=FbLAΔLb(for brass)
- Given that Ys=2Yb.
2. Setting Up the Ratio:
- From the definition, we can express the ratio of Young's moduli:
YsYb=FsΔLbFbΔLs
- Since the lengths (L) and areas (A) of both wires are the same, they cancel out.
3. Substituting the Given Relation:
- We know that Ys=2Yb, thus:
YsYb=2⇒FsΔLbFbΔLs=2
4. Equal Elongation Condition:
- For the ends of the wires to be at the same level after elongation, the elongations must be equal:
ΔLs=ΔLb
- Therefore, we can simplify the equation:
FsFb=2
5. Interpreting Forces as Weights:
- The forces Fs and Fb correspond to the weights added to the steel and brass wires respectively:
Fs=WsandFb=Wb
- Thus, we have:
WsWb=2
6. Final Ratio:
- This means the weight added to the steel wire is twice that added to the brass wire:
Ws:Wb=2:1
Conclusion:
The ratio of the weights added to the steel and brass wires must be 2:1.
- Young's modulus (Y) is defined as the ratio of stress (force per unit area) to strain (relative change in length).
- For two materials, we have:
Ys=FsLAΔLs(for steel)
Yb=FbLAΔLb(for brass)
- Given that Ys=2Yb.
- From the definition, we can express the ratio of Young's moduli:
YsYb=FsΔLbFbΔLs
- Since the lengths (L) and areas (A) of both wires are the same, they cancel out.
- We know that Ys=2Yb, thus:
YsYb=2⇒FsΔLbFbΔLs=2
- For the ends of the wires to be at the same level after elongation, the elongations must be equal:
ΔLs=ΔLb
- Therefore, we can simplify the equation:
FsFb=2
- The forces Fs and Fb correspond to the weights added to the steel and brass wires respectively:
Fs=WsandFb=Wb
- Thus, we have:
WsWb=2
- This means the weight added to the steel wire is twice that added to the brass wire:
Ws:Wb=2:1
The ratio of the weights added to the steel and brass wires must be 2:1.
Similar Questions
Two wires of the same materical having equal area of cross-section have L and 2L. Their respective resistances are in the ratio
View SolutionTwo steel wires of the same radius have their lengths in the ratio of 1:2. If they are stretched by the same force, then the strains produced in the two wires will be in the ratio of
View SolutionThe young's modulus for steel is much more then that for rubber. For the same longitudinal strain, which one will have greater tensil stress ?
View SolutionA piece of copper wire has twice the radius of a piece of steel wire. Young's modulus for steel is twice that of the copper. One end of the copper wire is joined to one end of the steel wire so that both can be subjected to the same longitudinal force. By what fraction of its length will the steel have stretched when the length of the copper has increased by 1%?
View SolutionA piece of copper wire has twice the radius of a piece of steel wire. Young's modulus for steel is twice that of the copper. One end of the copper wire is joined to one end of the steel wire so that both can be subjected to the same longitudinal force. By what fraction of its length will the steel have stretched when the length of the copper has increased by 1%?
View SolutionTwo wires have identical lengths and areas of cross-section are suspended by mass M. Their Young's modulus are in the ratio 2:5.
View SolutionTwo wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is 1.5m and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
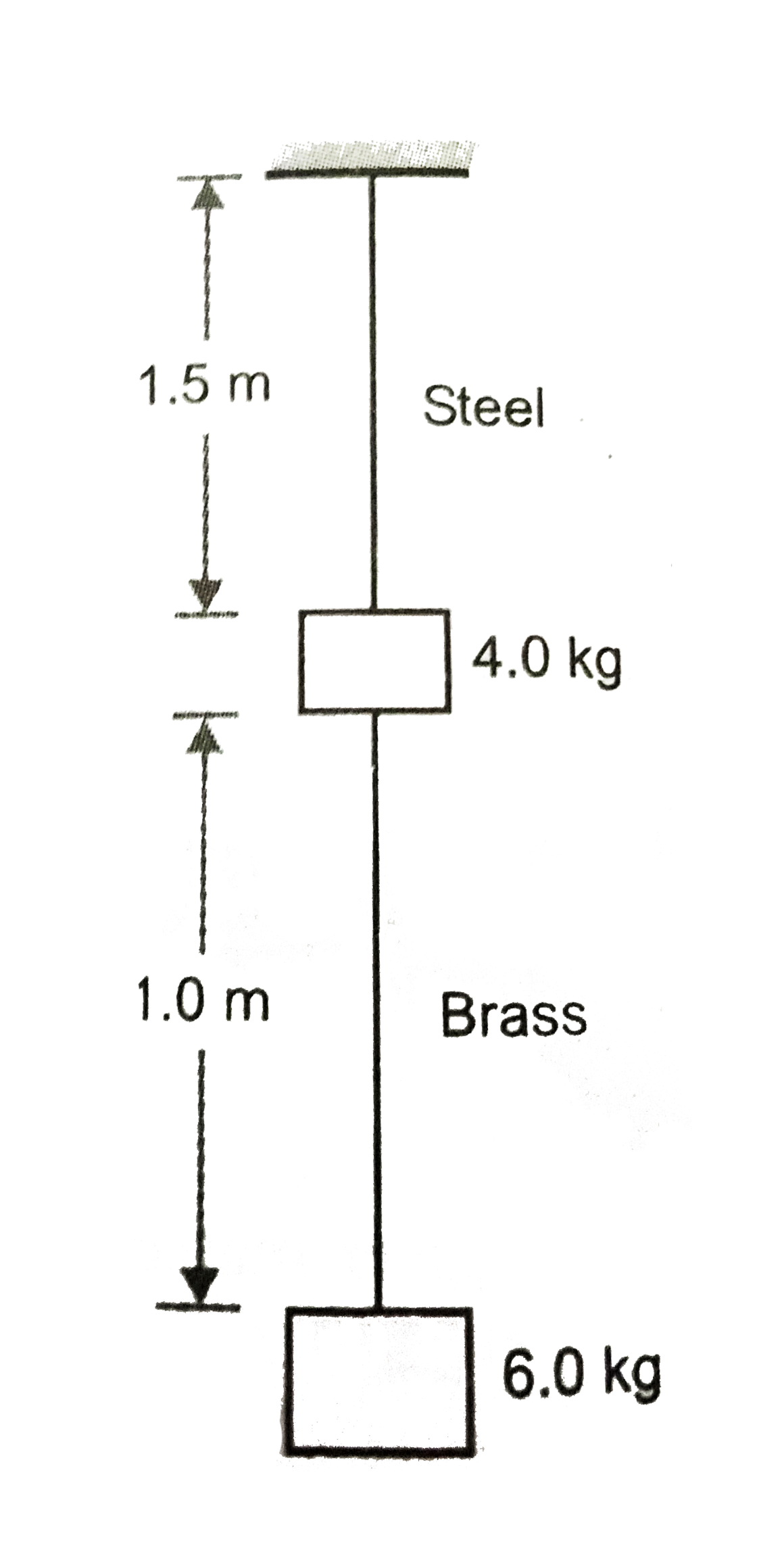
View SolutionIf two wires of same length l and are of the cross-section A with young modulus Y and 2Y connect in series and one end is fixed on roof and another end with mass m makes simple harmonic motion, then the time period is
View SolutionTwo wires A and B have the same cross section and are made of the same material, but the length of wire A is twice that of B. Then, for a given load
View SolutionTwo wires A and B have the same length and area of cross-secton. But Young's modulus of A is two times the Young's modulus of B. Then the ratio of force constant of A to that of B is
View Solution
