A point P lies on the axis of a fixed ring of mass M and radius a, at a distance a from its centre C. A small particle starts from P and reaches C under gravitational attraction only. Its speed at C will be.
zero
√2GMa
√2GMa(√2−1)
⎷2GMa(1−1√2)
The correct Answer is:D
To solve the problem, we will use the principle of conservation of mechanical energy. The total mechanical energy (potential energy + kinetic energy) of the particle remains constant as it moves under the influence of gravitational attraction.
1. Identify the Initial and Final Points:
- Initial Point (P): The particle starts from point P, which is at a distance a from the center C of the ring along the axis.
- Final Point (C): The particle reaches the center C of the ring.
2. Write the Conservation of Energy Equation:
- The total mechanical energy at point P (initial) is equal to the total mechanical energy at point C (final).
\(
\text{Initial Potential Energy} + \text{Initial Kinetic Energy} = \text{Final Potential Energy} + \text{Final Kinetic Energy}
\)
3. Calculate Initial Potential Energy:
- The gravitational potential energy \( U \) of the particle of mass \( m \) at point P due to the ring of mass \( M \) is given by:
\(
U_P = -\frac{GMm}{\sqrt{a^2 + a^2}} = -\frac{GMm}{\sqrt{2a^2}} = -\frac{GMm}{a\sqrt{2}}
\)
- The initial kinetic energy \( K_P \) is zero since the particle starts from rest:
\(
K_P = 0
\)
4. Calculate Final Potential Energy:
- At point C (the center of the ring), the potential energy \( U_C \) is:
\(
U_C = -\frac{GMm}{a}
\)
- The final kinetic energy \( K_C \) is given by:
\(
K_C = \frac{1}{2}mv^2
\)
5. Set Up the Equation:
- Using the conservation of energy:
\(
U_P + K_P = U_C + K_C
\)
Substituting the values:
\(
-\frac{GMm}{a\sqrt{2}} + 0 = -\frac{GMm}{a} + \frac{1}{2}mv^2
\)
6. Simplify the Equation:
- Cancel \( m \) from all terms (assuming \( m \neq 0 \)):
\(
-\frac{GM}{a\sqrt{2}} = -\frac{GM}{a} + \frac{1}{2}v^2
\)
- Rearranging gives:
\(
\frac{1}{2}v^2 = \frac{GM}{a} - \frac{GM}{a\sqrt{2}}
\)
7. Factor Out Common Terms:
- Factor out \( \frac{GM}{a} \):
\(
\frac{1}{2}v^2 = \frac{GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)
\)
8. Solve for \( v \):
- Multiply both sides by 2:
\(
v^2 = \frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)
\)
- Taking the square root:
\(
v = \sqrt{\frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)}
\)
Final Answer:
The speed of the particle at point C will be:
\(
v = \sqrt{\frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)}
\)
- Initial Point (P): The particle starts from point P, which is at a distance a from the center C of the ring along the axis.
- Final Point (C): The particle reaches the center C of the ring.
- The total mechanical energy at point P (initial) is equal to the total mechanical energy at point C (final).
\(
\text{Initial Potential Energy} + \text{Initial Kinetic Energy} = \text{Final Potential Energy} + \text{Final Kinetic Energy}
\)
- The gravitational potential energy \( U \) of the particle of mass \( m \) at point P due to the ring of mass \( M \) is given by:
\(
U_P = -\frac{GMm}{\sqrt{a^2 + a^2}} = -\frac{GMm}{\sqrt{2a^2}} = -\frac{GMm}{a\sqrt{2}}
\)
- The initial kinetic energy \( K_P \) is zero since the particle starts from rest:
\(
K_P = 0
\)
- At point C (the center of the ring), the potential energy \( U_C \) is:
\(
U_C = -\frac{GMm}{a}
\)
- The final kinetic energy \( K_C \) is given by:
\(
K_C = \frac{1}{2}mv^2
\)
- Using the conservation of energy:
\(
U_P + K_P = U_C + K_C
\)
Substituting the values:
\(
-\frac{GMm}{a\sqrt{2}} + 0 = -\frac{GMm}{a} + \frac{1}{2}mv^2
\)
- Cancel \( m \) from all terms (assuming \( m \neq 0 \)):
\(
-\frac{GM}{a\sqrt{2}} = -\frac{GM}{a} + \frac{1}{2}v^2
\)
- Rearranging gives:
\(
\frac{1}{2}v^2 = \frac{GM}{a} - \frac{GM}{a\sqrt{2}}
\)
- Factor out \( \frac{GM}{a} \):
\(
\frac{1}{2}v^2 = \frac{GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)
\)
- Multiply both sides by 2:
\(
v^2 = \frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)
\)
- Taking the square root:
\(
v = \sqrt{\frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)}
\)
The speed of the particle at point C will be:
\(
v = \sqrt{\frac{2GM}{a}\left(1 - \frac{1}{\sqrt{2}}\right)}
\)
Topper's Solved these Questions
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Multiple Correct
24 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Assertion- Reasoning
13 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Subjective
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
Similar Questions
A point P(R√3,0,0) lies on the axis of a ring of mass M and radius R. The ring is located in yx-plane with its centre at origin O. A small particle of mass m starts from P and reaches O under gravitational attraction only. Its speed at O will be
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View Solution
Knowledge Check
A mass m is placed at point P lies on the axis of a ring of mass M and radius R at a distance R from its centre. The gravitational force on mass m is
AGMm√2R2BGMm2R2CGMm2√2R2DGMm4R2
Draw two tangents to a circle of radius 3.5 cm from a point P at a distance of 6.2 cm from its centre.
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionA point particle of mass m is released from a distance √3R along the axis of a fixed ring of mass M and radius R as shown in figure. Find the speed of particle as it passes through the centre of the ring.

View SolutionFind the intensity of gravitational field at a point lying at a distance x from the centre on the axis of a ring of radius a and mass M.
View Solution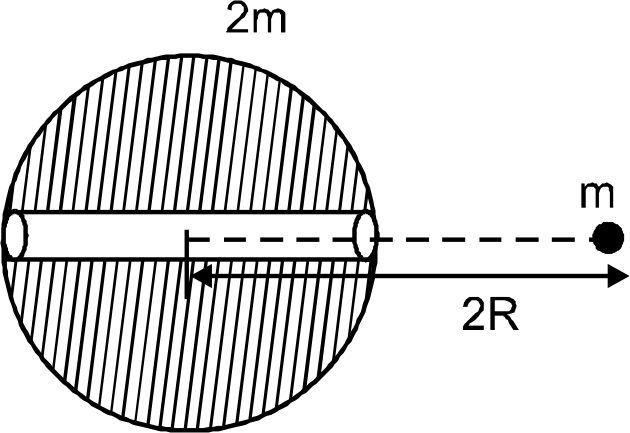
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View Solution
