Question

Mark the correct statements.

Options

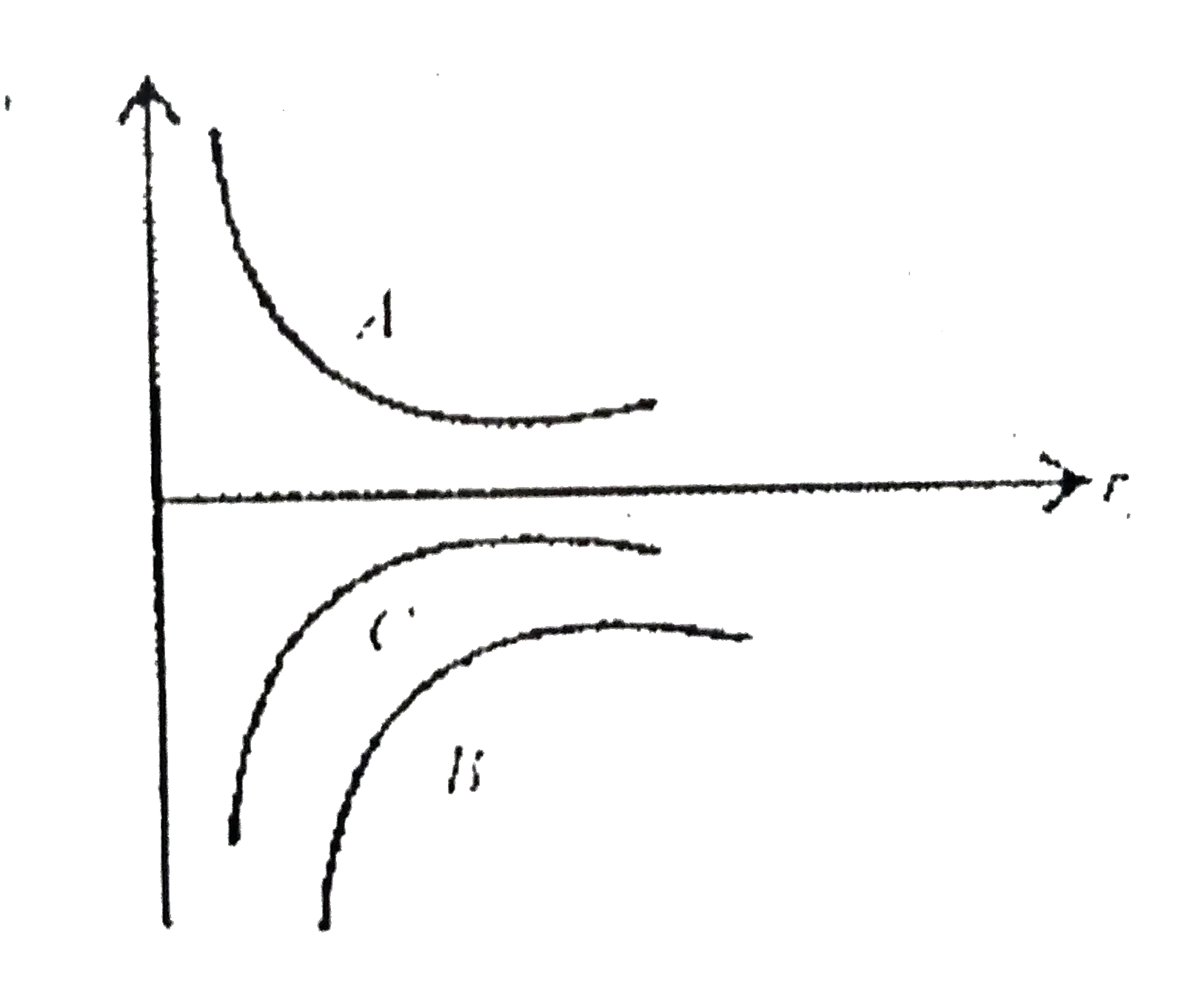
A. Gravitational potential at the centre of curvature of a thin hemispherical shell of radius `R` and mass `M` is equal to `GM//R`.
B. Gravitational field strength at a point lying on the axis of a thin, uniform circular ring of radius R and mass M is equal to `GMx//[(R^(2)+x^(2))^(3/2)]` where `x` is distance of that point from the centre of the ring.
C. Newton's law of gravitation for gravitational force between two bodies is applicable only when bodies have spherical symmetric distribution of mass.
D. None of these

Answer

B::C

Explanation

Since every element of the hemispherical shell is at a potential `R` from thecentre of curvature, therefore gravitational potential at its centre `=-GM//R`. It is not equal to `GM//R`. Hence, option a is incorrect.
Gravitational field strength at a point, lying on the axis of a thin uniform circular ring of radius `R`, can be calculated by considering two equal elemental lengths of the ring such that these considering two equal elemental lengths of the ring such that these are length lying on the ends of a diameter and thus the expression given in option b is obtained. Hence, option b is correct.
Newton's law of gravitation is applicable to only those bodies which have spherically symmertric distribution of mass. For example, if we consider two solid hemispheres of equal radii, one made of wood and the other made of iron. These two hemispheres are joined together to form a shape of complete sphere we cannot apply Newton's gravitational law of this sphere, so option c is correct.