A small sphere of mass m=0.6kg carrying a positive charge q=80μC is connected with a light, flexible, and inextensible string of length r=30cm and whirled in a vertical circle. If a horizontal rightward electric field of strength E=105NC−1 exists in the space, calculate the minimum velocity of the sphere required at the highest point so that it may just complete the circle (g=10ms−2).
To solve the problem, we need to find the minimum velocity of a sphere at the highest point of a vertical circle so that it can complete the circle under the influence of gravity and an electric field.
1. Identify the Known Values:
- Mass of the sphere, m=0.6kg
- Charge of the sphere, q=80μC=80×10−6C
- Length of the string (radius of the circle), r=30cm=0.3m
- Electric field strength, E=105N/C
- Acceleration due to gravity, g=10m/s2
2. Calculate the Electric Force:
The electric force acting on the sphere due to the electric field is given by:
FE=qE=(80×10−6C)(105N/C)=8N
3. Determine the Forces at the Highest Point:
At the highest point of the circle, the forces acting on the sphere are:
- The gravitational force Fg=mg=0.6×10=6N acting downwards.
- The electric force FE=8N acting horizontally to the right.
4. Centripetal Force Requirement:
At the highest point, the centripetal force required to keep the sphere moving in a circle is provided by the net downward force. The centripetal force Fc is given by:
Fc=mv2r
where v is the velocity at the highest point.
5. Net Force at the Highest Point:
The net downward force acting on the sphere is the sum of the gravitational force and the vertical component of the electric force. Since the electric force is horizontal, we only consider the gravitational force for centripetal motion:
Fnet=mg=6N
6. Set Up the Equation:
For the sphere to just complete the circle, the centripetal force must equal the gravitational force:
mv2r=mg
Rearranging gives:
v2=rg
7. Substituting Values:
Substitute r=0.3m and g=10m/s2:
v2=(0.3)(10)=3
v=√3≈1.73m/s
8. Conclusion:
The minimum velocity of the sphere required at the highest point to just complete the circle is approximately:
v≈1.73m/s
- Mass of the sphere, m=0.6kg
- Charge of the sphere, q=80μC=80×10−6C
- Length of the string (radius of the circle), r=30cm=0.3m
- Electric field strength, E=105N/C
- Acceleration due to gravity, g=10m/s2
The electric force acting on the sphere due to the electric field is given by:
FE=qE=(80×10−6C)(105N/C)=8N
At the highest point of the circle, the forces acting on the sphere are:
- The gravitational force Fg=mg=0.6×10=6N acting downwards.
- The electric force FE=8N acting horizontally to the right.
At the highest point, the centripetal force required to keep the sphere moving in a circle is provided by the net downward force. The centripetal force Fc is given by:
Fc=mv2r
where v is the velocity at the highest point.
The net downward force acting on the sphere is the sum of the gravitational force and the vertical component of the electric force. Since the electric force is horizontal, we only consider the gravitational force for centripetal motion:
Fnet=mg=6N
For the sphere to just complete the circle, the centripetal force must equal the gravitational force:
mv2r=mg
Rearranging gives:
v2=rg
Substitute r=0.3m and g=10m/s2:
v2=(0.3)(10)=3
v=√3≈1.73m/s
The minimum velocity of the sphere required at the highest point to just complete the circle is approximately:
v≈1.73m/s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Examples9videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.123videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q2videos
ELECTRICAL MEASURING INSTRUMENTS
Similar Questions
A small sphere of mass m=0.5kg carrying a positive charge q=110μC is connected with a light, flexible, and inextensible string of length of length r=60cm and whirled in a vertical circle. If a vertically upward electric field of strength E=105NC−1 exists in the space, calculate the minimum velocity of the sphere required at the highest point so that it may just complete the circle (g=10ms−2).
View SolutionTwo small particles A and B having masses m=0.5kg each and charges q1=(−155/18μC) and q2=(+100μC), respectively, are connected at the ends of a nonconducting, flexible, and inextensible string of length r=0.5m.
Particle A is fixed and B is whirled along a vertical circle with center at A. if a vertically upward electric field of Strength E=1.1×105NC−1 exists in the space, calculate the minimum velocity of particle B required at the highest point so that it may just complete the circle (g=10ms−2).
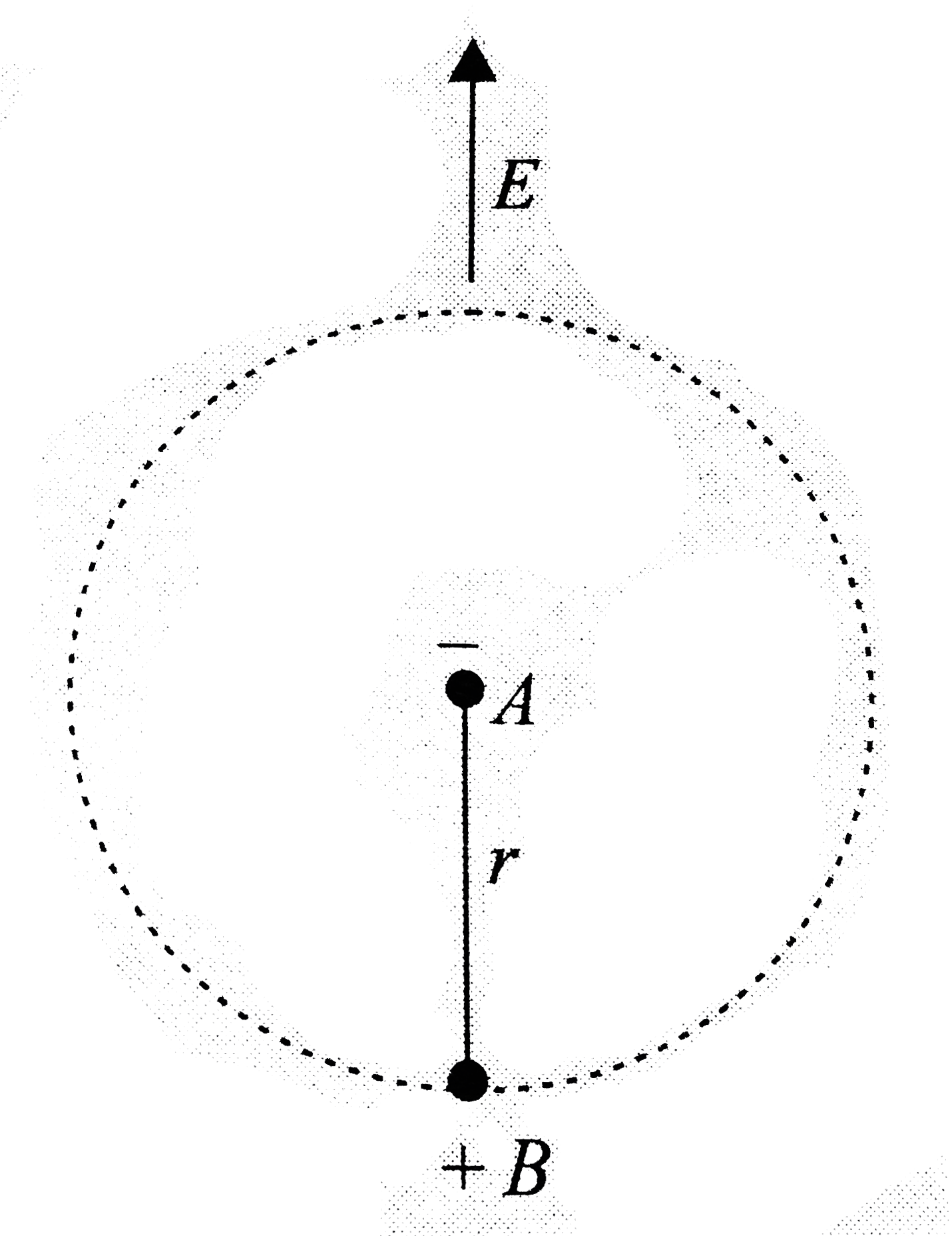 .View Solution
.View SolutionA stone of mass 2.0 kg is tied to the end of a string of 2m length. It is whirled in a horizontal circle. If the breaking tension of the string is 400 N, calculate the maximum velocity of the stone.
View SolutionA particle of mass m=0.1kg and having positive charge q=75μC is suspended from a point by a thread of length l=10cm. In the space, a uniform horizontal electric field E=104NC−1 exists. The particle is drawn aside so that the thread becomes vertical and then it is projected horizontally with velocity v such that the particle starts to move along a circle with the same constant speed V. Calculate the radius of the circle and speed v(g=10ms−2).
View SolutionA stone of 1 kg tied up with 10/3 m long string rotated in a vertical circle. If the ratio of maximum and minimum tension in string is 4 then speed of stone at highest point of circular path will be ( g=10ms2)
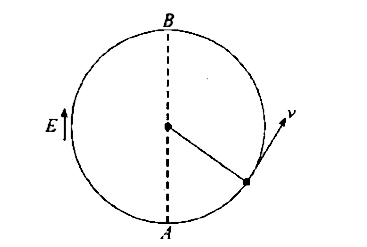
View SolutionA small ball of masss m and charge +q tied with a string of length l, rotating in a veticle circle under gravity and a uniform horizontal electric field E as shown. The tension in the string will be minimum for
 View Solution
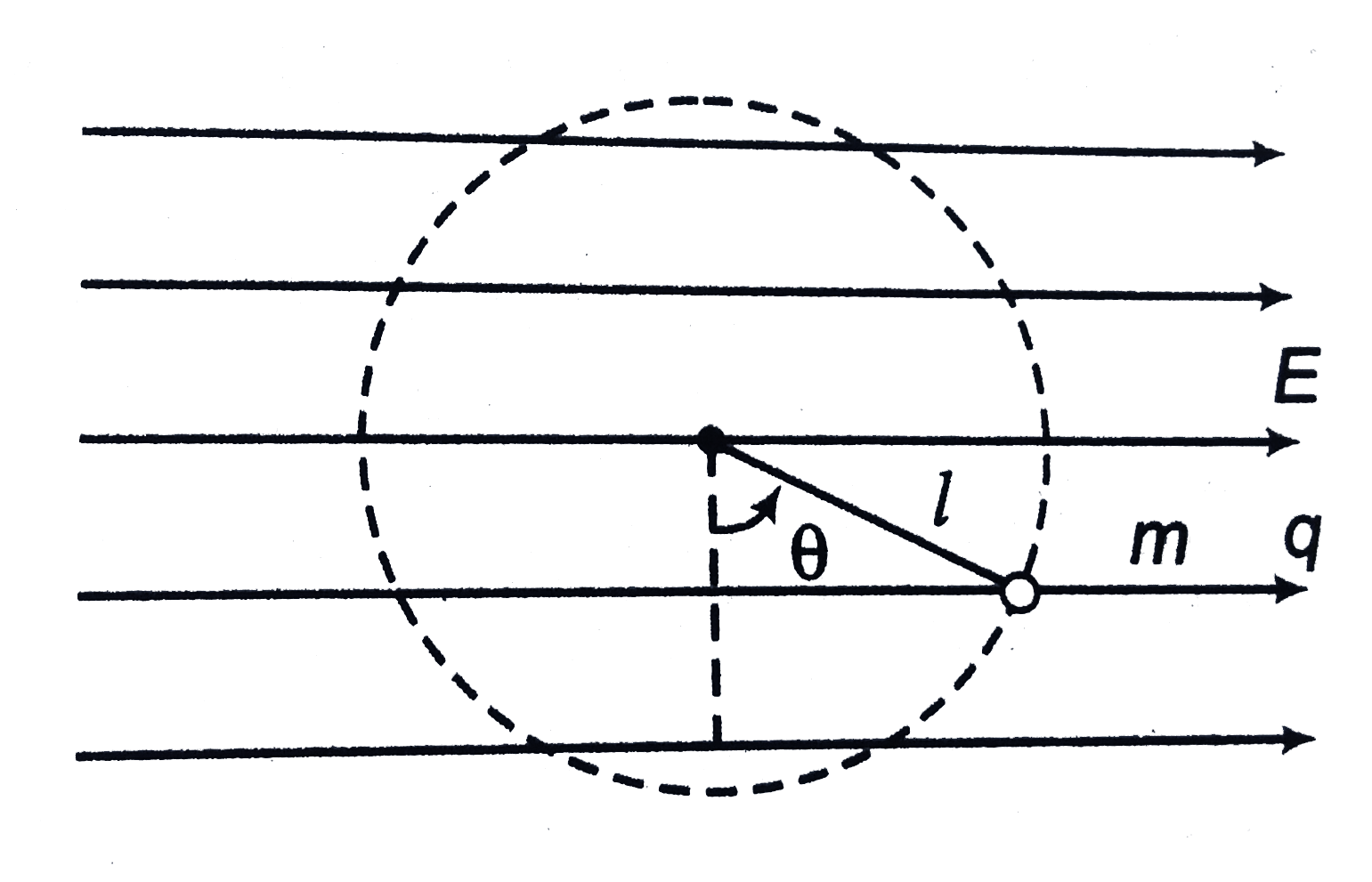
View SolutionA particle of mass m and charge Q is attached to a string of length l. It is whirled in a vertical circle in the region of an electric field E as shown in the figure-5.105.What is the speed given to the particle at the point B,so that tension in the string when the particle is at A is ten times the weight of the particle?
 View Solution
View SolutionA cabin is moving upwards with a constant acceleration g. A boy standing in the cabin wants to whirl a particle of mass m in a vertical circle of radius l. (Mass is attached to an ideal string.) Calculate minimum velocity which should be provided at lowermost point (w.r.t cabin) so that particle can just complete the circle.
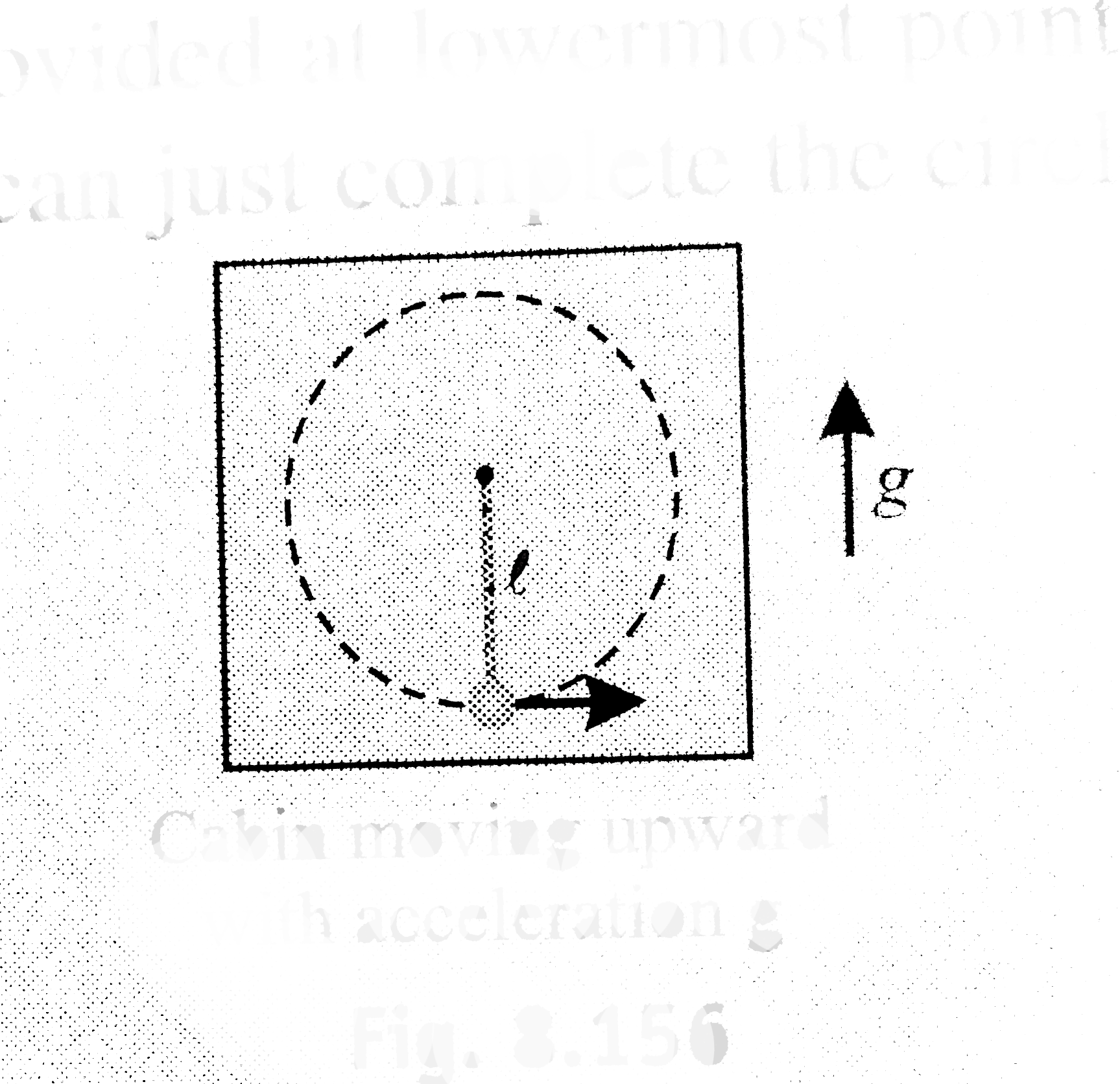 View Solution
View SolutionA small particle of mass m attached with a light inextensible thread of length L is moving in a vertical circle. In the given case particle is moving in complete vertical circle and ratio of its maximum to minimum velocity is 2:1 .
Kinetic energy of the particle at the lower most position is
 View Solution
View SolutionA charged particle of mass m = 2 kg and charge 1μC is projected from a horizontal ground at an angle θ=45∘ with speed 10ms−1. In space, a horizontal electric field towards the direction of projection E=2×107NC−1 exists. The range of the projectile is
View Solution

