We have an isolated conducting spherical shell of radius 10cm. Some positive charge is given to it so that the resulting electric field has a maximum intensity of 1.8×106NC−1. The same amount of negative charge is given to another isolated conducting spherical shell of radius 20cm. Now, the first shell is placed inside the second so that both are concentric as shown in (Fig. 3.154).
 .
.
The electric field intensity just inside the outer sphere.
4.5×105NC−1
9×105NC−1
4.5×104NC−1
5×104NC−1
The correct Answer is:A
1.8×106=9×109×q1(0.1)2orq1=2×10−6C
V=9×109×2×10−10[10.1−10.2]=9×104V
E=kq1r2=9×109×2×10−6(0.20)2=4.5×105NC−1
Interaction energy is
U=kq0.2(−q)=9×109×(2×10−6)20.2=−0.18J
Self - energies are
U1=q28πε0r1=9×109×(2×10−6)22×0.1=0.18J
U2=q28πε0r2=9×109×(2×10−6)22×0.2=0.09J
Total energy is U+U1+U2=0.09J
Both the charges will get neutralized and there will be no charge left on any sphere. So no energy will be left in the system. It means whole amount of energy will convert into heat.
 .
.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Integer
5 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.1
14 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - Multiple Correct
10 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
We have an isolated conducting spherical shell of radius 10cm. Some positive charge is given to it so that the resulting electric field has a maximum intensity of 1.8×106NC−1. The same amount of negative charge is given to another isolated conducting spherical shell of radius 20cm. Now, the first shell is placed inside the second so that both are concentric as shown in (Fig. 3.154).
 .
.
The electric potential at any point inside the first shell is.
View SolutionWe have an isolated conducting spherical shell of radius 10cm. Some positive charge is given to it so that the resulting electric field has a maximum intensity of 1.8×106NC−1. The same amount of negative charge is given to another isolated conducting spherical shell of radius 20cm. Now, the first shell is placed inside the second so that both are concentric as shown in (Fig. 3.154).
 .
.
The electrostatic energy stored in the system is.
View SolutionWe have an isolated conducting spherical shell of radius 10cm. Some positive charge is given to it so that the resulting electric field has a maximum intensity of 1.8×106NC−1. The same amount of negative charge is given to another isolated conducting spherical shell of radius 20cm. Now, the first shell is placed inside the second so that both are concentric as shown in (Fig. 3.154).
 .
.
If both the spheres are connected by a conducting wire, then.
View SolutionA 10 C charge is given to a conducting spherical shell, and a-3 C point charge is placed inside the shell. For this arrangement, find the correct statement(s).
View SolutionIn side a hollow isolated spherical shell-
View SolutionTwo concentric metallic spherical shells are given positive charges . Then
View SolutionA uniformly charged non-conducting spherical shell is given +q charge and a point charge -q is placed as shown. The electric field lines will be

View SolutionA spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
View SolutionA point positive charge is brought near an isolated conducting sphere as shown in figure the electric field is best given by
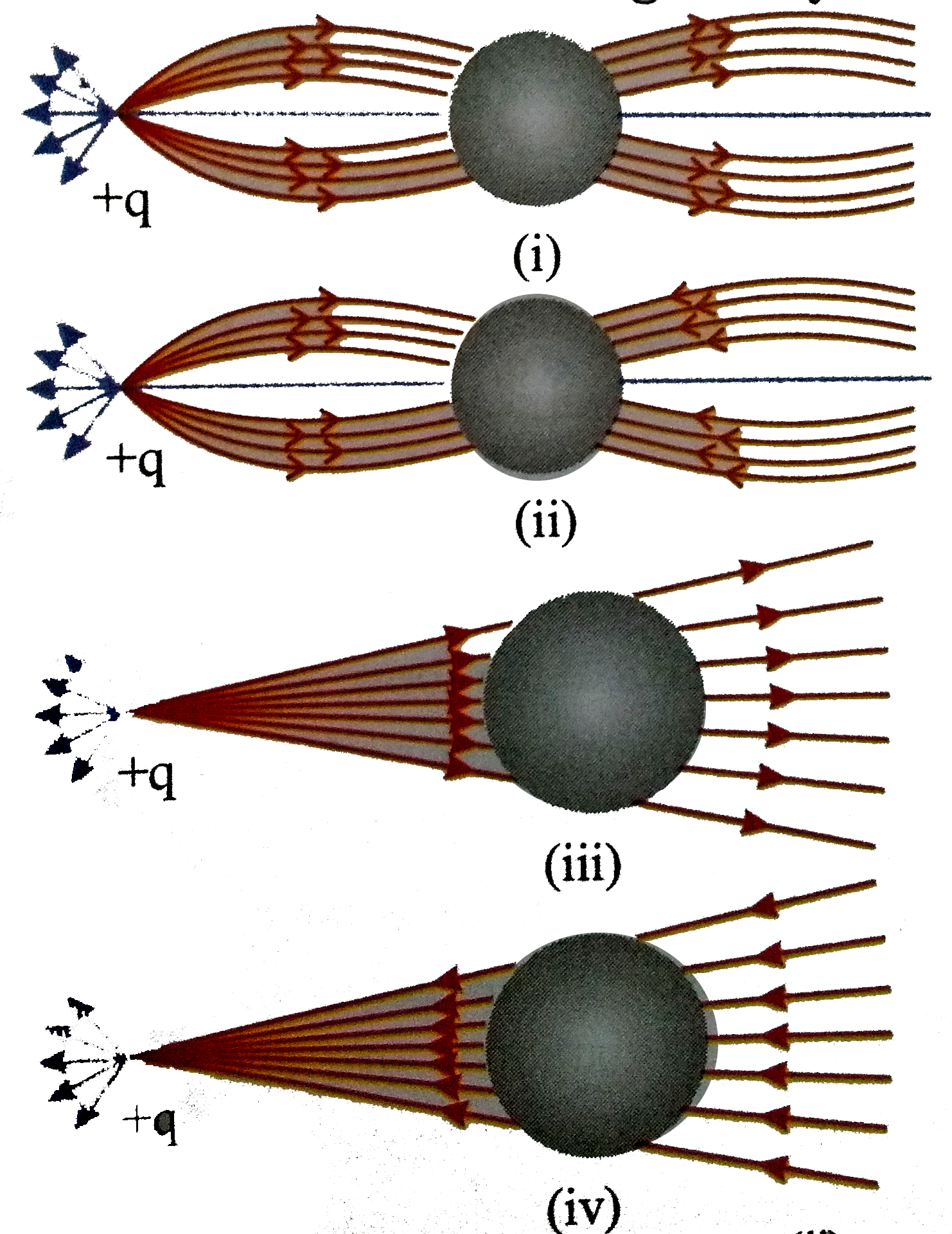
View SolutionA point positive charge is brought near an isolated conducting sphere. The electric field is best given by
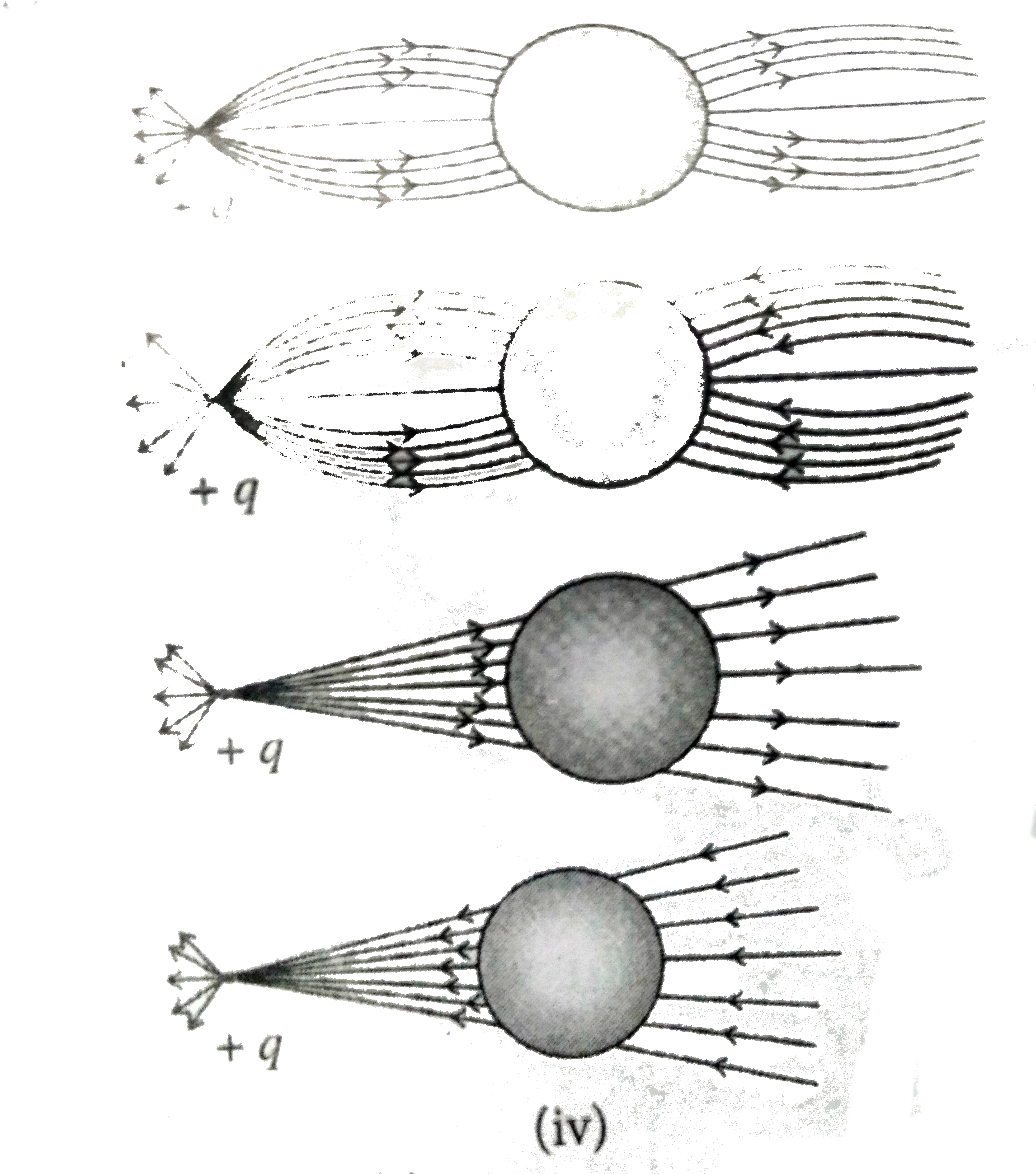
View Solution
