A conductor is an extreme case of a dielectric, because if an electric field is applied to a conductor, charges are free to move within the conductor to set up ''induced charges''. What is the dielectric constant of a perfect conductor? Is it , or something in between ? Explain your reasoning.
To determine the dielectric constant of a perfect conductor, we can follow these steps:
Step 1: Understand the Concept of Dielectric Constant
The dielectric constant (K) of a material is defined as the ratio of the electric field in a vacuum (E₀) to the electric field in the material (E) when an external electric field is applied. Mathematically, it is expressed as:
K=E0E
Step 2: Analyze the Behavior of a Perfect Conductor
In a perfect conductor, when an external electric field is applied, the free charges within the conductor move in response to the field. This movement of charges creates an induced electric field that opposes the applied electric field, leading to a situation where the net electric field inside the conductor becomes zero.
Step 3: Set the Electric Field Inside the Conductor
Since the electric field inside a perfect conductor is zero (E = 0), we can substitute this into the equation for the dielectric constant:
K=E0E=E00
Step 4: Evaluate the Expression
The expression E00 indicates that we are dividing a non-zero quantity (the electric field in vacuum) by zero. In physics, this division implies that the dielectric constant approaches infinity:
K→∞
Step 5: Conclusion
Thus, the dielectric constant of a perfect conductor is infinite. This means that a perfect conductor can completely shield its interior from any external electric field.
Final Answer
The dielectric constant (K) of a perfect conductor is infinite.
---
The dielectric constant (K) of a material is defined as the ratio of the electric field in a vacuum (E₀) to the electric field in the material (E) when an external electric field is applied. Mathematically, it is expressed as:
K=E0E
In a perfect conductor, when an external electric field is applied, the free charges within the conductor move in response to the field. This movement of charges creates an induced electric field that opposes the applied electric field, leading to a situation where the net electric field inside the conductor becomes zero.
Since the electric field inside a perfect conductor is zero (E = 0), we can substitute this into the equation for the dielectric constant:
K=E0E=E00
The expression E00 indicates that we are dividing a non-zero quantity (the electric field in vacuum) by zero. In physics, this division implies that the dielectric constant approaches infinity:
K→∞
Thus, the dielectric constant of a perfect conductor is infinite. This means that a perfect conductor can completely shield its interior from any external electric field.
The dielectric constant (K) of a perfect conductor is infinite.
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICS ENGLISHChapter:CAPACITOR AND CAPACITANCEExercise:Subjective
Explore 24 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICS ENGLISHChapter:CAPACITOR AND CAPACITANCEExercise:Single Correct
Explore 49 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICS ENGLISHChapter:CAPACITOR AND CAPACITANCEExercise:Exercise 4.1
Explore 13 Videos
- ATOMIC PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:ATOMIC PHYSICSExercise:ddp.4.3
Explore 15 Videos
- CENGAGE PHYSICS DPPBook:CENGAGE PHYSICS ENGLISHChapter:CENGAGE PHYSICS DPPExercise:subjective type
Explore 51 Videos
Similar Questions
Assertion : Electric field inside a conductor is 0.
Reason : Charge is present on surface of conductor.
View SolutionAssetrion: Net electric field insider conductor is zero
Reason: Total positive charge equals to total negative charge in a conductor
View SolutionIn a metal in the solid state, such as a copper wire, the atoms are strongly bound to one another and occupý fixed positions. Some electrons (called the conductor electrons) are free to move in the body of the metal while the other are strongly bound to their atoms. In good conductors, the number of free electrons is very large of the order of 1028 electrons per cubic metre in copper. The free electrons are in random motion and keep colliding with atoms. At room temperature, they move with velocities of the order of 105 m/s. These velocities are completely random and there is not net flow of charge in any directions. If a potential difference is maintained between the ends of the metal wire (by connecting it across a battery), an electric field is set up which accelerates the free electrons: These accelerated electrons frequently collide with the atoms of the conductor, as a result, they acquire a constant speed called the drift speed which is given by Ve= 1/enA where I = current in the conductor due to drifting electrons, e = charge of electron, n = number of free electrons per unit volume of the conductor and A = area of cross-section of the conductor. A constant potential difference is maintained between the ends of a conductor having nonuniform cross-section. Which of the following quantities will not change along the length of the conductor
View SolutionStatement I: If an electric field is applied to a metallic conductor, the free electrons experience a force but do not accelerate, they only drift at a constant speed.
Statement II: The force exerted by the electric field is completely balanced by the Coulomb force between electrons and protons .
View SolutionAssertion : In a cavity within a conductor, the electric field is zero.
Reason : Charges in a conductor reside only at its surface.
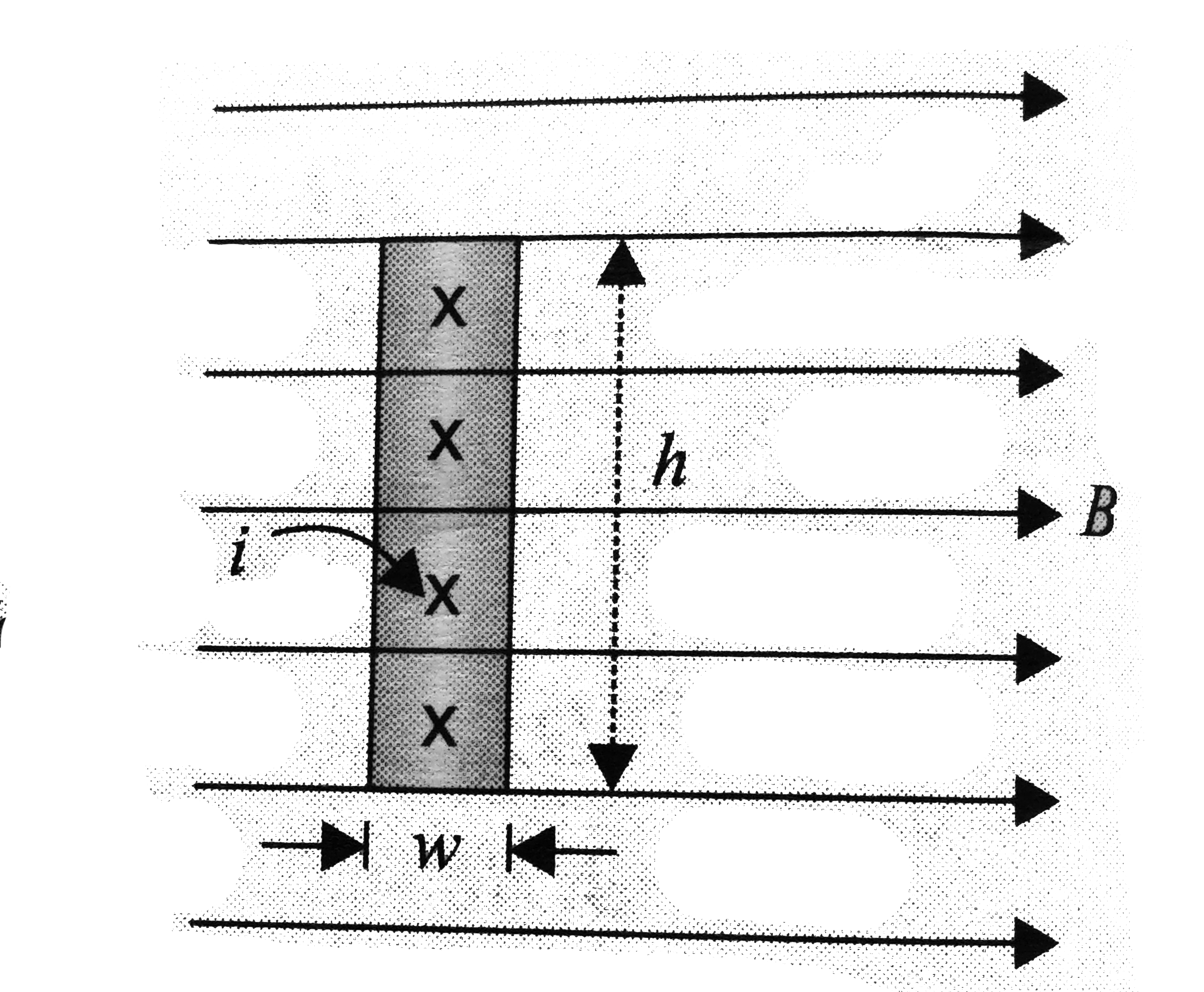
View SolutionA current i, indicated by the crosses in figure, is established in a strip of copper of height h and width w. A uniform field of magnetic induction B is applied at right angle to the strip.
(a) Calculate the drift velocity vd of the electrons.
(b) What are the magnitude and direction of the magnetic force F acting on the electrons?
(c) What should the magnitude and direction of a homogeneous electric field E be in order to counterbalance the effect of mangetic field?
(d) Calculate voltage V necessary between two sides of the conductor in order to creat this field E Between which sides of the conductor would this voltage have to be applied?
(e) If no electric field is applied from the outside, the electrons will be pushed somewhat to one side and therefore will give rise to a uniform electric field EH across the conductor until the forces of this electrostatic field EH balance the magnetic forces encountered in part (b). What will be the magnitude and direction of field EH?
Assume that n, the number of conductor electrons per unit volume is 1.1×1029m−3,h=0.02m,w=0.1cm,i=50A,andB=2T.
View SolutionA parallel plate capacitor has a dielectric (of dielectric constant k) between the plates. The plates are charged to a surface charge density σ0. What is the density of the polarized charge that appear on the surface of the dielectric.
View SolutionA spherical charged conductor has surface density of charge as σ. The electric field intensity on its surface is E. If the radius of the surface is doubled, keeping σ uncharged, what will be the electric field intensity on the new sphere ?
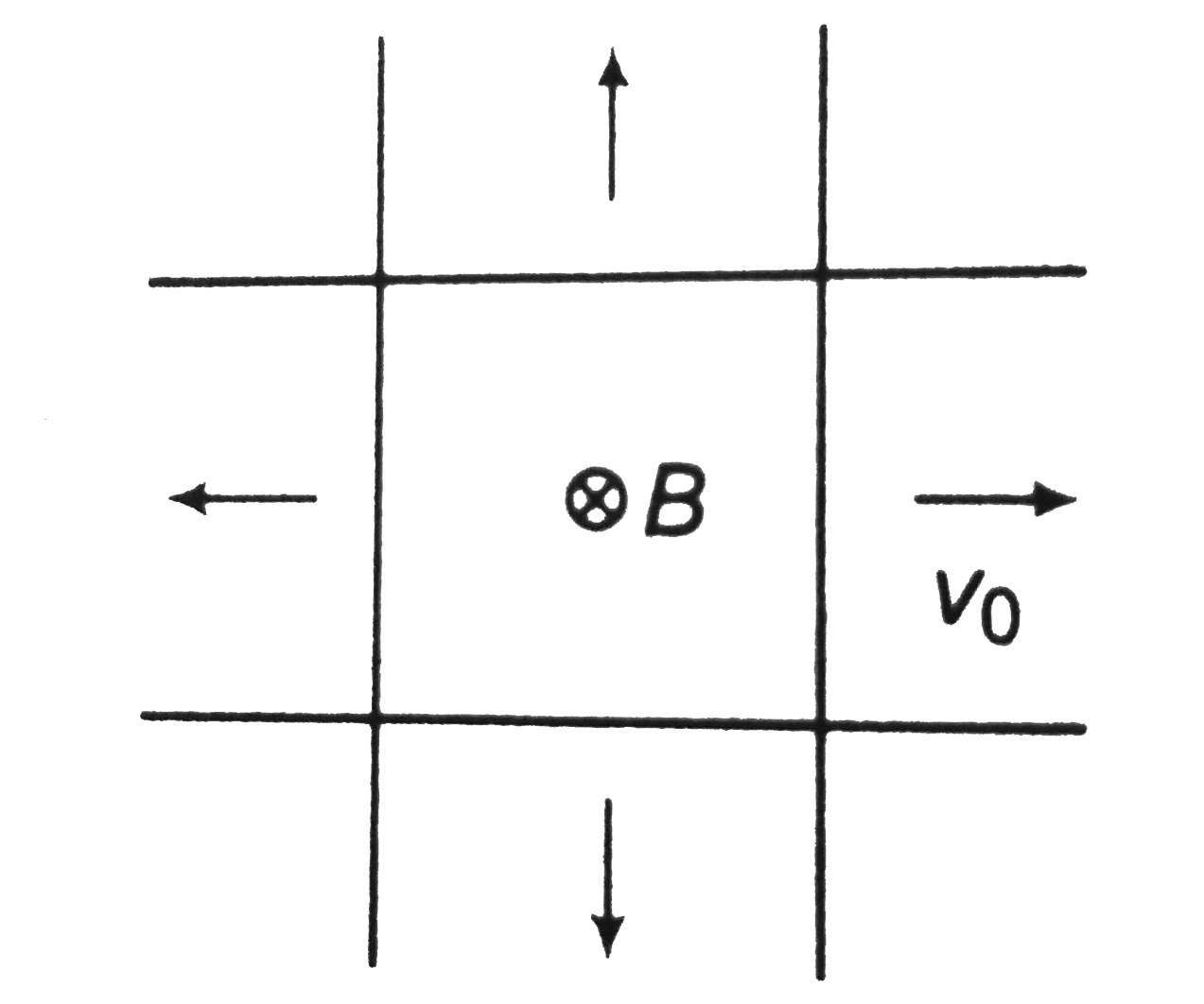
View SolutionTwo parallel long straight conductors lie on a smooth plane surface. Two other parallel conductors rest on them at right angle so as to form a square of side a. A uniform magnetic field B exists at right angle to the plane containing the conductors. Now, conductors starts moving outward witha constant velocity v0 at t=0. Then, induced current in the loop at any time is (λ is resistance per unit length of the conductors)
View SolutionIn a metal in the solid state, such as a copper wire, the atoms are strongly bound to one another and occupý fixed positions. Some electrons (called the conductor electrons) are free to move in the body of the metal while the other are strongly bound to their atoms. In good conductors, the number of free electrons is very large of the order of 1028 electrons per cubic metre in copper. The free electrons are in random motion and keep colliding with atoms. At room temperature, they move with velocities of the order of 105 m/s. These velocities are completely random and there is not net flow of charge in any directions. If a potential difference is maintained between the ends of the metal wire (by connecting it across a battery), an electric field is set up which accelerates the free electrons: These accelerated electrons frequently collide with the atoms of the conductor, as a result, they acquire a constant speed called the drift speed which is given by Ve= 1/enA where I = current in the conductor due to drifting electrons, e = charge of electron, n = number of free electrons per unit volume of the conductor and A = area of cross-section of the conductor. A current of 1 A flows through a copper wire. The number of electrons passing through any cross-section of the wire in 1.6 sec is (charge of a electron = 1.6 xx 10^(-19 c).
View Solution