A capacitor of capacitance C1=1μF withstand a maximum voltage of V1=6KV, and another capacitor of capacitance C2=2μF, can with stand a maximum voltage of V2=4KV. If they are connected in series, what maximum voltage will the system withstand?
The correct Answer is:9KV
To solve the problem step by step, we will follow these steps:
Step 1: Identify the given values
We have two capacitors:
- Capacitor 1:
- Capacitance C1=1μF=1×10−6F
- Maximum Voltage V1=6kV=6000V
- Capacitor 2:
- Capacitance C2=2μF=2×10−6F
- Maximum Voltage V2=4kV=4000V
Step 2: Calculate the maximum charge for each capacitor
The charge Q on a capacitor is given by the formula:
Q=C×V
For Capacitor 1:
Q1=C1×V1=(1×10−6F)×(6000V)=6×10−3C
For Capacitor 2:
Q2=C2×V2=(2×10−6F)×(4000V)=8×10−3C
Step 3: Determine the maximum charge the series combination can withstand
When capacitors are connected in series, the charge Q on each capacitor is the same. Therefore, the maximum charge that the series combination can withstand is determined by the capacitor with the lower maximum charge.
From Step 2:
- Q1=6×10−3C
- Q2=8×10−3C
The maximum charge Qmax for the series combination is:
Qmax=min(Q1,Q2)=6×10−3C
Step 4: Calculate the total voltage across the series combination
The total voltage V across the series combination of capacitors can be calculated using the formula:
V=QmaxCeq
where Ceq is the equivalent capacitance of the capacitors in series.
The equivalent capacitance Ceq for capacitors in series is given by:
1Ceq=1C1+1C2
Calculating Ceq:
1Ceq=11×10−6+12×10−6
1Ceq=1000000+500000=1500000
Ceq=11500000≈6.67×10−7F
Now substituting Qmax and Ceq into the voltage formula:
V=6×10−3C6.67×10−7F
V≈9000V=9kV
Final Answer
The maximum voltage that the system can withstand when the two capacitors are connected in series is 9 kV.
---
We have two capacitors:
- Capacitor 1:
- Capacitance C1=1μF=1×10−6F
- Maximum Voltage V1=6kV=6000V
- Capacitance C2=2μF=2×10−6F
- Maximum Voltage V2=4kV=4000V
The charge Q on a capacitor is given by the formula:
Q=C×V
Q1=C1×V1=(1×10−6F)×(6000V)=6×10−3C
Q2=C2×V2=(2×10−6F)×(4000V)=8×10−3C
When capacitors are connected in series, the charge Q on each capacitor is the same. Therefore, the maximum charge that the series combination can withstand is determined by the capacitor with the lower maximum charge.
- Q1=6×10−3C
- Q2=8×10−3C
Qmax=min(Q1,Q2)=6×10−3C
The total voltage V across the series combination of capacitors can be calculated using the formula:
V=QmaxCeq
where Ceq is the equivalent capacitance of the capacitors in series.
1Ceq=1C1+1C2
1Ceq=11×10−6+12×10−6
1Ceq=1000000+500000=1500000
Ceq=11500000≈6.67×10−7F
V=6×10−3C6.67×10−7F
V≈9000V=9kV
The maximum voltage that the system can withstand when the two capacitors are connected in series is 9 kV.
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Single Correct
49 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Multile Correct
11 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Exercise 4.2
32 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.3
15 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
A Capacitor of capacitance C1=1μF can withstand a maximum voltage V1=6.0 KV while another capacitor of capacitance C2=2.0μF withstands the maximum voltage V2=4.0 KV . What maximum will the system of these two capacitance withstand when connected in series as shown below .
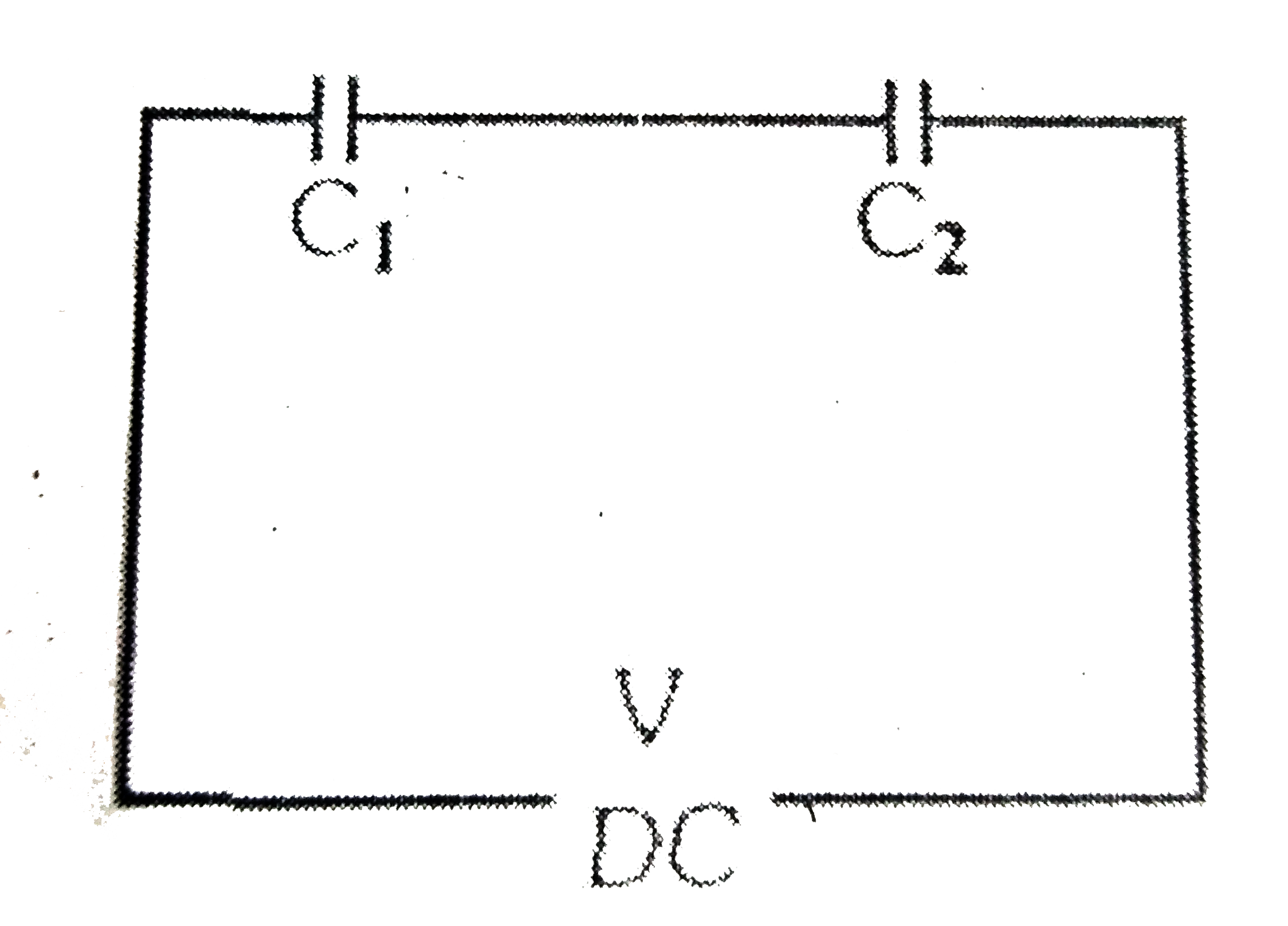
View SolutionA capaitor of capacitance C1=1.0μF withstands teh maximum voltage V1=6.0kV while a capacitor of capacitance Cs=2.0μF, the maximum voltage Vs=4.0kV. What voltage will the system of these two capacitors withsatand if they are connected in sereis ?
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionThree capacitors 2μF, 3μF and 5μF can withstand voltages to 3V,2V and 1V respectively. Their series combination can withstand a maximum voltage equal to
View SolutionTwo capacitor having capacitances 8μF and 16μF have breaking voltage 20V and 80V. They are combined in series. The maximum charge they can store individually in the combination is
View SolutionA capacitor of 0.75μF is charged to a voltage of 16 V. What is the magnitude of the charge on each plate of the capacitor ?
View SolutionThree capacitors each of capacitance C and of breakdown voltage V are joined in series. The capacitance and breakdown voltage of the combination will be
View SolutionA capacitor of capacitance C=2.0±0.1μF is charged to a voltage V=20±0.2V. What will be the charge Q on the capacitor ? Use Q=CV.
View SolutionTwo capacitors of capacitance of 6μFand12μF are connected in series with a battery. The voltage across the 6μF capacitor is 2V. Compute the total battery voltage.
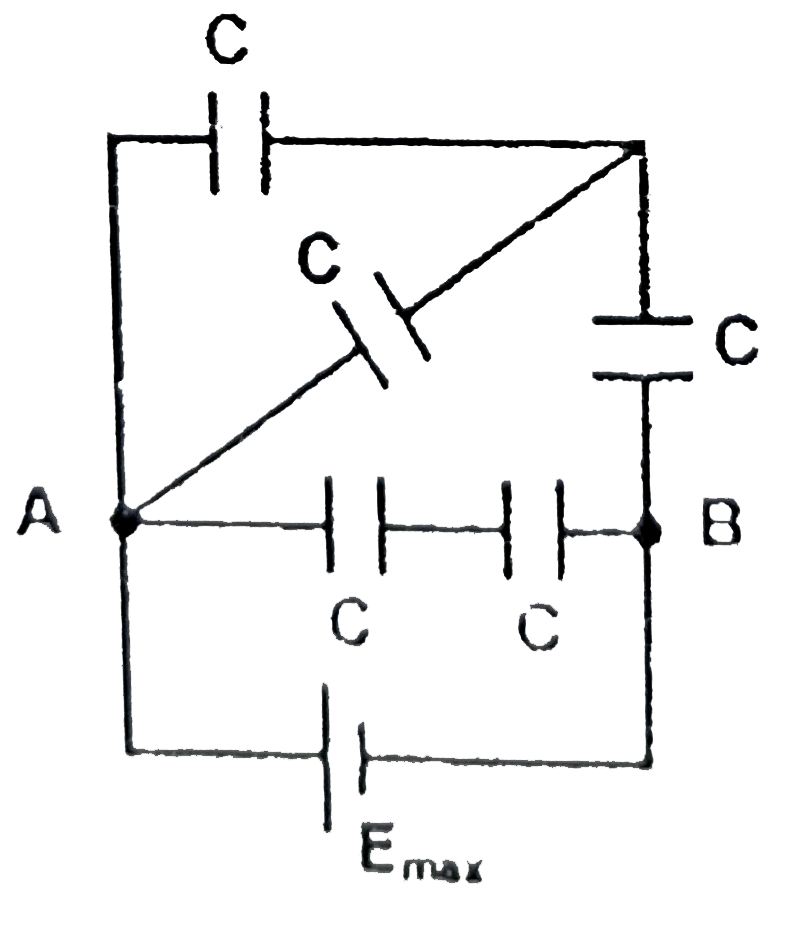
View SolutionAn RC circuit is made using five identical capacitors, each of which can withstand a maximum voltage of 2 volts. The maximum voltage which can be safely applied across points A and B is
View Solution
