In a mixed grouping of identical cells, five rows are connected in parallel and each row contains 10 cells. This combination sends a current I through an external resistance of 20Ω. if the emf and internal resistance of each cell is 1.5 V and 1Ω, respectively then find the value of I.
5/22 A
15/22 A
3/7 A
5/9 A
The correct Answer is:B
To solve the problem step by step, we will analyze the configuration of the cells and calculate the current I flowing through the external resistance.
Step 1: Understand the Configuration
We have 5 rows of cells connected in parallel, with each row containing 10 cells. Each cell has an EMF (electromotive force) of 1.5 V and an internal resistance of 1 Ω.
Step 2: Calculate the EMF and Internal Resistance for One Row
For one row of 10 cells connected in series:
- The total EMF Erow of one row is:
Erow=10×1.5V=15V
- The total internal resistance rrow of one row is:
rrow=10×1Ω=10Ω
Step 3: Calculate the Equivalent EMF and Internal Resistance for the Parallel Configuration
Since we have 5 rows connected in parallel:
- The equivalent EMF Eeq remains the same as that of one row:
Eeq=15V
- The equivalent internal resistance req for the 5 rows in parallel is calculated using the formula for resistors in parallel:
1req=1rrow+1rrow+1rrow+1rrow+1rrow=510⟹req=105=2Ω
Step 4: Set Up the Circuit with External Resistance
Now, we have:
- Equivalent EMF Eeq=15V
- Equivalent internal resistance req=2Ω
- External resistance R=20Ω
Step 5: Apply Ohm's Law to Find the Current I
Using Ohm's law, the total resistance Rtotal in the circuit is:
Rtotal=req+R=2Ω+20Ω=22Ω
Now, we can calculate the current I using the formula:
I=EeqRtotal=15V22Ω
Step 6: Final Calculation
Calculating the current:
I=1522A
Thus, the value of the current I is:
I=1522A
---
We have 5 rows of cells connected in parallel, with each row containing 10 cells. Each cell has an EMF (electromotive force) of 1.5 V and an internal resistance of 1 Ω.
For one row of 10 cells connected in series:
- The total EMF Erow of one row is:
Erow=10×1.5V=15V
- The total internal resistance rrow of one row is:
rrow=10×1Ω=10Ω
Since we have 5 rows connected in parallel:
- The equivalent EMF Eeq remains the same as that of one row:
Eeq=15V
- The equivalent internal resistance req for the 5 rows in parallel is calculated using the formula for resistors in parallel:
1req=1rrow+1rrow+1rrow+1rrow+1rrow=510⟹req=105=2Ω
Now, we have:
- Equivalent EMF Eeq=15V
- Equivalent internal resistance req=2Ω
- External resistance R=20Ω
Using Ohm's law, the total resistance Rtotal in the circuit is:
Rtotal=req+R=2Ω+20Ω=22Ω
Now, we can calculate the current I using the formula:
I=EeqRtotal=15V22Ω
Calculating the current:
I=1522A
I=1522A
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Solved Examples12videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Exercise 5.128videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits15videos
ELECTRIC CURRENT & CIRCUITS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
Similar Questions
Find the minimum number of cell required to produce an electron current of 1.5A through a resistance of 30Ω .Given that the emf internal resistance of each cell are 1.5V and 1.0Ω respectively
View SolutionThe n rows each containinig m cells in series are joined parallel. Maximum current is taken from this combination across jan external resistance of 3Ω resistance. If the total number of cells used are 24 and internal resistance of each cell is 0.5Ω then
View SolutionThe internal resistance of a 2.1V cell which gives a current 0.2A through a resistance of 10Ω
View SolutionWhen two identical cells are connected either in series or in parallel across a 4 ohm resistor, they send the same current through it. The internal resistance of the cell in ohm is
View SolutionA cell supplies a current of 2 A when it is connected to a 5Ω resistance and supplies a current of 1.2 A, if it is connected to a resistance of 9 Ω. Find the e.m.f and internal resistance of the cell.
View SolutionN identical cells whether joined together in series or in parallel, give the same current, when connected to an external resistance of 'R'. The internal resistance of each cell is:-
View SolutionThe internal resistance of a 2.1 V cell which gives a current of 0.2A through a resistance of 10Ω is
View SolutionA cell has an emf 1.5V. When connected across an external resistance of 2Ω, the terminal potential difference falls to 1.0V. The internal resistance of the cell is:
View SolutionIn the circuit shown in fig. the cell has emf 10V and internal resistance 1Ω
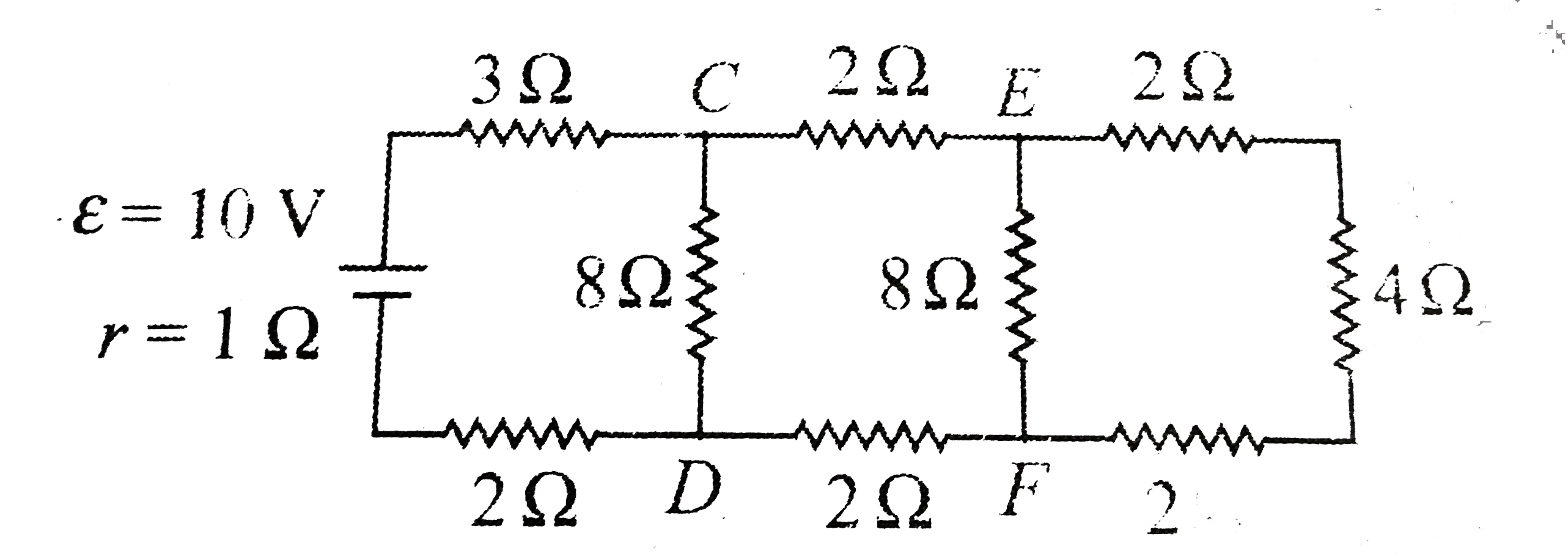 View Solution
View SolutionA cell which has an emf 1.5 V is connectedin series with an external resistance of 10Ω. If the potential difference across the cell is 1.25 V, then the internal resistance of the cell is (inΩ)
View Solution

