Question

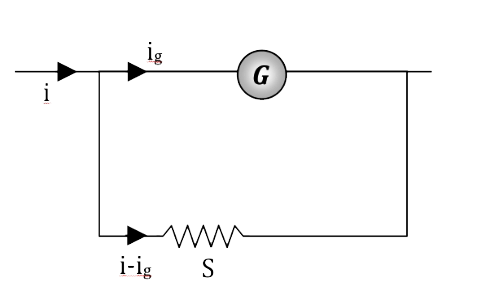
What is the value of the shunt that passes $10\%$ of the main current through a galvenomenter of $99\Omega$?

Explanation

To find the value of the shunt resistance that allows 10% of the main current to pass through a galvanometer with a resistance of 99 ohms, we can follow these steps:

1. Understand the Current Distribution:
- Let the total main current be \( I \).
- According to the problem, 10% of this current passes through the galvanometer. Therefore, the current through the galvanometer \( I_G = 0.1 I \).
- The remaining current, which passes through the shunt resistance \( S \), is \( I_S = 0.9 I \).

2. Use Ohm's Law:
- The galvanometer and the shunt are connected in parallel, which means the potential difference across both is the same.
- According to Ohm's Law, the voltage across the galvanometer can be expressed as:
\( V_G = I_G \times R_G = (0.1 I) \times 99 \)
- The voltage across the shunt can be expressed as:
\( V_S = I_S \times S = (0.9 I) \times S \)

3. Set the Voltages Equal:
- Since the voltages across the galvanometer and the shunt are equal, we can set them equal to each other:
\( (0.1 I) \times 99 = (0.9 I) \times S \)

4. Cancel \( I \):
- Since \( I \) is common in both sides and is not zero, we can cancel it out:
\( 0.1 \times 99 = 0.9 \times S \)

5. Solve for \( S \):
- Rearranging the equation gives:
\( S = \frac{0.1 \times 99}{0.9} \)
- Calculating this gives:
\( S = \frac{9.9}{0.9} = 11 \text{ ohms} \)

6. Final Answer:
- Therefore, the value of the shunt resistance \( S \) is \( 11 \) ohms.