Question

To send 10% of the main current through a moving coil galvanometer of resistance $99\Omega$, the shunt required is –

Options

A. `9Omega`
B. `11Omega`
C. `10Omega`
D. `9.9Omega`

Answer

B

Explanation

To find the shunt resistance required to send 10% of the main current through a moving coil galvanometer with a resistance of 99 ohms, we can follow these steps:

Step 1: Understand the Circuit Configuration
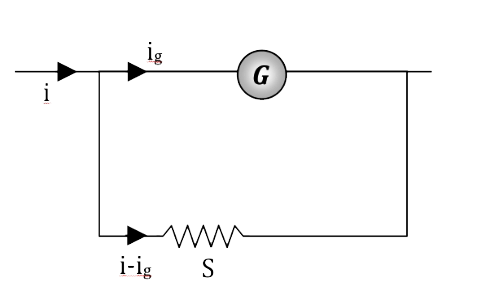
In a galvanometer circuit, we have two paths for the current: one path goes through the galvanometer (G) and the other path goes through the shunt resistor (Rs). We want to ensure that only 10% of the total current (I) flows through the galvanometer.

Step 2: Define the Currents
Let:
- I = Total current flowing in the circuit
- Ig = Current through the galvanometer
- Is = Current through the shunt resistor

Since we want 10% of the total current to flow through the galvanometer:
\( Ig = \frac{I}{10} \)

The remaining current will flow through the shunt:
\( Is = I - Ig = I - \frac{I}{10} = \frac{9I}{10} \)

Step 3: Apply Ohm's Law
According to Ohm's law, the potential difference (V) across the galvanometer and the shunt resistor must be the same.

The voltage across the galvanometer (Vg) is given by:
\( Vg = Ig \cdot Rg \)
Where \( Rg = 99 \, \Omega \) (the resistance of the galvanometer).

Substituting for Ig:
\( Vg = \left(\frac{I}{10}\right) \cdot 99 \)

The voltage across the shunt resistor (Vs) is given by:
\( Vs = Is \cdot Rs \)

Since \( Vg = Vs \):
\( \left(\frac{I}{10}\right) \cdot 99 = \left(\frac{9I}{10}\right) \cdot Rs \)

Step 4: Simplify the Equation
We can cancel \( I \) from both sides (assuming \( I \neq 0 \)):
\( \frac{99}{10} = \frac{9}{10} \cdot Rs \)

Step 5: Solve for Rs
To isolate Rs, multiply both sides by \( \frac{10}{9} \):
\( Rs = \frac{99}{9} \)

Calculating this gives:
\( Rs = 11 \, \Omega \)

Conclusion
The shunt resistance required is \( 11 \, \Omega \).

---