Question

A galvanometer of resistance 150 ohm is shunted such that only 1/11 of the main current flows through the galvanometer. The resistance of the shunt is, (in ohm)

Options

A. 5
B. 10
C. 15
D. 25

Answer

C

Explanation

To find the resistance of the shunt in the given problem, we can follow these steps:

1. Understand the Problem:
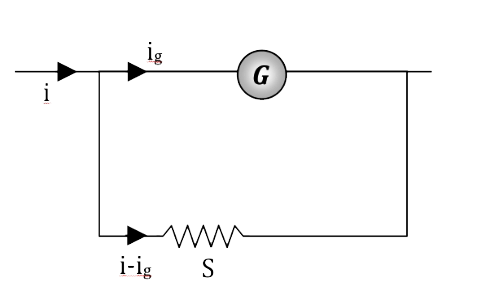
We have a galvanometer with a resistance of \( R_g = 150 \, \Omega \) and it is shunted such that only \( \frac{1}{11} \) of the main current \( I \) flows through the galvanometer.

2. Define Variables:
Let \( I_g \) be the current through the galvanometer. According to the problem, \( I_g = \frac{I}{11} \).

3. Use the Concept of Parallel Resistance:
The galvanometer and the shunt resistor \( R_s \) are in parallel. The equivalent resistance \( R_{eq} \) of the parallel combination can be given by:
\( \frac{1}{R_{eq}} = \frac{1}{R_g} + \frac{1}{R_s} \)

4. Relate the Currents:
The total current \( I \) splits into two parts: \( I_g \) through the galvanometer and \( I_s \) through the shunt. We know:
\( I_s = I - I_g = I - \frac{I}{11} = \frac{10I}{11} \)

5. Apply Ohm's Law:
The voltage across both resistors is the same. Therefore, we can write:
\( I_g R_g = I_s R_s \)
Substituting the values we have:
\( \left(\frac{I}{11}\right) \cdot 150 = \left(\frac{10I}{11}\right) \cdot R_s \)

6. Cancel \( I \):
Since \( I \) is common in both sides, we can cancel it out (assuming \( I \neq 0 \)):
\( \frac{150}{11} = \frac{10}{11} R_s \)

7. Solve for \( R_s \):
Multiply both sides by \( 11 \) to eliminate the fraction:
\( 150 = 10 R_s \)
Now, divide by \( 10 \):
\( R_s = 15 \, \Omega \)

Final Answer:
The resistance of the shunt \( R_s \) is \( 15 \, \Omega \).