A homogeneous ball (mass=m) of ideal black material at rest is illuminated with a radiation having a set of photons (wavelength=λ), each with the same momentum and the same energy. The rate at whoch photons fall on the ball is n. the linear acceleration of the ball is
mλnh
nhmλ
nh(2π)(mλ)
2λnh
The correct Answer is:B
To find the linear acceleration of the ball when illuminated by photons, we can follow these steps:
Step 1: Understand the Problem
We have a homogeneous ball of mass m that is illuminated by photons with a wavelength λ. The rate at which photons strike the ball is n. Each photon has a certain momentum and energy.
Step 2: Determine the Momentum of a Single Photon
The momentum p of a single photon can be expressed using the formula:
p=hλ
where h is Planck's constant.
Step 3: Calculate the Total Momentum Imparted to the Ball
If n photons are falling on the ball per second, the total momentum imparted to the ball per unit time (which is the force F) can be calculated as:
F=n⋅p=n⋅hλ
Step 4: Apply Newton's Second Law
According to Newton's second law, the force acting on an object is equal to the mass of the object multiplied by its acceleration a:
F=m⋅a
Substituting the expression for force from Step 3, we have:
n⋅hλ=m⋅a
Step 5: Solve for Linear Acceleration a
Rearranging the equation to solve for acceleration a:
a=n⋅hλm
This simplifies to:
a=n⋅hm⋅λ
Final Answer
Thus, the linear acceleration of the ball is:
a=n⋅hm⋅λ
We have a homogeneous ball of mass m that is illuminated by photons with a wavelength λ. The rate at which photons strike the ball is n. Each photon has a certain momentum and energy.
The momentum p of a single photon can be expressed using the formula:
p=hλ
where h is Planck's constant.
If n photons are falling on the ball per second, the total momentum imparted to the ball per unit time (which is the force F) can be calculated as:
F=n⋅p=n⋅hλ
According to Newton's second law, the force acting on an object is equal to the mass of the object multiplied by its acceleration a:
F=m⋅a
Substituting the expression for force from Step 3, we have:
n⋅hλ=m⋅a
Rearranging the equation to solve for acceleration a:
a=n⋅hλm
This simplifies to:
a=n⋅hm⋅λ
Thus, the linear acceleration of the ball is:
a=n⋅hm⋅λ
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Multiple Correct
10 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Linked Comprehension
44 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Subjective
16 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
Similar Questions
Knowledge Check
n' photons of wavelength 'λ' are absorbed by a black body of mass 'm'. The momentum gained by the body is
AhmλBmnhλCnhλDnhλIf m is the mass of an electron and c the speed of light, the ratio of the wavelength of a photon of energy E to that of the electron of the same energy is
Ac√2mEB√2mEC√2mcED√mE
A ball of mass m moves with speed v and stricks a wall having infinite mass and it returns with same speed then the work done by the ball on the wall is
View SolutionTwo balls of same material and same surface finish have their diameters in the ratio 1:2. They are heated to the same temperature and are left in a room to cool by radiation, then the initial rate of loss of heat
View SolutionA ball of mass 4 kg moving on a smooth horizontal surface makes an elastic collision with another ball of mass m at rest in the line of motion of first ball. If after collision first ball moves in the same direction with one fourth of its velocity before collision, then mass of second ball is
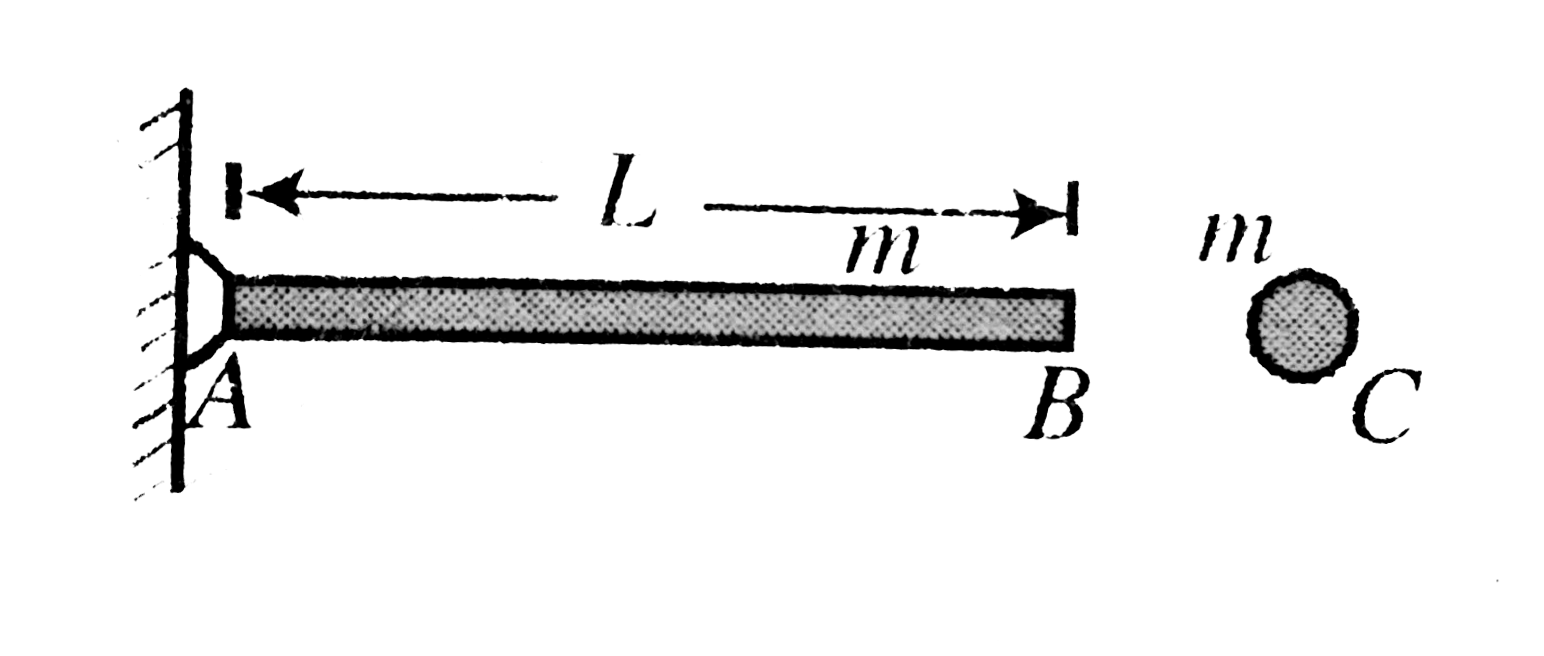
View SolutionA uniform bar AB of mass m and a ball of the same mass are released from rest from the same horizontal position. The bar is hinged at end A. There is gravity downwards. What is the distance of the point from point B that has the same acceleration as the ball, immediately after release?
View SolutionA metallic ball and highly stretched spring are made of the same material and have the same mass. They are heated so that they melt. The latent heat required
View SolutionA spherical ball of mass 1kg moving with a uniform speed of 1m/s collides symmetrically with two identical spherical balls of mass 1kg each at rest touching each other. If the two balls move with 0.5m/s in two directions at the same angle of 60∘ with the direction of the first ball, the loss of kinetic energy on account of the collision is :-
View Solution
