An atom emits a spectral line of wavelength when an electron makes a transition between levels of energy . Which expression correctly relates ?
The correct Answer is:D
To solve the problem, we need to relate the wavelength of the emitted spectral line (λ) to the energy levels (E₁ and E₂) of the atom.
1. Understand the Energy Transition: When an electron transitions between two energy levels in an atom, it emits or absorbs energy in the form of a photon. The energy of the photon corresponds to the difference in energy between the two levels.
2. Write the Energy Difference: The energy difference (ΔE) between the two levels can be expressed as:
ΔE=E2−E1
(Note: If E₂ is higher than E₁, the transition is from E₂ to E₁, and the energy difference will be E₂ - E₁.)
3. Relate Energy to Wavelength: The energy of the emitted photon is also related to its wavelength (λ) by the equation:
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s).
4. Set the Two Expressions Equal: Since the energy of the photon emitted during the transition is equal to the energy difference between the two levels, we can set the equations equal to each other:
E2−E1=hcλ
5. Rearrange for Wavelength: To find the expression for λ, rearrange the equation:
λ=hcE2−E1
6. Final Expression: The final expression that relates λ, E₁, and E₂ is:
λ=hcE2−E1
Conclusion:
The correct expression relating λ, E₁, and E₂ is:
λ=hcE2−E1
ΔE=E2−E1
(Note: If E₂ is higher than E₁, the transition is from E₂ to E₁, and the energy difference will be E₂ - E₁.)
E=hcλ
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s).
E2−E1=hcλ
λ=hcE2−E1
λ=hcE2−E1
The correct expression relating λ, E₁, and E₂ is:
λ=hcE2−E1
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Multiple Correct
13 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Linked Comprehension
62 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Subject
17 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
Similar Questions
The wavelength λe of an electron and λp of a photon of same energy E are related by
View SolutionTransition between three energy energy levels in a particular atom give rise to three Spectral line of wevelength , in increasing magnitudes. λ1,λ2 and λ3. Which one of the following equations correctly ralates λ1,λ2 and λ3?
View SolutionTransition between three energy energy levels in a particular atom give rise to three Spectral line of wevelength , in increasing magnitudes. λ1,λ2 and λ3. Which one of the following equations correctly ralates λ1,λ2 and λ3?
λ1=λ2−λ3
λ1=λ3−λ2
1λ1=1λ2+1λ3
1λ2=1λ3+1λ1
View SolutionThe wavelength of the spectral line when the electron is the hydrogen atom undergoes a transition from the energy level 4 to energy level 2 is.
View SolutionThe wavelengths of photons emitted by electron transition between two similar leveis in H and He+ are λ1 and λ2 respectively. Then :-
View SolutionThe wavelengths of photons emitted by electron transition between two similar leveis in H and He+ are λ1 and λ2 respectively. Then :-
View SolutionA dye absorbs a photon of wavelength λ and re− emits the same energy into two phorons of wavelengths λ1 and λ2 respectively. The wavelength λ is related with λ1 and λ2 as :
View SolutionCalculate the wavelength of radiation emited when an electron in a hydrogen atom makes a transition from an energy level with n=3 to a level with n=2
View SolutionThe following diagram indicates the energy levels of a certain atom when the system moves from 4E level to E. A photon of wavelength λ1 is emitted. The wavelength of photon produced during its transition from 73E level to E is λ2. the ratio λ1λ2 will be
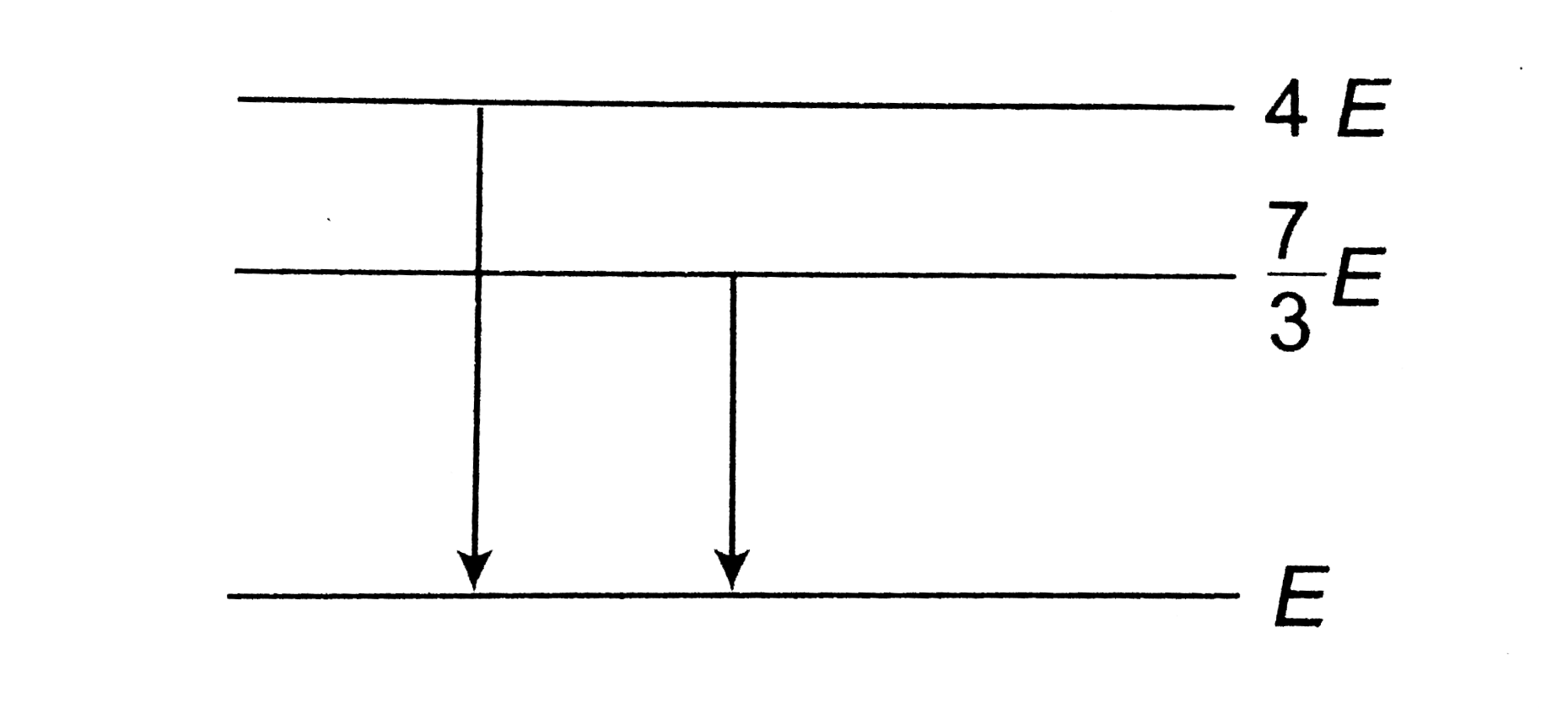
View SolutionThe following diagram indicates the energy levels of a certain atom when the system moves from 4E level to E. A photon of wavelength λ1 is emitted. The wavelength of photon produced during its transition from 73E level to E is λ2. the ratio λ1λ2 will be

View Solution
