Masses of two isobars .29Cu64 and .30Zn64 are 63.9298u and 63.9292u, respectively. It can be concluded from these data that .
both the isobars are stable
.64Zn is radioactive, decaying to .64Cu through β− decay
.64Cu is radioactive, decaying to .64Zn through β-decay
.64Cu is radioactive, decaying to .64Zn through γ-decay
The correct Answer is:c
To solve the problem regarding the isobars Copper-64 and Zinc-64, we will analyze the information given about their masses and the nature of their decay.
1. Identify the Isobars:
- We have two isobars: Copper-64 (6429Cu) and Zinc-64 (6430Zn). Isobars are nuclides that have the same mass number but different atomic numbers.
2. Analyze the Masses:
- The mass of Copper-64 is given as 63.9298u and the mass of Zinc-64 is 63.9292u.
- Since the mass of Copper-64 is greater than that of Zinc-64, this indicates that Copper-64 is less stable and is likely to undergo decay.
3. Determine the Type of Decay:
- Since Copper-64 is decaying into Zinc-64, we can conclude that this is a beta decay process. In beta decay, a neutron in the nucleus is converted into a proton and an electron (beta particle) is emitted.
- The atomic number increases by 1 (from 29 for Copper to 30 for Zinc), which is characteristic of beta negative decay.
4. Write the Decay Equation:
- The decay can be represented as follows:
6429Cu→6430Zn+β−+¯ν
- Here, β− represents the emitted beta particle (electron), and ¯ν represents the antineutrino.
5. Conclude the Nature of the Isobars:
- From the above analysis, we conclude that Copper-64 is radioactive and decays into Zinc-64 through beta decay.
- Since Zinc-64 is the product of this decay, it is stable and does not undergo further decay.
Conclusion:
From the data provided, we can conclude that Copper-64 is radioactive and decays into Zinc-64 through beta decay.
- We have two isobars: Copper-64 (6429Cu) and Zinc-64 (6430Zn). Isobars are nuclides that have the same mass number but different atomic numbers.
- The mass of Copper-64 is given as 63.9298u and the mass of Zinc-64 is 63.9292u.
- Since the mass of Copper-64 is greater than that of Zinc-64, this indicates that Copper-64 is less stable and is likely to undergo decay.
- Since Copper-64 is decaying into Zinc-64, we can conclude that this is a beta decay process. In beta decay, a neutron in the nucleus is converted into a proton and an electron (beta particle) is emitted.
- The atomic number increases by 1 (from 29 for Copper to 30 for Zinc), which is characteristic of beta negative decay.
- The decay can be represented as follows:
6429Cu→6430Zn+β−+¯ν
- Here, β− represents the emitted beta particle (electron), and ¯ν represents the antineutrino.
- From the above analysis, we conclude that Copper-64 is radioactive and decays into Zinc-64 through beta decay.
- Since Zinc-64 is the product of this decay, it is stable and does not undergo further decay.
From the data provided, we can conclude that Copper-64 is radioactive and decays into Zinc-64 through beta decay.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Linked Comprehension29videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Integer6videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Subjective35videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer12videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Integer Type4videos
PHOTOELECTRIC EFFECT
Similar Questions
An object is placed in front of two convex lenses one by one at a distance u from the lens. The focal lengths of the lenses are 30 cm and 15 cm respectively. If the size of image formed in the two cases is same, then u is
View SolutionAs part of his discovery of the neutron in 1932, James Chadwick determined the mass of the neutron (newly identified particle) by firing a beam of fast meutrons, all having the same speed, as two different targets and measuing the maximum recoil speeds of the target nuclei. The maximum speed arise when an elastic head-on collision occurs between a neutron and a stationary target nucleus. Represent the masses and final speeds of the two target nuclei as m1,v1,m2 and v2 and assume Newtonian mechanics applies. The neutron mass can be calculated from the equation:
mn=m1v1−m2v2v2−v1
Chadwick directed a beam of neutrons on paraffin, which contains hydrogen. The maximum speed of the protons ejected was found to be 3.3×107m/s. A second experiment was performed using neutrons from the same source and nitrogen nuclei as the target. The maximum recoil speed of the nitrogen nuclei was found to be 4.7×106m/s. The masses of a proton and a nitrogen nucleus were taken as 1u and 14u, respectively. What was Chadwick's value for the neutron mass?View SolutionA helium atom, a hydrogen atom and a neutron have mases of4.003u,1.008u and 1.009u (unified atomic mass units), respectively. Assuming that hydogen atoms and neutrons can be fuse to from helium, what is the binding energy of a helium nucleus?
View Solution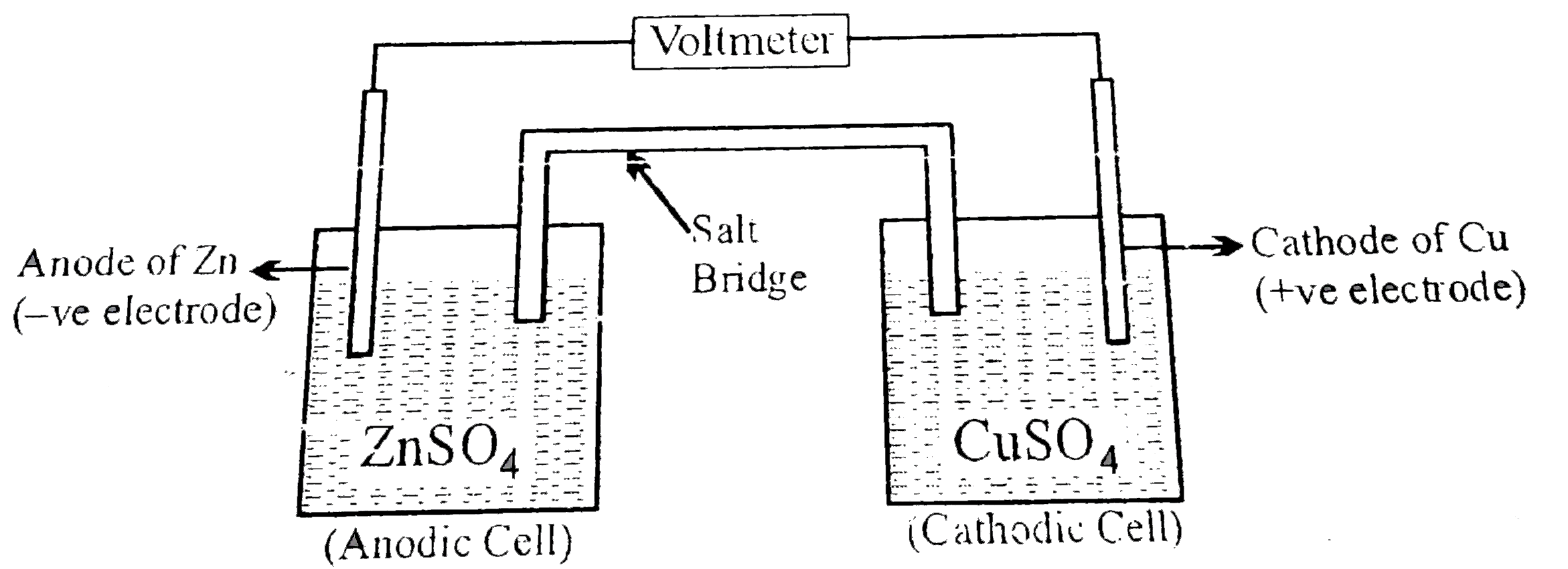
The most pupular electrochemical cell, daniel cell was originally developed by the English chemist john F daniel the general assembly of the cell is as given below An undergraduate student made a Daniel cell using 100cm3 of 0.100MCuSO4 ad 0.100M ZnSO4 solution respectively. The two compartments are connected by suitable salt bridge.
[given E∘(Cu2+/Cu)=0.34V,E(Zn2+/Zn)=−0.76V,2.30RTF=0.06,log2=0.3]
Q. A labmate of the student asked her for some solid CuCl2. while she was lifting the bottle from a shelf, the lid of the bottle slipped and some amount of CuCl2 fell in the CuSO4 compartment at constant volume. She measured the emf of the cell again and found that it has increase by 9 mV. She used this data to calculate the amount of CuCl2 that had spilled in the compartment. Calculate the mass of CuCl2 that had spilled into the daniel cell? (molar mass of CuCl2=135gmol)View SolutionTwo inclined planes OA and OB having inclination (with horizontal) 30∘and60∘, respectively, intersect each other at O as shown in figure. A particle is projected from point P with velocity u=10√3ms−1 along a direction perpendicular to plane OA. If the particle strikes plane OB perpendicularly at Q, calculate
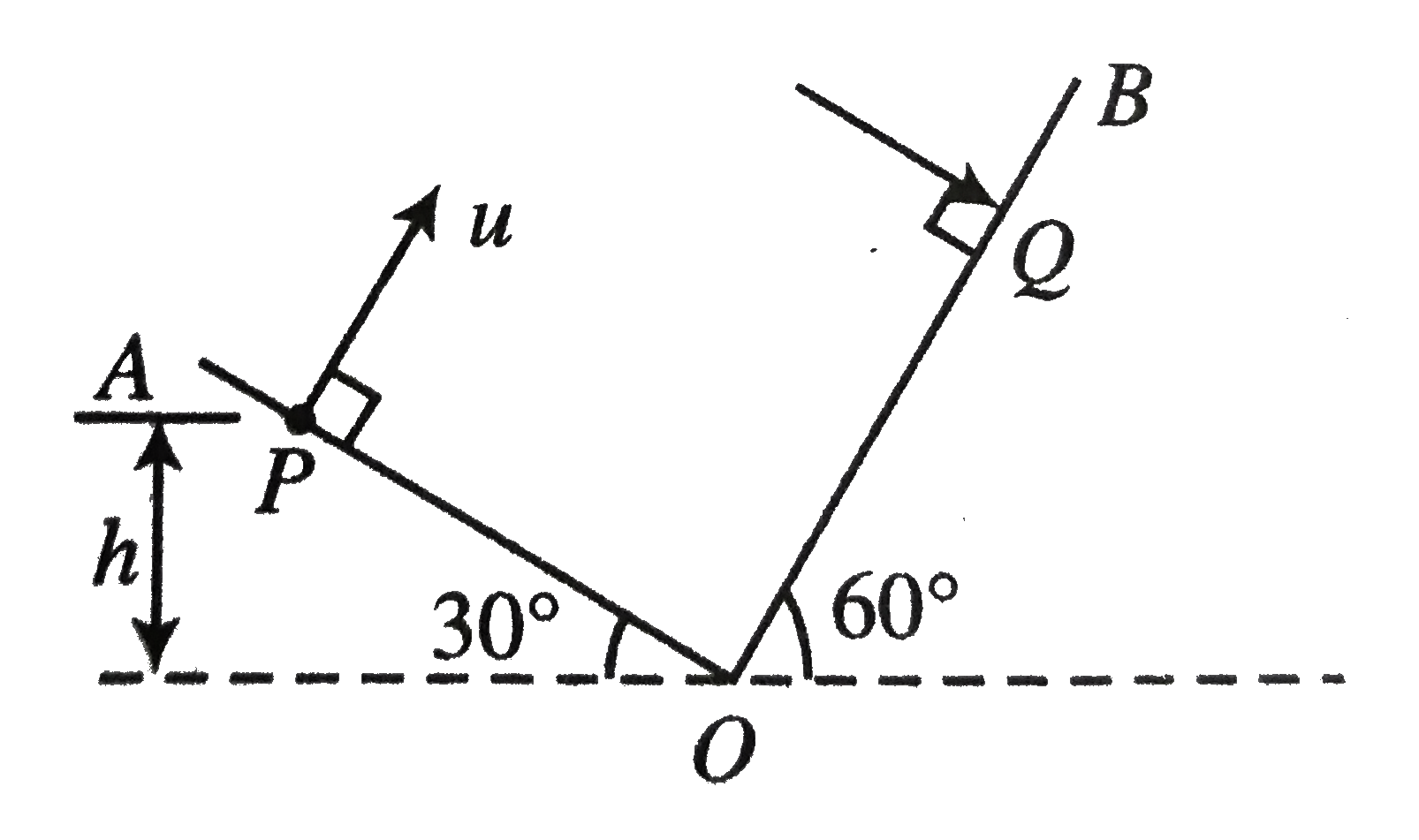
The vertical height h of P from O,View SolutionTwo inclined planes OA and OB having inclination (with horizontal) 30∘ and 60∘, respectively, intersect each other at O as shown in figure. A particle is projected from point P with velocity u=10(√3)ms−1 along a direction perpendicular to plane OA. If the particle strikes plane OB perpendicularly at Q, calculate
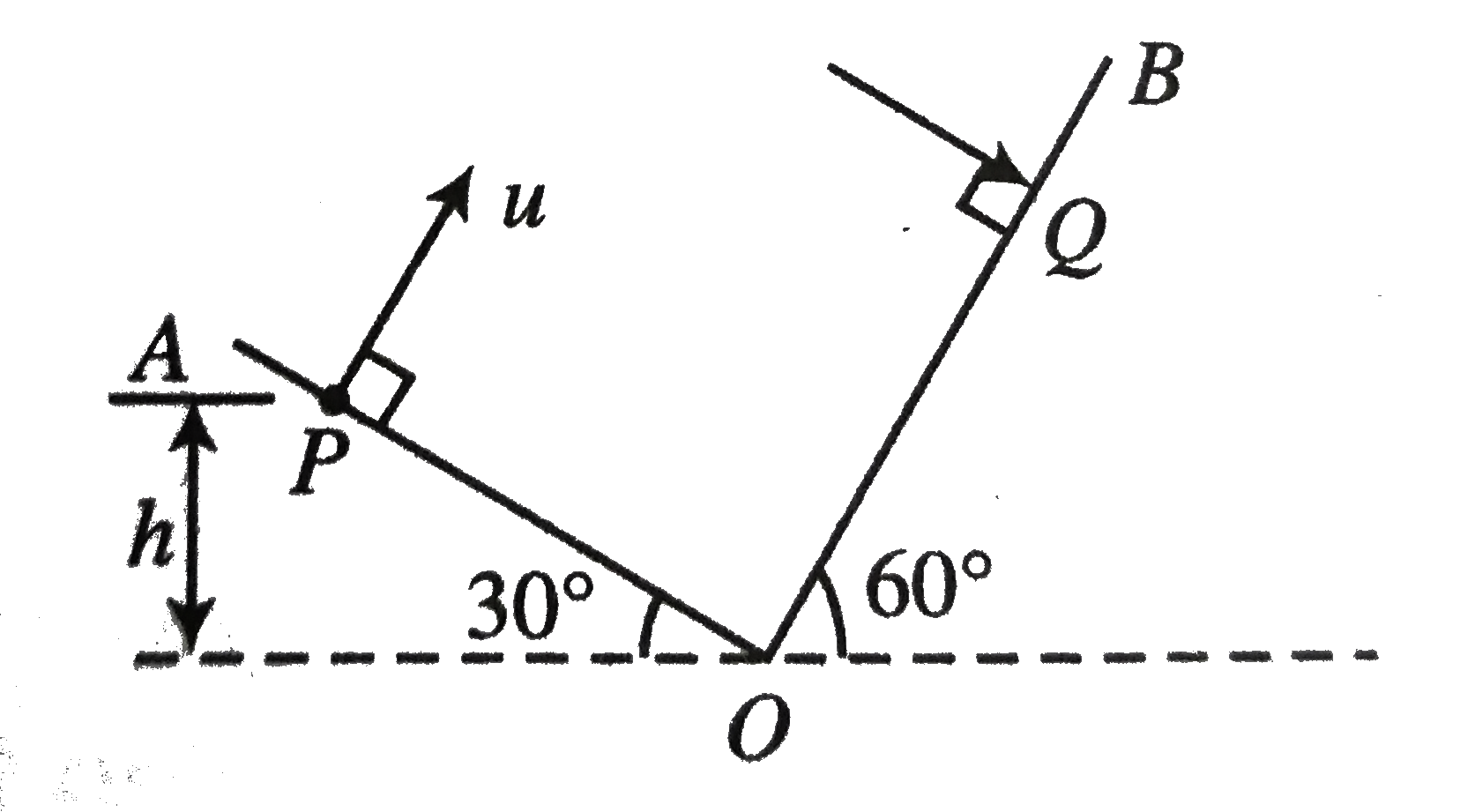
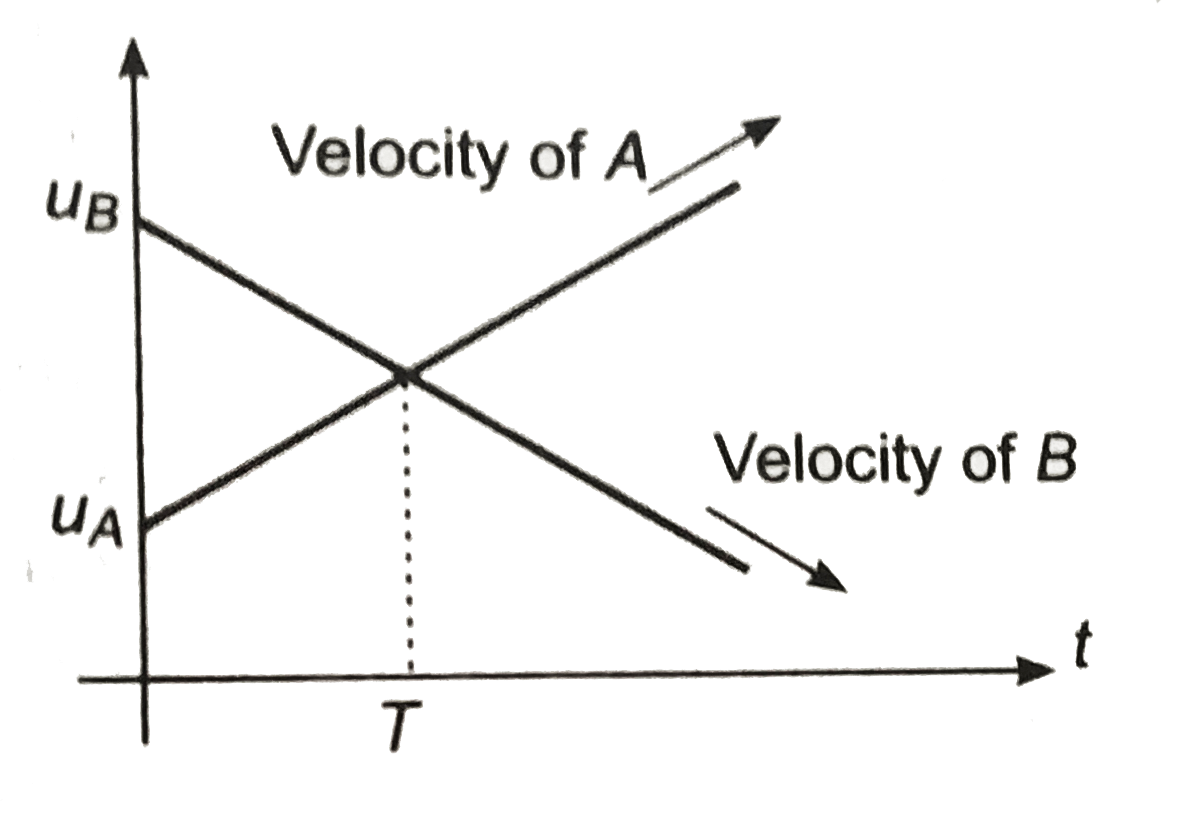
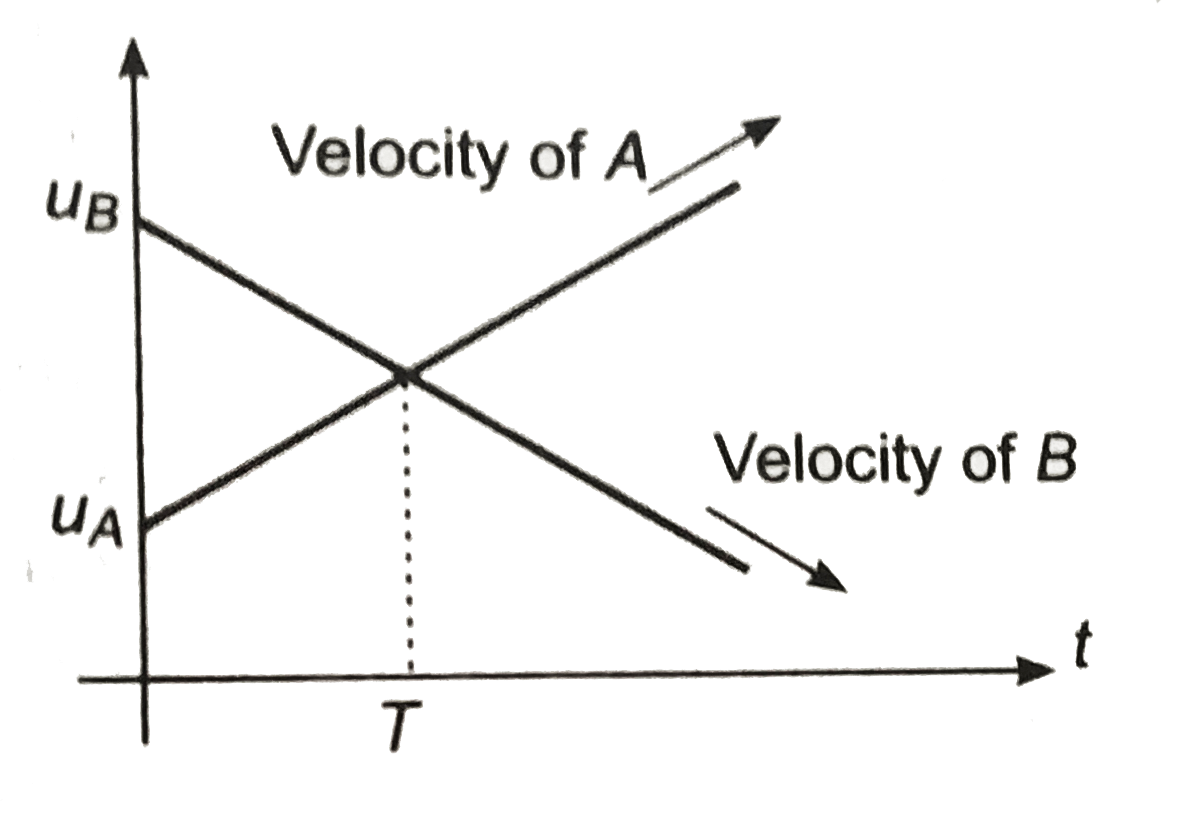
The velocity with which particle strikes the plane OB,View SolutionA situation is shown in which two objects A and B start their motion from same point in same direction. The graph of their velocities against time is drawn. uAanduB are the initial velocities of A and B respectively. T is the time at which their velocities become equal after start of motion. You cannot use the data of one question while solving another question of the same set. So all the questions are independent of each other.
6. Let vAandvB be the velocities of the particles A and B respectively at the moment A and B meet after start of the motion. If uA=5ms−1anduB=15ms−1, then the magnitude of the difference of velocities vAandvB is
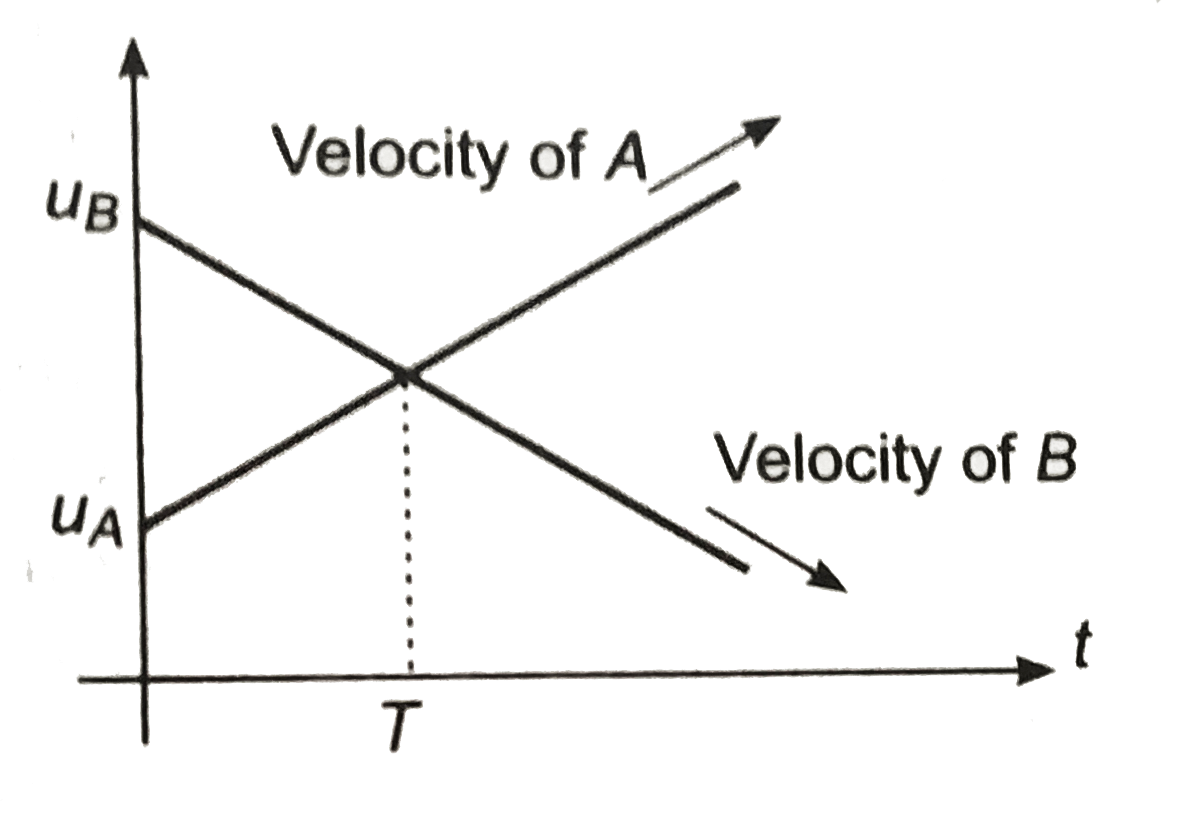 View Solution
View SolutionA situation is shown in which two objects A and B start their motion from same point in same direction. The graph of their velocities against time is drawn. uAanduB are the initial velocities of A and B respectively. T is the time at which their velocities become equal after start of motion. You cannot use the data of one question while solving another question of the same set. So all the questions are independent of each other.
7. After 10 s of the start of motion of both objects A and B, find the value of velocity of A if uA=6ms−1,uB=12ms−1 and at T velocity of A is 8ms−1andT=4s
 View Solution
View SolutionA situation is shown in which two objects A and B start their motion from same point in same direction. The graph of their velocities against time is drawn. uAanduB are the initial velocities of A and B respectively. T is the time at which their velocities become equal after start of motion. You cannot use the data of one question while solving another question of the same set. So all the questions are independent of each other.
5. If the value of T is 4 s, then the time after which A will meet B is
 View Solution
View SolutionOxygen is of vital importance for all of us . Oxygen enters the body via the lungs and is transported to the tissues in our body by blood . There it can deliver energy by the oxidation of sugars.
C6H12O6+6O2→6CO2+6H2O
This reaction releases 400 KJ of energy per mole of oxygen O2 uptake by blood is at four heme (Hm) group in this protein hemoglobin (Hb).
Free Hm consists of an Fe2+ giving HmO2 complex. Carbon monoxides can be complexed similarily giving a Hm CO complex . CO is poison as it bonds more strongly to Hm than O2 does. The equilibrium constant Kf for the reaction:
Hm+CO⇔HCO ........(i)
is 1000 times larger than the equilibrium constant K2 for the reaction:
Hm+CO2⇔HmO2 ........(ii)
Each Hb molecules can take up four molecules of O2 absorbs a fraction of this amount, depending on the oxygen pressure , as shown in figure1 (curve 1) . Also shown are the curve (2) and (3) for blood with two kinds of dificient Hb . These occur in patients with certain hereditary diseases.

Relevant data , O2 pressure in lungs is 15 KPa , in the muscles it is 2KPa . The maximum flow of blood through heart and lungs is 4×10−4m−3s−1 . The red cells in blood occupy 40% of the volume, inside the cells the concentration of Hb has a molar mass of 64 kg mol−1 R=8.314 Jmol−1K−1 , T=298k . Using the relation between K and the standard Gibbs energy ΔG∘ for a reaction, calculated the difference between the ΔG∘ values for the home reactions (i) and (ii).View Solution

