If mass of U235=235.12142a.m.u., mass of U236=236.1205aμ, and mass of neutron =1.008665amu, then the energy required to remove one neutron from the nucleus of U236 is nearly about.
75MeV
6.5MeV
1eV
zero
The correct Answer is:b
To find the energy required to remove one neutron from the nucleus of Uranium-236, we can follow these steps:
1. Identify the Masses:
- Mass of Uranium-236 (U-236) = 236.1205 amu
- Mass of Uranium-235 (U-235) = 235.12142 amu
- Mass of neutron = 1.008665 amu
2. Write the Reaction:
The reaction for removing one neutron from U-236 can be represented as:
U-236→U-235+n
where n is the neutron.
3. Calculate the Mass Defect (Δm):
The mass defect is calculated as:
Δm=mass of reactants−mass of products
Here, the mass of reactants is the mass of U-236, and the mass of products is the sum of the masses of U-235 and the neutron:
Δm=mass of U-236−(mass of U-235+mass of neutron)
Substituting the values:
Δm=236.1205amu−(235.12142amu+1.008665amu)
Δm=236.1205amu−236.130085amu
Δm=−0.009585amu
4. Convert Mass Defect to Energy:
The energy equivalent of the mass defect can be calculated using Einstein's equation E=Δmc2. However, in nuclear physics, we often use the conversion factor:
1amu≈931.5MeV
Therefore, the energy required (E) is:
E=Δm×931.5MeV
Substituting the value of Δm:
E=−0.009585amu×931.5MeV/amu
E≈−8.93MeV
5. Interpret the Result:
Since we are looking for the energy required to remove the neutron, we take the absolute value:
E≈8.93MeV
Final Answer:
The energy required to remove one neutron from the nucleus of Uranium-236 is approximately 8.93 MeV.
- Mass of Uranium-236 (U-236) = 236.1205 amu
- Mass of Uranium-235 (U-235) = 235.12142 amu
- Mass of neutron = 1.008665 amu
The reaction for removing one neutron from U-236 can be represented as:
U-236→U-235+n
where n is the neutron.
The mass defect is calculated as:
Δm=mass of reactants−mass of products
Here, the mass of reactants is the mass of U-236, and the mass of products is the sum of the masses of U-235 and the neutron:
Δm=mass of U-236−(mass of U-235+mass of neutron)
Substituting the values:
Δm=236.1205amu−(235.12142amu+1.008665amu)
Δm=236.1205amu−236.130085amu
Δm=−0.009585amu
The energy equivalent of the mass defect can be calculated using Einstein's equation E=Δmc2. However, in nuclear physics, we often use the conversion factor:
1amu≈931.5MeV
Therefore, the energy required (E) is:
E=Δm×931.5MeV
Substituting the value of Δm:
E=−0.009585amu×931.5MeV/amu
E≈−8.93MeV
Since we are looking for the energy required to remove the neutron, we take the absolute value:
E≈8.93MeV
The energy required to remove one neutron from the nucleus of Uranium-236 is approximately 8.93 MeV.
Topper's Solved these Questions
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Linked Comprehension
29 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Integer
6 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Subjective
35 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer
12 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Integer Type
4 Videos
Similar Questions
One a.m.u. stands for
View SolutionOne 'u' stands for the mass of
View SolutionIn the nuclear raction .1H2+.1H2→.2He3+.0n1 if the mass of the deuterium atom =2.014741amu, mass of .2He3 atom =3.016977amu, and mass of neutron =1.008987amu, then the Q value of the reaction is nearly .
View SolutionIn the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
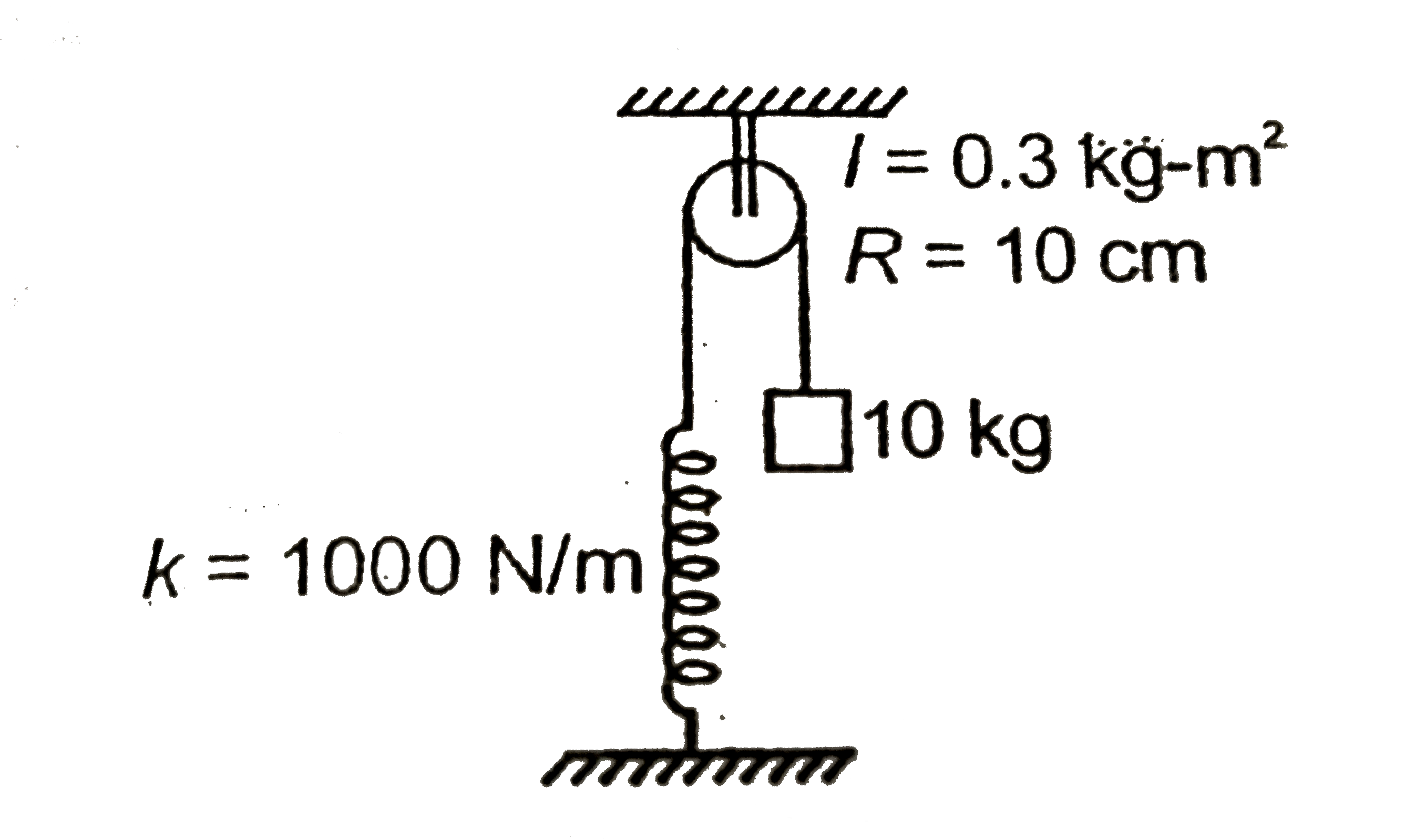
View SolutionOne a.m.u. is equal to
View SolutionWrite the approximate value of the energy released in the fission of one nucleus of 23592U. What is the reason for it ?
View SolutionThe mass of proton is 1.0073u and that of neutron is 1.0087u (u= atomic mass unit). The binding energy of .2He4 is (mass of helium nucleus =4.0015u)
View SolutionThe nuclear reaction .2H+.2H→.4He (mass of deuteron =2.0141a.m.u and mass of He=4.0024a.m.u) is
View SolutionIn fission of one uranium-235 nucleus, the loss in mass is 0.2 a.m.u. Calculate the energy released.
View SolutionConsider the case of bombardment of U235 nucleus with a thermal neutron. The fission products are Mo95 & La139 and two neutrons. Calculate the energy released by one U235 nucleus. (Rest masses of the nuclides are U235=235.0439u,.10n=1.0087u,Mo95=94.9058u,La139.9061u).
View Solution
