A long insulated copper wire is closely wound as a spiral of N turns. The spiral has inner radius a and outer radius b. The spiral lies in the xy-plane and a steady current I flows through the wire. Thez-component of the magetic field at the centre of the spiral is
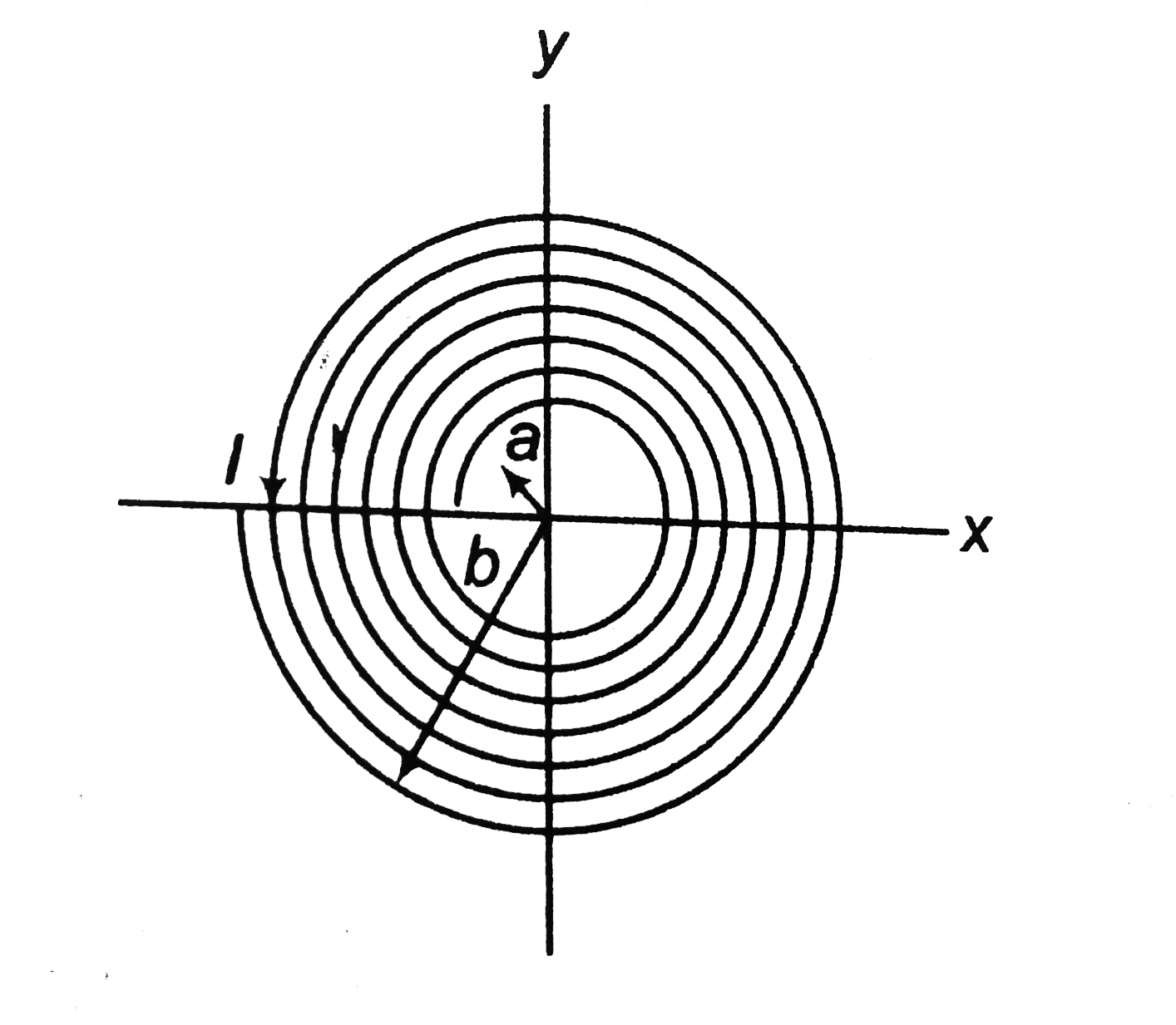
μ0NIb
2μ0NIa
μ0NI2(b−a)Inba
μ0IN2(b−a)Inba
The correct Answer is:C
(c) Let us consider a thickness dx of wire.
Let it be at a distance x form the centre
O.
Number of turns per unit length =Nb−a
Number of turns in thickness
dx=Nb−adx
Small amount of magnetic field produced at O due to thick-
ness dx of the wire
dB=μ02NI(b−a)dxx
On integrating , we get
B=∫baμ02NI(b−a)dxx=μ02NI(b−a)∫badxx
=μ02NI(b−a)[logex]ba=μ02NI(b−a)loge(ba)
Correct option is (c).

Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Archives (multiple Correct )
Explore 1 Video
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Archives (linked Comprehension)
Explore 4 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Archives (fill In The Blanks)
Explore 1 Video
- RAY OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:RAY OPTICSExercise:DPP 1.6
Explore 12 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Comprehension Type
Explore 14 Videos
Similar Questions
The magnetic field at the centre of a tightly wound spiral coil with inner radius R and outer radius 4.5 R with number of turns 22, when current i is flowing through it, is
View SolutionA coil having N turns is would tightly in the form of a spiral with inner and outer radii a and b respectively. When a current I passes through the coil, the magnetic field at the centre is.
View SolutionA circular coil 'A' has a radius R and the current flowing through it is I.Another circular coil 'B' has a radius 2R and if 2I is the current flowing through it then the magnetic fields at the centre of the circular coil are in the ratio of
View SolutionA conducting circular loop of radius a is connected to two long, straight wires . The straight wires carry a current I as shown in figure . Find the magnetic field B at the centre of the loop.

View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is

View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is :

View SolutionA long wire bent as shown in fig. carries current I. If the radius of the semicircular portion is a, the magnetic field at centre C is

View SolutionA current I flows along a thin wire shaped as shown in figure. The radius of the curved part of the wire is r. The field at the centre O of the coil is:
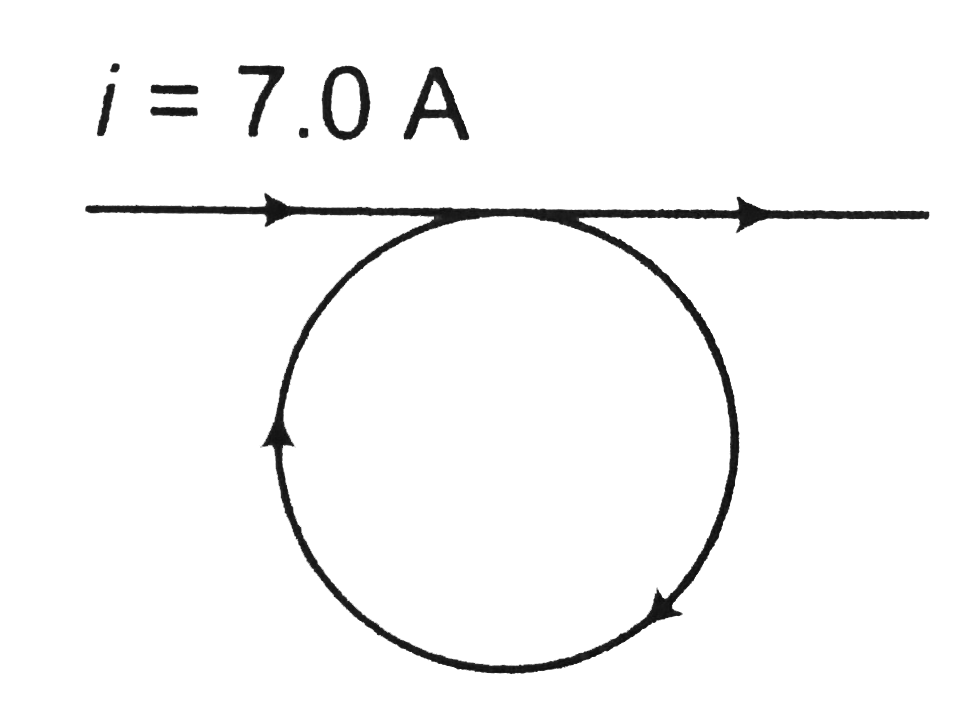
View SolutionA conductor consists of a circular loop of radius R=10cm and two straight, long sections as shown in figure. The wire lies in the plane of the paper and carries a current of i=7.00A Determine the magnitude and direction of the magnetic field at the centre of the loop.
View SolutionA thin insulated wire forms a plane spiral of N=100 turns carrying a current i=8mA. The inner and outer radii are equal to a=5cmandb=10cm. Find the magnetic induction at the centre of the spiral

View Solution