The coefficient of mutual inductance of two circuits A and B is 3mH and their respective resistanaces are 10 and 4Ω. How much current should change in 0.02s in circuit A, so that the induced current in B should be 0.0060A?
0.24A
1.6A
0.18A
0.16A
The correct Answer is:D
To solve the problem step by step, we will use the relationship between mutual inductance, induced EMF, and the change in current.
Step 1: Understand the given values
- Coefficient of mutual inductance, M=3mH=3×10−3H
- Resistance of circuit A, RA=10Ω
- Resistance of circuit B, RB=4Ω
- Induced current in circuit B, IB=0.0060A=6×10−3A
- Time interval, dt=0.02s
Step 2: Calculate the induced EMF in circuit B
The induced EMF (E) in circuit B can be calculated using Ohm's law:
E=IB×RB
Substituting the values:
E=(6×10−3A)×(4Ω)=24×10−3V=0.024V
Step 3: Relate induced EMF to the change in current in circuit A
The induced EMF can also be expressed in terms of mutual inductance and the rate of change of current in circuit A:
E=MdIdt
Where dI is the change in current in circuit A.
Step 4: Rearranging the equation to find dI
From the equation above, we can rearrange to find dI:
dI=E⋅dtM
Step 5: Substitute the known values
Now, substituting the values we have:
dI=(24×10−3V)⋅(0.02s)3×10−3H
Step 6: Calculate dI
Calculating the above expression:
dI=(24×10−3)⋅(0.02)3×10−3=0.000480.003=0.16A
Final Answer
The change in current in circuit A should be dI=0.16A.
---
- Coefficient of mutual inductance, M=3mH=3×10−3H
- Resistance of circuit A, RA=10Ω
- Resistance of circuit B, RB=4Ω
- Induced current in circuit B, IB=0.0060A=6×10−3A
- Time interval, dt=0.02s
The induced EMF (E) in circuit B can be calculated using Ohm's law:
E=IB×RB
Substituting the values:
E=(6×10−3A)×(4Ω)=24×10−3V=0.024V
The induced EMF can also be expressed in terms of mutual inductance and the rate of change of current in circuit A:
E=MdIdt
Where dI is the change in current in circuit A.
From the equation above, we can rearrange to find dI:
dI=E⋅dtM
Now, substituting the values we have:
dI=(24×10−3V)⋅(0.02s)3×10−3H
Calculating the above expression:
dI=(24×10−3)⋅(0.02)3×10−3=0.000480.003=0.16A
The change in current in circuit A should be dI=0.16A.
Topper's Solved these Questions
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Exercises (multiple Correct )
7 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Exercises (assertion-reasoning)
2 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Exercises (subjective)
7 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
Similar Questions
The coefficient of mutual induction between two circuits is equal to the, emf produced in one circuit when the current in the second circuit is
View SolutionThe coefficient of mutual inductance when magnetic flux change by 4×10−2Wb and current changes by 0.01A, will be
View SolutionThe coefficient of mutual inductance when magnetic flux change by 2×10−2Wb and current changes by 0.01A, will be
View SolutionThe coefficient of mutual inductance of the two coils is 0.5H. If the current is increased from 2 to 3 A in 0.01sec. In one of them, then the induced e.m.f. in the second coil is :
View SolutionThe coefficient of mutual inductance between the primary and secondary of the coil is 5H .A current of 10 A is cut - off in 0.5 s . The induced emf is :
View SolutionTwo circuits have coefficient of mutual induction of 0.09 henry. Average e.m.f. induced in the secondary by a change of current from 0 to 20 ampere in 0.006 second in the primary will be
View SolutionThe coefficient of mutual induction between two coils is 4H. If the current in the primary reduces from 5A to zero in 10−3 second then the induced e.m.f in the secondary coil will be
View SolutionIn a transformer , the coefficient of mutual inductance between the primary and the secondary coil is 0.2 henry. When the current changes by 5 ampere//second in the primary, the induced e.m.f. in the secondary will be
View Solutionthe resistance and inductance of series circuit are 5Ω and 20H respectively. At the instant of closing the switch, the current is increasing at the rate 4A−s. The supply voltage is
View SolutionIn the circuit shown, if a wire is connected between points A and B. How much current will flow through that wire?
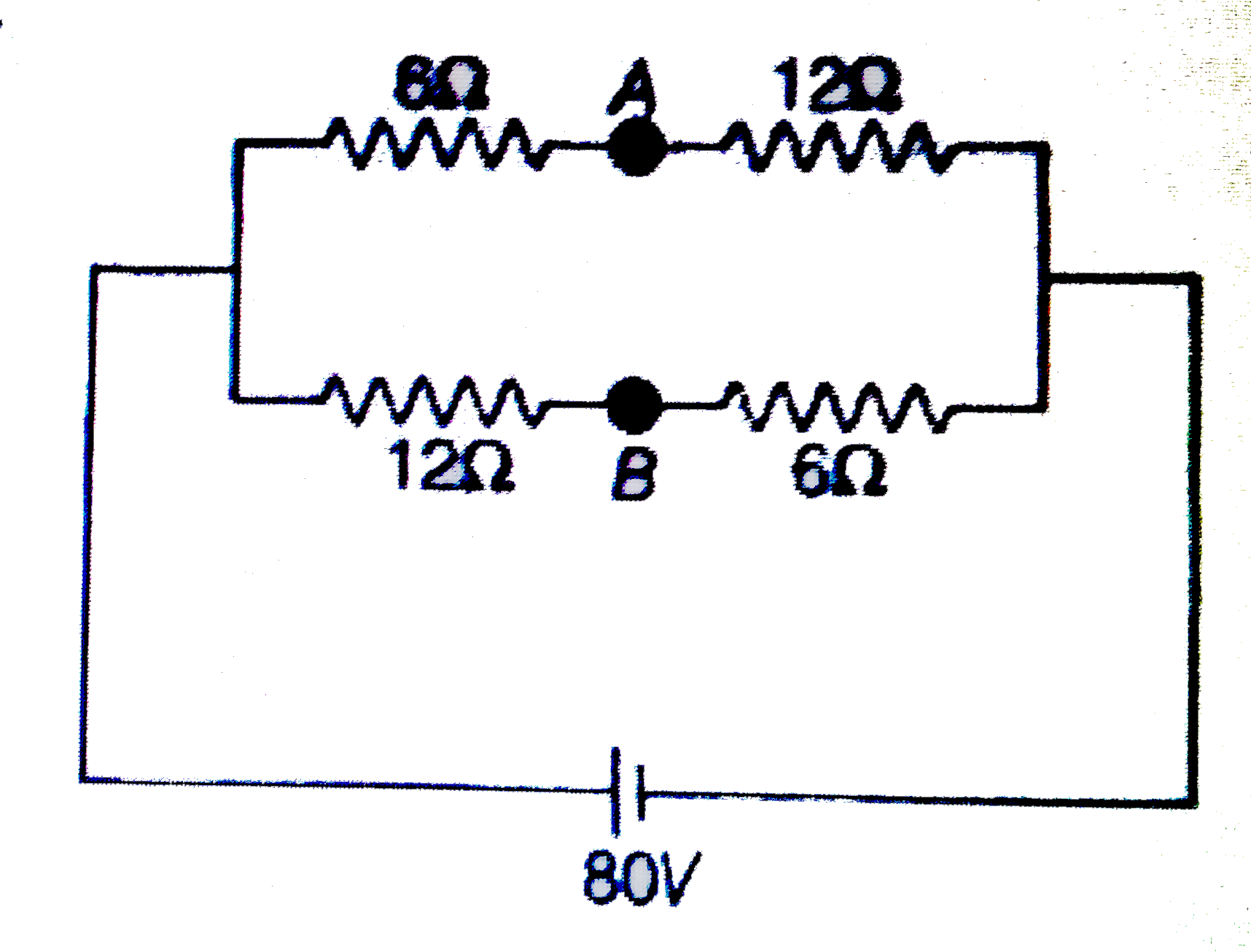
View Solution
