A layer of ice of 0∘C of thickness x1 is floating on a pond. If the atmospheric temperature is −7∘C. Show that the time taken for thickness of the layer of ice to increase from x1 to x2 is given by
t=pL2kT(x22−x21)
where p is the density of ice, k its thermal conductivity and L is the latent heat of fusion of ice.
When the temperature of the air is less than 0∘C, the cold air near the surface of the pond takes heat (latent) from the water which freezes in the fonm of layers. Consequently, the thickness of the ice layer keeps increasing with time. Let x be the thickness of the ice layer at a certain time. If the thickness is increased by dx in time dt, thn the amount of heat flowing through the salb in time dt is given by

Where A is the area of the layer of ice and −7∘C is the temperature of the surounding air. If dm is the mass of water frozen into ice, then Q=dm×L. But dm=Apdx, where p is the density of ice. Hence
Q=ApLdx (ii)
Equating (i) and (ii). we have
KATdtx=ApLdx or dt=pLkTxds
Integrating, we have
t∫0dt=pLkTx2∫x1 or t=pLkT∣∣∣x22∣∣∣x2x1=pL2kT(x22−x11)
Topper's Solved these Questions
Similar Questions
Bottom of a lake is at 0∘C and atmospheric temperature is −20∘C. If 1 cm ice is formed on the surface in 24 h, then time taken to form next 1 cm of ice is
View SolutionA layer of ice of thickness y is on the surface of a lake. The air is at a constant temperature −θ∘C and the ice water interface is at 0∘C. Show that the rate at which the thickness increases is given by
dydt=KθLρy
where, K is the thermal conductivity of the ice, L the latent heat of fusion and ρ is the density of the ice.
View Solution
Knowledge Check
A lake surface is exposed to an atmosphere where the temperature is <0∘C. If the thickness of the ice layer formed on the surface grows form 2cm to 4cm in 1 hour. The atmospheric temperature, Ta will be- (Thermal conductivity of ice K=4×10−3cal/cm/s/.∘C, density of ice =0.9gm/ice. Latent heat of fustion of ice =80cal/m. Neglect the change of density during the state change. Assume that the water below the ice has 0∘ temperature every where)
A−20.∘CB0.∘CC−30.∘CD15.∘C
Ice starts forming in lake with water at 0∘C and when the atmospheric temperature is −10∘C. If the time taken for 1cm of ice be 7 hours. Find the time taken for the thickness of ice to change from 1cm to 2cm
View SolutionA pond has a layer of ice of thickness 0.25 m on its surface the temperature of the atmosphere is 10∘C. Find out the time required to increase the thickness of the layer of ice 0.5mm. K of ice =2Wm−2K−1. Density of ice =900kg m−3 latent heat of fusion of ice =336KJ kg−1.
View SolutionOn a cold winter dry, the temperature of atmosphere is −T∘C. The cylindrical dram shown is made of insultating material and it contains water at 0∘C. If L is latent heat of fusion of ice, ρ is density of ice and KR is thermal conductivity of ice, the time taken for total mass of water to freeze is
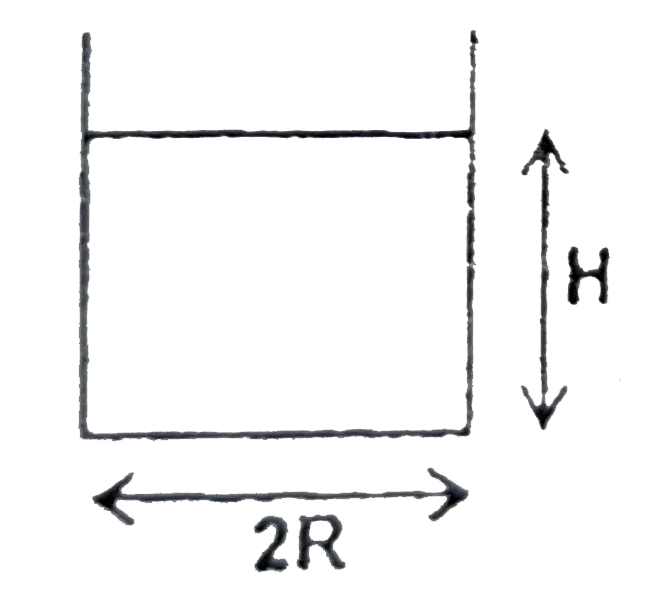
View SolutionThe thickness of ice on a Jake is 6 cm and the temperature of air is −10∘C. At what rate is the thickness of ice increasing ? Thermal conductivity of ice =2Wm−1K−1. Density of ice =920kg m3, specific latent heat of ice =336KJ kg−1
View SolutionA slab of stone of area of 0.36m2 and thickness 0.1m is exposed on the lower surface to steam at 100∘C. A block of ice at 0∘C rests on the upper surface of the slab. In one hour 4.8kg of ice is melted. The thermal conductivity of slab is
(Given latent heat of fusion of ice =3.63×105Jkg−1)
View SolutionOne end of a brass rod of length 2.0 m and cross section 1cm2 is kept in steam at 100∘C and the other end in ice at 0∘C. The lateral surface of the rod is covered by heat insulator. Determine the amount of ice melting per minute. Thermal conductivity of brass is 110W/m−K and specific latent heat of fusion of ice is 80cal/g.
View SolutionOne side of an iron plate one metre square is kept at 100°C and the other side is at 0°C. The thickness of the plate is 1 cm. If all the heat conducted across the plate in one minute is used in melting ice, calculate the amount of ice so melted. Thermal conductivity of iron =0.162 in C.G.S. unit L.H. of fusion of ice =80 cal/gm.
View Solution
