A cubical block of co-efficient of linear expansion is submerged partially inside a liquid of co-efficient of volume expansion . On increasing the temperature of the system by , the height of the cube inside the liquid remains unchanged. Find the relation between .
To find the relation between the coefficient of linear expansion of the solid cube (αs) and the coefficient of volumetric expansion of the liquid (γl), we can follow these steps:
Step 1: Understand the Problem
We have a cubical block partially submerged in a liquid. When the temperature of the system is increased by ΔT, the height of the cube submerged in the liquid remains unchanged. This indicates that the buoyant force acting on the cube must remain constant.
Step 2: Initial Volume of the Cube
Let the side length of the cube be a. The initial volume V of the cube is given by:
V=a3
The area of the base of the cube is:
A=a2
Step 3: Change in Volume of the Cube
When the temperature increases by ΔT, the volume of the cube changes due to linear expansion. The new volume V′ can be expressed as:
V′=A⋅h′=A⋅(h⋅(1+αsΔT))
Where h is the original height of the cube submerged in the liquid, and h′ is the new height after expansion.
Step 4: Change in Volume of the Liquid
The volume of the liquid will also change with temperature. The new volume of the liquid V′l can be expressed as:
V′l=Vl⋅(1+γlΔT)
Where Vl is the initial volume of the liquid.
Step 5: Buoyant Force
The buoyant force Fb acting on the cube is given by the weight of the liquid displaced:
Fb=ρl⋅Vsubmerged⋅g
Where ρl is the density of the liquid, and g is the acceleration due to gravity. Since the height of the cube submerged remains unchanged, the buoyant force must remain constant.
Step 6: Relate the Forces
Since the buoyant force remains unchanged, we can set up the equation:
ρl⋅Vsubmerged⋅g=ρ′l⋅V′submerged⋅g
Where ρ′l is the new density of the liquid after expansion. The new density can be expressed as:
ρ′l=ρl1+γlΔT
Step 7: Equate the Volumes
Since the height remains unchanged, we can equate the submerged volume before and after the temperature change:
A⋅h=A⋅h⋅(1+αsΔT)
This simplifies to:
1=(1+αsΔT)
Step 8: Final Relation
Now, using the relation for the volume expansion of the liquid and the condition for buoyancy, we can derive:
αs=2γl
Conclusion
Thus, the relation between the coefficient of linear expansion of the solid cube and the coefficient of volumetric expansion of the liquid is:
αs=2γl
We have a cubical block partially submerged in a liquid. When the temperature of the system is increased by ΔT, the height of the cube submerged in the liquid remains unchanged. This indicates that the buoyant force acting on the cube must remain constant.
Let the side length of the cube be a. The initial volume V of the cube is given by:
V=a3
The area of the base of the cube is:
A=a2
When the temperature increases by ΔT, the volume of the cube changes due to linear expansion. The new volume V′ can be expressed as:
V′=A⋅h′=A⋅(h⋅(1+αsΔT))
Where h is the original height of the cube submerged in the liquid, and h′ is the new height after expansion.
The volume of the liquid will also change with temperature. The new volume of the liquid V′l can be expressed as:
V′l=Vl⋅(1+γlΔT)
Where Vl is the initial volume of the liquid.
The buoyant force Fb acting on the cube is given by the weight of the liquid displaced:
Fb=ρl⋅Vsubmerged⋅g
Where ρl is the density of the liquid, and g is the acceleration due to gravity. Since the height of the cube submerged remains unchanged, the buoyant force must remain constant.
Since the buoyant force remains unchanged, we can set up the equation:
ρl⋅Vsubmerged⋅g=ρ′l⋅V′submerged⋅g
Where ρ′l is the new density of the liquid after expansion. The new density can be expressed as:
ρ′l=ρl1+γlΔT
Since the height remains unchanged, we can equate the submerged volume before and after the temperature change:
A⋅h=A⋅h⋅(1+αsΔT)
This simplifies to:
1=(1+αsΔT)
Now, using the relation for the volume expansion of the liquid and the condition for buoyancy, we can derive:
αs=2γl
Thus, the relation between the coefficient of linear expansion of the solid cube and the coefficient of volumetric expansion of the liquid is:
αs=2γl
Topper's Solved these Questions
Similar Questions
A cube of coefficient of liear expansion αs is floating in a bath containing a liquid of coefficient of volume expansion γL. When the temperature is raised by ΔT, the depth upto which the cube is submerged in the liquid remains the same. Find the relation between αs and γL showing all the steps.
View SolutionA cube of coefficient of linear expansion α is floating in a bath containing a liquid of coefficient of volume expansion γl. When the temperature is raised by ΔT, the depth upto which the cube is submerged in the liquid remains the same. Find relation between α and γl
View SolutionA solid cube is first floating in a liquid. The coefficient of linear expansion of cube is α and the coefficient of volume expansion of liquid is γ. On increasing the temperature of (liquid + cube) system, the cube will sink if
 View Solution
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionA liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
View SolutionLet γ : coefficient of volume expansion of the liquid and α : coefficient of linear expansion of the material of the tube
View SolutionA rod has variable co-efficient of linear expansion α=x5000. If length of the rod is 1 m . Determine increase in length of the rod in (cm) on increasing temperature of the rod by 100∘C.
_S01_121_Q01.png) View Solution
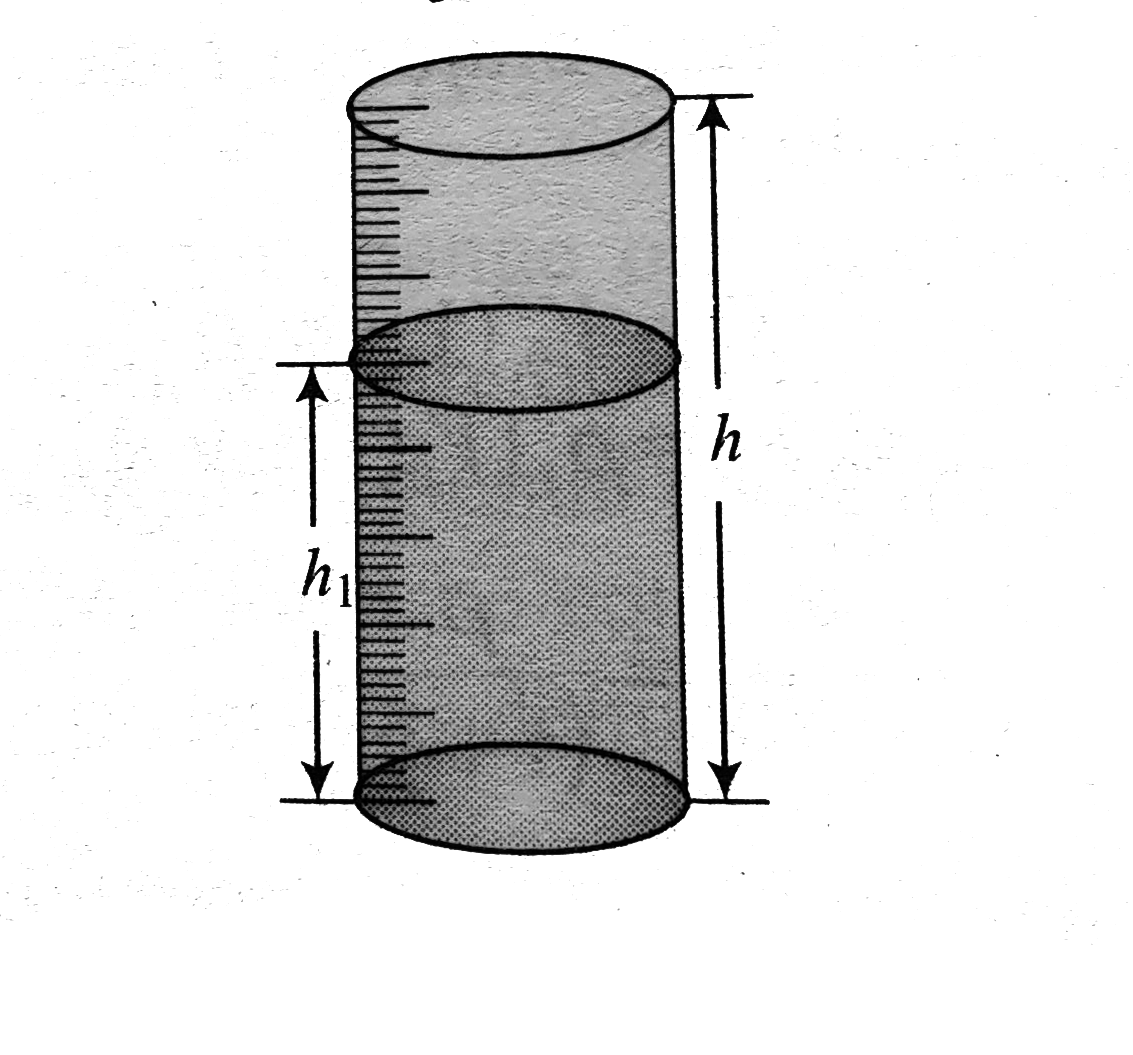
View SolutionConsider a cylindrical container of cross-section area A length h and having coefficient of linear expansion αc. The container is filled by liquid of real expansion coefficient γL up to height h1. When temperature of the system is increased by Δθ then
(a). Find out the height, area and volume of cylindrical container and new volume of liquid.
(b). Find the height of liquid level when expansion of container is neglected.
(c). Find the relation between γL and αc for which volume of container above the liquid level
(i) increases
(ii). decreases
(iii). remains constant.
(d). On the surface of a cylindrical container a scale is attached for the measurement of level of liquid of liquid filled inside it. If we increase the temperature of the temperature of the system by Δθ, then
(i). Find height of liquid level as shown by the scale on the vessel. Neglect expansion of liquid.
(ii). Find the height of liquid level as shown by the scale on the vessel. Neglect expansion of container.
 View Solution
View SolutionA beaker of height H is made up of a material whose coefficient of linear thermal expansion is 3α. It is filled up to the brim by a liquid whose coefficient of volume expansion is α. If now the beaker along with its contents is uniformly heated through a small temperature T, the level of liquid will reduced by (Given, αT<<1)
View SolutionAn isosceles triangles is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is
 View Solution
View Solution

