A uniform plank of mass m, free to move in the horizontal direction only , is placed at the top of a solid cylinder of mass 2m and radius R. The plank is attached to a fixed wall by mean of a light spring constant k. There is no slipping between the cylinder and the planck , and between the cylinder and the ground. Find the time period of small oscillation of the system.
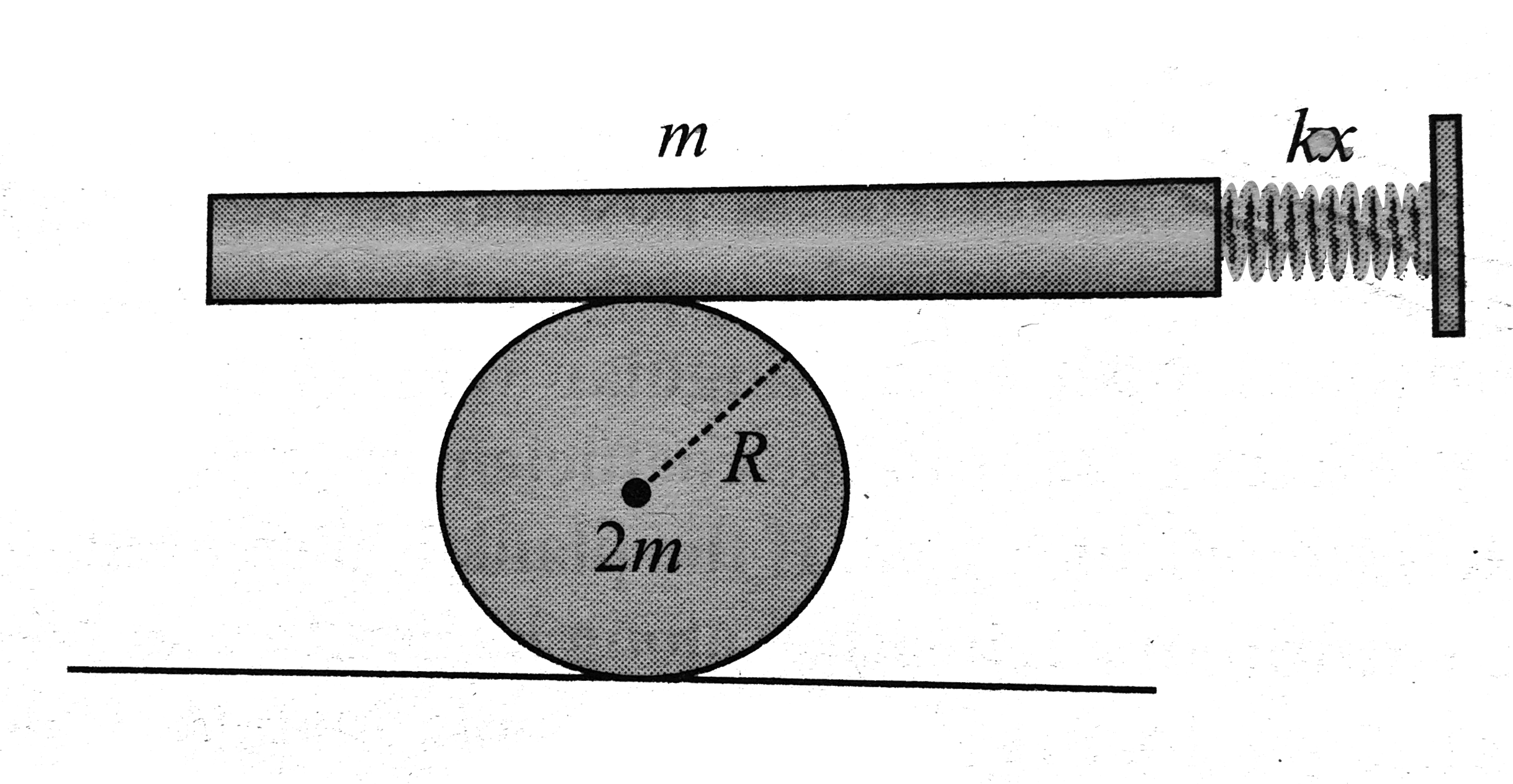
Method 1: When the plank is displaced slightly (say x) from equilibrium , as there is no sliding at any surface , the friction will be of static nature . As cylinder is rolling we can take the motion of the cylinder as pure rotation about point of contact.
Free body diagrams of plank and cylinder

Equation of motion for plank
kx−f=m(a)
Writing torque equation to cylinder about plank of contact
f−(2R)=ip.α[12(2m)R2+2mR2]α
=3mR2α
or f=32mRa
As cylinder is rolling we can write, the acceleration of topmost point of cylinder (or plank)
a=α(2R)⇒αR=a2
Substituting the value of (iii) and (ii), we get f=34ma
Now, from Eqs (i) and (iv),
kx−(34ma)=ma
⇒a=4k7mx
Wtiting above equation with proper vector relation
¯a=−(4k7m)¯x
Comparing with stander equation ¯a=−ω2¯x
which gives ω2=4k7morT=π√7mk
Method 2: Suppose that the plank is displaced from its equilibrium position by x at time t , the center of the cylinder is therefore , displaced by x/2.
Therefore the mechanical energy of the system is given by
E=KE(plank)+PE(spring)+KE(cylinder)
=12mv2+12kx2+12Ipω2
IP=IG+(2m)R2=12(2m)R2+(2m)R2
=3mR2
As cylinder is rolling velocity of center of cylinder will be half of the velocity of the plank (top most point of the cylinder)
νcyl∈der=ν2=ωR⇒ω=ν2R
Substituting the value of ω in Eq. (i) we get
E=12mv2+12kx2+12(3mR2)(ν2R)
=12mv2+12kx2+38mv2
=78mv2+12kx2
Differentiating Eq. (ii) w.r.t. time
dEdt=0=78m.2v(dνdt)+k.2x,(dxdt
=78mν.a+kx.ν
Here 74ma+kx=0ora=−4k7mx
Comparing with a=−ω2x⇒T=2π√7m4k=π√7mk
Method 3: we can write Eq (ii)as E=ν2+4k7ms2=constant
as total energy of sysytem performing SHM is constant. Comparing with standard equation ν2+ω2x2=constant
which gives ω2=4k7morT=π√7mk
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Exercise 4.1
Explore 23 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Exercise 4.2
Explore 23 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Interger
Explore 11 Videos
- MISCELLANEOUS KINEMATICSBook:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS KINEMATICSExercise:Interger type
Explore 3 Videos
Similar Questions
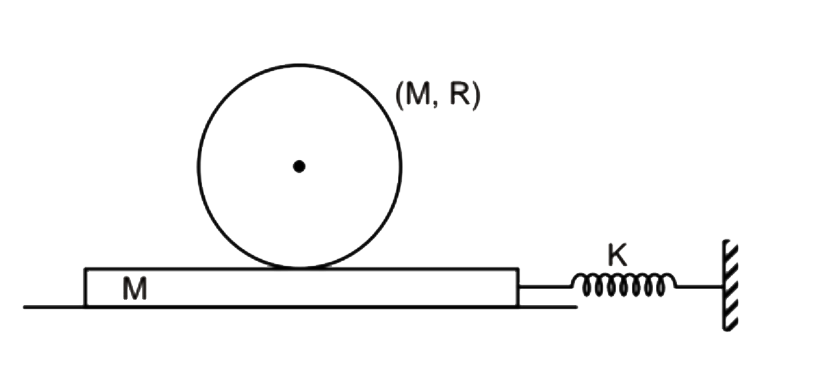
A cylinder of mass M and radius R lies on a plank of mass M as shown. The surface between plank and ground is smooth, and between cylinder and plank is rough. Assuming no slipping between cylinder and plank, the time period of oscillation (When displaced from equilibrium) of the system is
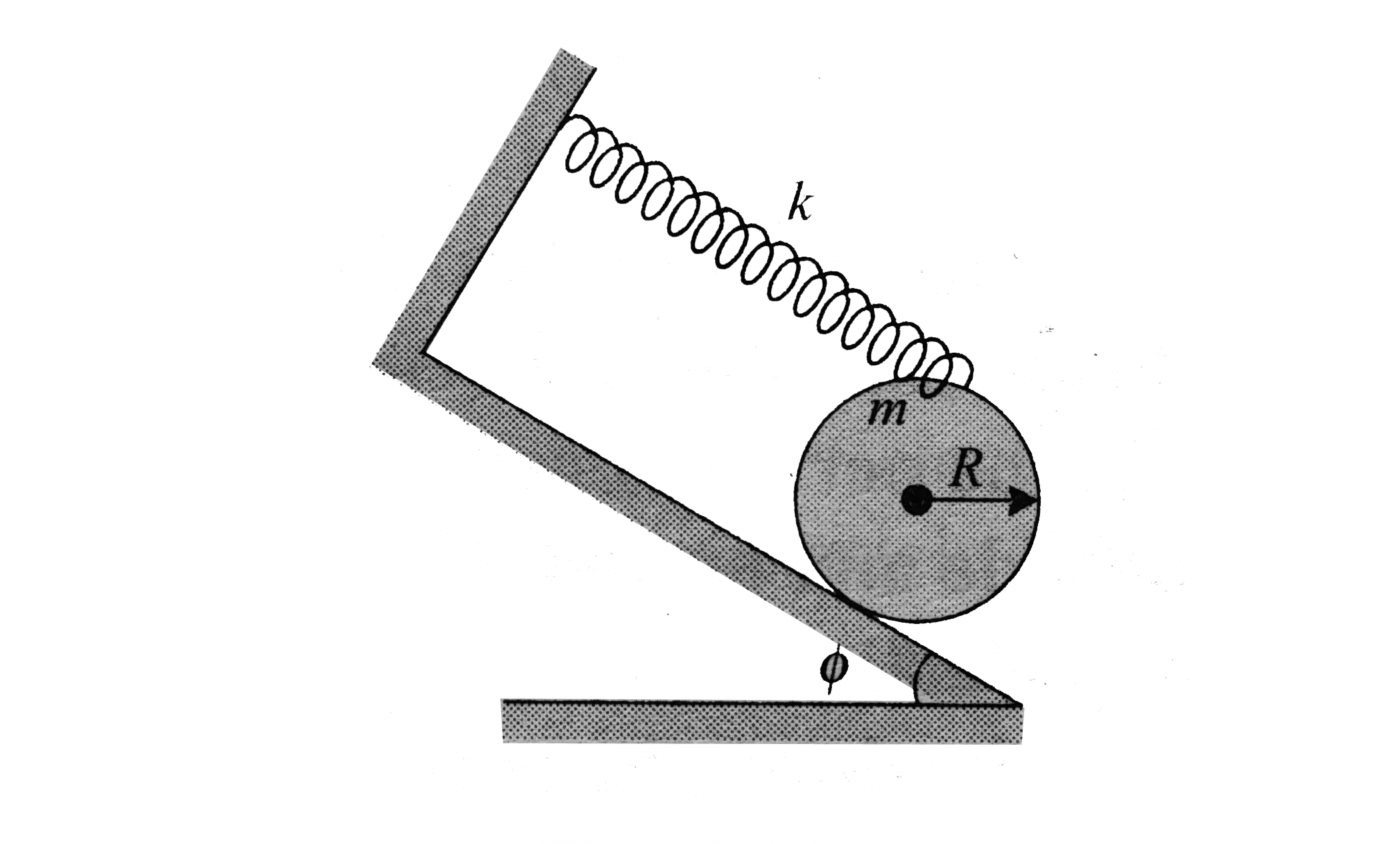
View SolutionA uniform cylinder of mass m and radius R is in equilibrium on an inclined by the action of a light spring of stiffnesk, gravity and reaction force acting on it .If the angle of inclination of the plane is ϕ , find the angular frequency of small oscillation of the cylinder
View Solution
Knowledge Check
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.
A1/3 g sinθB2/3 g sinθC2/5 g sinθD2/7 g sinθ
A solid sphere of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between sphere and plank to prevent slipping. Force of friction between sphere and plank is

View SolutionFind the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder.
View SolutionA solid cylinder of mass M and radius R rolls without slipping down an inclined plane of length L and height h. What is the speed of its center of mass when the cylinder reaches its bottom
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View Solution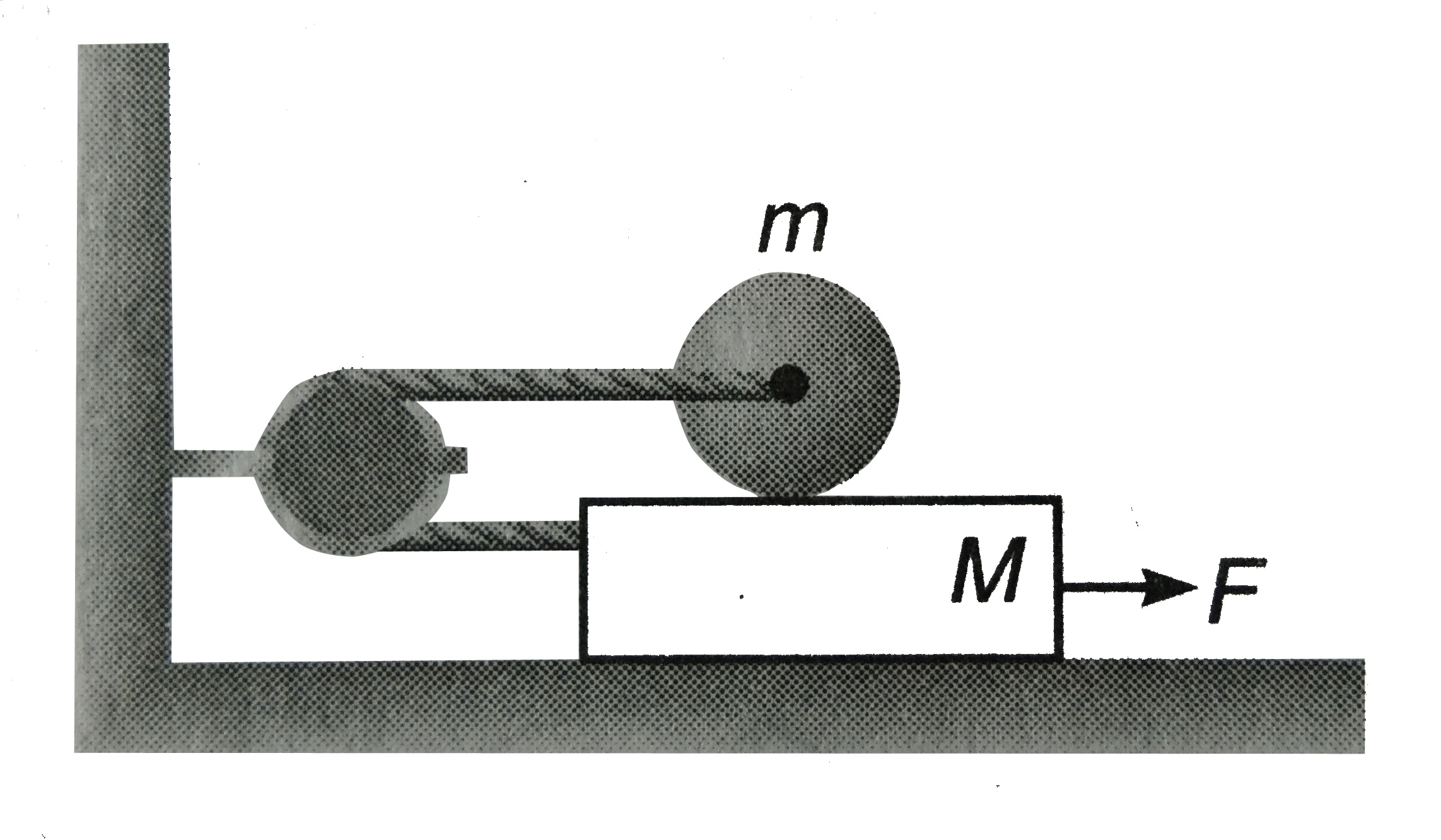
Find the acceleration of the cylinder of mass m and radius R and that of plank of mass M placed on smooth surface if pulled with a force F as shown in figure. Given that sufficient friction is present between cylinder and the plank surface to prevent sliding of cylinder.
View Solution
A uniform solid cylinder having mass M and radius R is pulled by a horiozntal force F acting through the center as shown. The cylinder rolls to the right without slipping. What is the magnitude of the force of friction between the cylinder and the ground?
View SolutionA bullet of mass m moving with a velocity of u just grazes the top of a solid cylinder of mass M and radius R resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

View Solution
