Small amplitude progressive wave in a stretched string has a speed of 100cm/s.and frequency 100 Hz. The phase difference between two points 2.75 cm apart on the string in radians, is
0
11π/2
π/4
3π/8
The correct Answer is:B
To solve the problem of finding the phase difference between two points 2.75 cm apart on a stretched string with a wave speed of 100 cm/s and a frequency of 100 Hz, we can follow these steps:
1. Identify Given Values:
- Speed of the wave, v=100cm/s
- Frequency of the wave, f=100Hz
- Distance between two points, Δx=2.75cm
2. Calculate the Wavelength (λ):
- Use the formula for wave speed:
v=f⋅λ
- Rearranging gives:
λ=vf
- Substituting the values:
λ=100cm/s100Hz=1cm
3. Calculate the Phase Difference (Δϕ):
- The formula for phase difference is:
Δϕ=2πλ⋅Δx
- Substituting the values we have:
Δϕ=2π1cm⋅2.75cm
- Simplifying this gives:
Δϕ=2π⋅2.75=5.5π
4. Convert to Fractional Form:
- We can express 5.5π as:
5.5π=112π
5. Final Answer:
- The phase difference between the two points is:
Δϕ=11π2radians
Summary:
The phase difference between the two points 2.75 cm apart on the string is 11π2 radians.
- Speed of the wave, v=100cm/s
- Frequency of the wave, f=100Hz
- Distance between two points, Δx=2.75cm
- Use the formula for wave speed:
v=f⋅λ
- Rearranging gives:
λ=vf
- Substituting the values:
λ=100cm/s100Hz=1cm
- The formula for phase difference is:
Δϕ=2πλ⋅Δx
- Substituting the values we have:
Δϕ=2π1cm⋅2.75cm
- Simplifying this gives:
Δϕ=2π⋅2.75=5.5π
- We can express 5.5π as:
5.5π=112π
- The phase difference between the two points is:
Δϕ=11π2radians
The phase difference between the two points 2.75 cm apart on the string is 11π2 radians.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer1videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct25videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Subjective16videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A progressive wave moves with a velocity of 36m/ s in a medium with a frequency of 200Hz. The phase difference between two particles seperated by a distance of 1 cm is
View SolutionThe speed of a wave in a streched string is 20ms−1 and its frequency is 50 Hz. Calculate the phase difference in radian between two points situated at a distance of 10 cm on the string.
View SolutionConsider a sinusoidal travelling wave shown in figure. The wave velocity is +40cm/s.
Find
(a) the frequency
(b) the phase difference between points 2.5cm apart
(c) the velocity of a particle at P at the instant shown.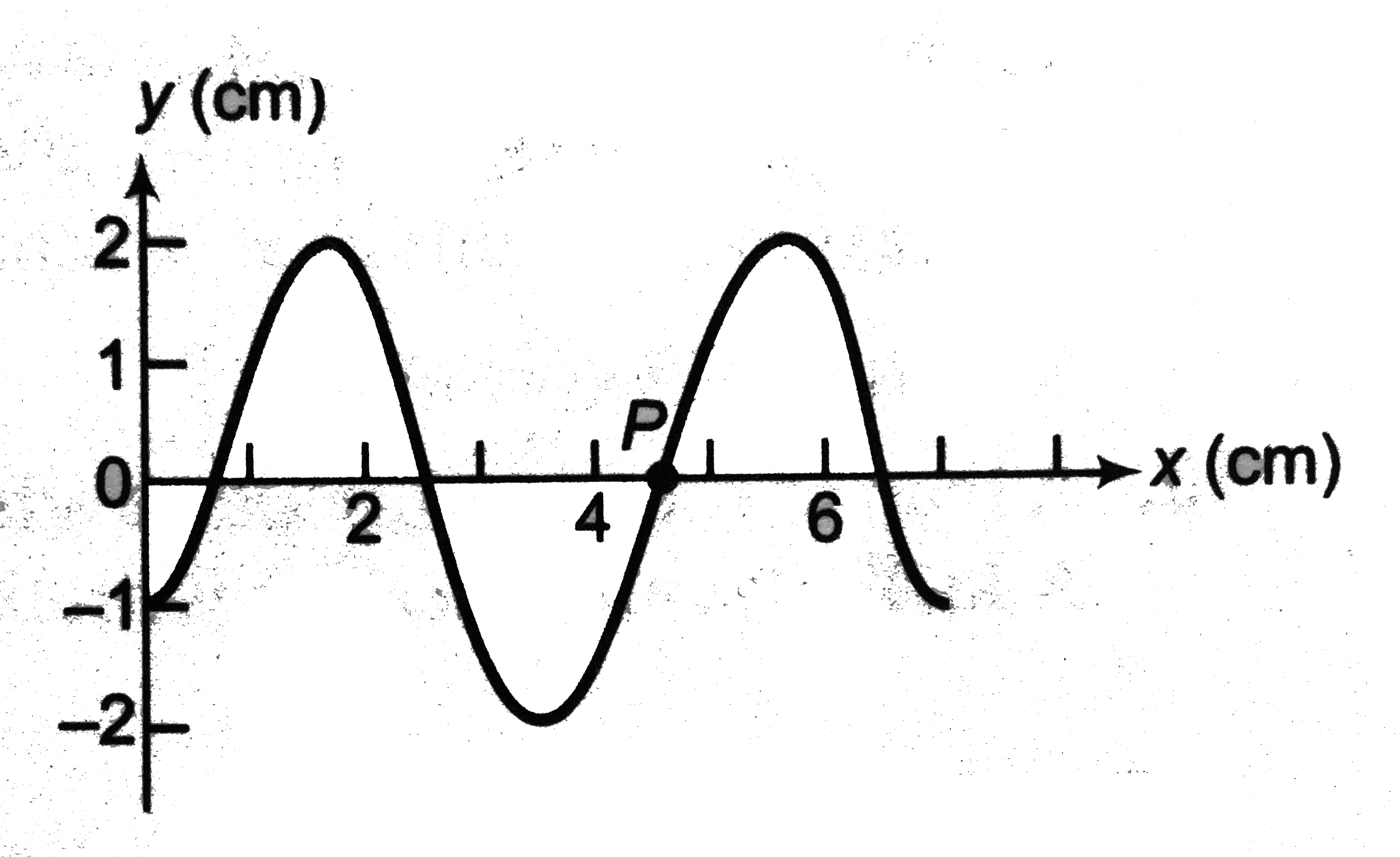 View Solution
View SolutionA plane progessive wave of frequency 25Hz, amplitude 2.5×10−5m and initial phase zero propagates along the negative x-direction with a velocity of 300m/s. At any instant, the phase difference between the oscillations at two points 6m apart the line of propagation is _____ .
View SolutionWave of frequency 500 Hz has a phase velocity 360m/s. The phase difference between two displacement at a certain point at time 10−3s apart will be
View SolutionThe angular frequency of a particle in a progressive wave in an elastic medium is 100πrads−1 and it is moving with a velocity of 200ms−1 The phase difference between two particles seperated by a distance of 20m is
View SolutionA wave of frequency 20 Hz is propagatinig is space with speed 200 m/s. the phase difference of the two points of wave separated by a distance 5 m is
View SolutionA 12 m long vibrating string has the speed of wave 48 m/s . To what frequency it will increase .
View SolutionA transverse sine wave of amplitude 10 cm and wavelength 200 cm travels from left to right along a long horizontal stretched, string with a speed of 100 cm/s. Take the origin at left end of the string. At time t = 0 the left end of the string is at the origin and is moving downward. Then the equation of the wave will be ( in CGS system )
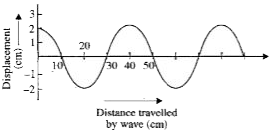
View SolutionA wave travelling along the length of a string is shown in Fig. 14.4.12. Using the scale on the given axis, find
(i) The amplitude of the wave
(ii) The wavelength of the wave
(iii) If the frequency of the wave is 10 Hz, What is the speed of the wave?
(iv) What is the initial phase of the wave ?
(v) What is the phase difference between two points separated by a distance 10 cm ?
(vi) If this wave is made to travel through water what is the wavelength of the wave, if the speed of, the waves in water is 10 m/s.
 View Solution
View Solution

