Two boats A and B having same speed relative to river are moving in a river. Boat A moves normal to the river current as observed by an observer moving with velocity of river current. Boat B moves normal to the river as observed by the observer on the ground.
To a ground observer Boat B moves faster than A
To a ground observer boat A moves faster than B
to the given moving observer boat B moves faster than A
To the given moving observer the speeds of boat A and B are same
The correct Answer is:B, D
To solve the problem, we need to analyze the motion of both boats A and B in relation to the river current and the observers.
1. Understanding the Setup:
- We have two boats, A and B, moving in a river with the same speed relative to the river.
- Boat A moves perpendicular (normal) to the river current as observed by an observer who is moving with the velocity of the river current.
- Boat B moves perpendicular (normal) to the river current as observed by an observer on the ground.
2. Defining the Velocities:
- Let the speed of both boats relative to the river be v.
- Let the speed of the river current be u.
3. Analyzing Boat A:
- For the observer moving with the river current, Boat A moves directly across the river. Its velocity relative to this observer is v (perpendicular to the current).
- However, from the perspective of a ground observer, Boat A also has a component of velocity in the direction of the river current due to the river's flow.
- The resultant velocity of Boat A as seen by the ground observer can be calculated using the Pythagorean theorem:
VA=√v2+u2
- This is because Boat A has a velocity v across the river and u downstream due to the river current.
4. Analyzing Boat B:
- For Boat B, which moves perpendicular to the river as observed from the ground, its velocity relative to the ground is simply v (perpendicular to the current) since it is not affected by the river's current in the direction of its motion.
- The velocity of Boat B remains v as it moves straight across the river.
5. Comparing the Velocities:
- From the ground observer's perspective:
- The velocity of Boat A is VA=√v2+u2.
- The velocity of Boat B is VB=v.
- Since √v2+u2>v (because u>0), it follows that:
VA>VB
6. Conclusion:
- To a ground observer, Boat A moves faster than Boat B.
Final Answer:
To a ground observer, Boat A moves faster than Boat B.
- We have two boats, A and B, moving in a river with the same speed relative to the river.
- Boat A moves perpendicular (normal) to the river current as observed by an observer who is moving with the velocity of the river current.
- Boat B moves perpendicular (normal) to the river current as observed by an observer on the ground.
- Let the speed of both boats relative to the river be v.
- Let the speed of the river current be u.
- For the observer moving with the river current, Boat A moves directly across the river. Its velocity relative to this observer is v (perpendicular to the current).
- However, from the perspective of a ground observer, Boat A also has a component of velocity in the direction of the river current due to the river's flow.
- The resultant velocity of Boat A as seen by the ground observer can be calculated using the Pythagorean theorem:
VA=√v2+u2
- This is because Boat A has a velocity v across the river and u downstream due to the river current.
- For Boat B, which moves perpendicular to the river as observed from the ground, its velocity relative to the ground is simply v (perpendicular to the current) since it is not affected by the river's current in the direction of its motion.
- The velocity of Boat B remains v as it moves straight across the river.
- From the ground observer's perspective:
- The velocity of Boat A is VA=√v2+u2.
- The velocity of Boat B is VB=v.
- Since √v2+u2>v (because u>0), it follows that:
VA>VB
- To a ground observer, Boat A moves faster than Boat B.
To a ground observer, Boat A moves faster than Boat B.
Similar Questions
River Boat Problem
View SolutionA boat moves perpendicular to the bank with a velocity of 7.2 km/h. The current carries it 150 m downstream, find the velocity of the current. (The width of the river is 0.5 km).
View SolutionA boat which has a speed of 6km/h in still water crosses a river of width 1 km along the shortest possible path in 20 min. the velocity of the river water in km/h is
View SolutionTwo boats A and B moved away from a buoy anchored in the middle of a river along the mutually perpendicular straight lines. A moved along the river and B at fight angle to it Having moves off equal distances from the boy, the boats returned. Find the ratio of the times of motion of the boats, if the velocity of each boat with respect to still water in η=1.2 times greater than the velocity of water current.
View Solution
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. The boat crosses the river from A to the other side in shortest possible time, then how far is the boat from the port B after crossing the river
View Solution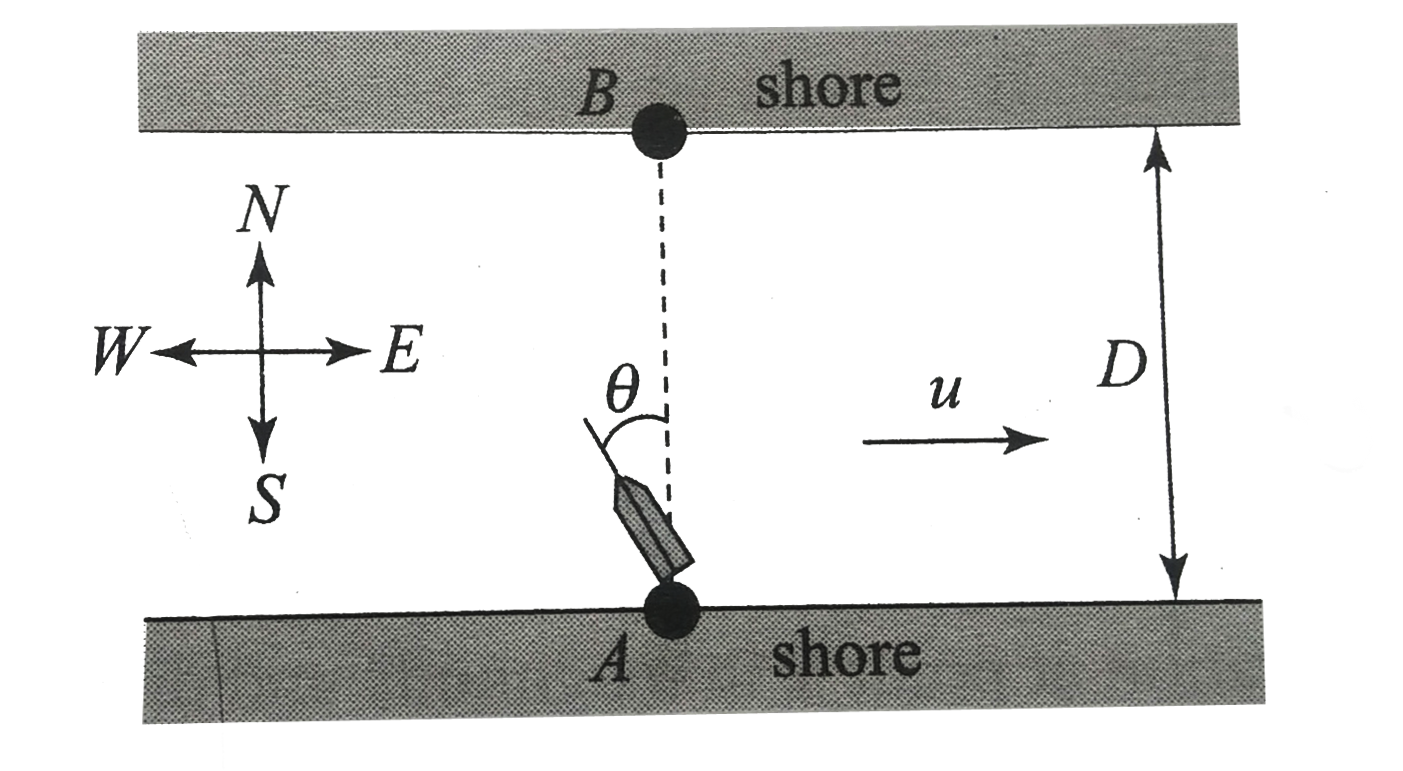
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. What is the direction of the velocity of boat relative to river θ, sothat it crosses directly on a line from A to B?
View SolutionTwo boats A and B are moving along perpendicular paths in a still lake at night. Boat A move with a speed of 3m/s and boat B moves with a speed of 4m/s in the direction such that they collide after sometime. At t=0, the boats are 300m apart. The ratio of distance of distance travelled by boat A to the distance travelled by boat B at the instant of collision is :-
View SolutionA boat is moving with a velocity 3ˆi+4ˆj with respect to ground. The water in the river is moving with a velocity −3ˆi−4ˆj with respect to ground. The relative velocity of the boat with respect to water is.
View Solution
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. Suppose the boat wants to cross the river from A to the other side in the shortest possible time. Then what should be the direction of the velocity of boat relative to river?
View SolutionA boat crosses a river with a velocity of 8kmh. If the resulting velocity of boat is 10kmh then the velocity of river water is
View Solution
