A perfectly straight protion of a uniform rope has mass M and length L . At end A of the segment, the tension in the rope is TA and at end B it is TB(TB>TA) . Neglect effect of gravity and no contact force acts on the rope in between points A and B . The tension in the rope at a distance L/5 from end A is.
TB−TA
(TA+TB)5
(4TA+TB)5
(TA−TB)5
The correct Answer is:C
To solve the problem step by step, we will analyze the tension in the rope at a distance L5 from point A.
Step 1: Understand the setup
We have a uniform rope of mass M and length L. The tension at point A is TA and at point B is TB (with TB>TA). We need to find the tension TC at a point C which is located at a distance L5 from point A.
Hint: Visualize the rope and label the points clearly to understand the tension distribution.
Step 2: Define the relationship between tensions
The difference in tension between points B and A can be related to the mass and acceleration of the rope segment. We can express this as:
TB−TA=M⋅a
where a is the acceleration of the rope.
Hint: Remember that the net force acting on the rope segment is equal to the mass of the segment multiplied by its acceleration.
Step 3: Analyze the segment from A to C
For the segment from A to C (length L5), the mass of this segment is M5. The tension difference can be expressed as:
TC−TA=M5⋅a
Hint: Use the mass of the segment to relate the tension at point C to the tension at point A.
Step 4: Substitute the acceleration
From Step 2, we know that:
a=TB−TAM
Substituting this expression for a into the equation from Step 3 gives:
TC−TA=M5⋅(TB−TAM)
Hint: This substitution allows you to eliminate a and express everything in terms of TA and TB.
Step 5: Simplify the equation
Now, simplifying the equation:
TC−TA=15(TB−TA)
Rearranging gives:
TC=TA+15(TB−TA)
Hint: This step combines the tensions effectively to find TC.
Step 6: Combine and finalize the expression
Distributing the term on the right-hand side:
TC=TA+15TB−15TA
Combining like terms results in:
TC=45TA+15TB
This can be rewritten as:
TC=4TA+TB5
Hint: Ensure that you combine the terms correctly to arrive at the final expression for TC.
Final Answer
The tension in the rope at a distance L5 from end A is:
TC=4TA+TB5
We have a uniform rope of mass M and length L. The tension at point A is TA and at point B is TB (with TB>TA). We need to find the tension TC at a point C which is located at a distance L5 from point A.
The difference in tension between points B and A can be related to the mass and acceleration of the rope segment. We can express this as:
TB−TA=M⋅a
where a is the acceleration of the rope.
For the segment from A to C (length L5), the mass of this segment is M5. The tension difference can be expressed as:
TC−TA=M5⋅a
From Step 2, we know that:
a=TB−TAM
Substituting this expression for a into the equation from Step 3 gives:
TC−TA=M5⋅(TB−TAM)
Now, simplifying the equation:
TC−TA=15(TB−TA)
Rearranging gives:
TC=TA+15(TB−TA)
Distributing the term on the right-hand side:
TC=TA+15TB−15TA
Combining like terms results in:
TC=45TA+15TB
This can be rewritten as:
TC=4TA+TB5
The tension in the rope at a distance L5 from end A is:
TC=4TA+TB5
Similar Questions
A pulse is generated at lower end to a hanging rope of uniform density and length L. The speed of the pulse when it reaches the mid point of rope is
 View Solution
View SolutionA lift is going up with variable velocity as shown in figure if mass of the lift is 2000 kg Then tension in the rope of lift at t = 5 second is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A rope of length L is pulled by a constant force F. What is the tension in the rope at a distance x from the end where the force is applied ?
AFL/xBF(L−x)/LCFL/(L−x)DFx/(L−x)
Tension in a uniform string of length L varies with length uniformly. At one end A tension is T0 and at other end B it is 16T0. If mass per unit length of string is μ. Choose the correct options (s)
View SolutionA horizontal uniform rope of length L, resting on a frictionless horizontal surface, is pulled at one end by force F. What is the tension in the rope at a distance l from the end where the force is applied?
View SolutionA uniform rope of mass m and length L hangs from a celling. (a) Show that the speed of a transverse wave on the rope is a function of y, the distance from the lower end, and is given by t=2√L/g.
View SolutionA uniform rope of length L, resting on a frictionless horizontal table is pulled at one end by a force F. What is the tension in the rope at a distance x form the end where the force is applied?
View SolutionA uniform rope of mass M length L hangs vertically from the ceiling, with lower end free. A distbance on the rope trvelling upwards starting from the lower end has a velocity v. At a point P at distance x from the lower end.
View SolutionA rope of length 5 m has uniform mass per unit length. λ=2kgm The rope is pulled by a constant force of 10N on the smooth horizontal surface as shown in figure The tension in the rope at x = 2 m from polit A is
View SolutionA uniform rope of mass (m) and length (L) placed on frictionless horizontal ground is being pulled by two forces FAandFB at its ends as shown in the figure. As a result, the rope accelerates toward the right.
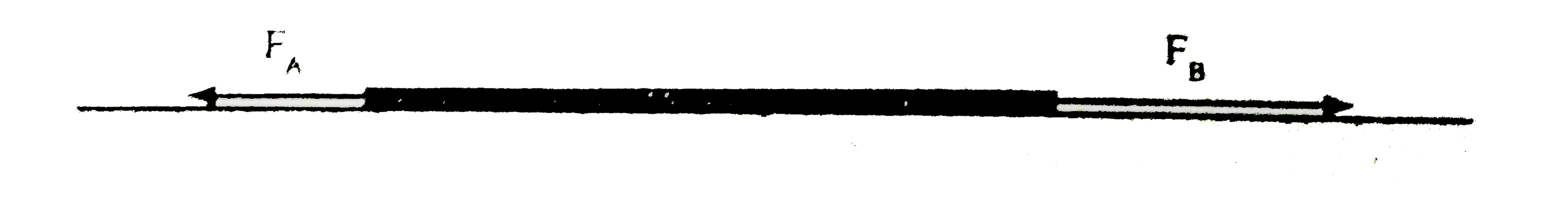
Expression Tx of tension at a point at distance x from the end A isView Solution

