
In the figure shown , the coefficient of static friction between B and the wall is 23 and the coefficient of kinetic friction between B and the wall is 13. Other contacts are smooth. Find the minimum force F required to lift B, up. Now if the force applied on A is slightly increased than the calculated value of minimum force, then find the acceleration of B. mass of A is 2m and the mass of B is m. Take tanθ=34


The FBDs of A and B are
For A to be in equilibrium:
F=Nsinθ .(1)
For B to just lift off,
Nsinθ=mg+μSN).(2)<br>ForhorizontalequilibriumofB,<br>N^()=Nsinθ .(3)
From (2) and (3)
N(cosθ−μSsinθ)=mg or N(45−23×35)mg
or N=52mg .(4)
From equation (1) F=N×35
∵ F=32mg
(ii) The acceleration of the block A be a and B be b
F−Nsinθ=2ma .(1)
Ncosθ−mg−μSN)=mb.(2)<br>N^()=Nsinθ .(3)
From constraint =
asinθ=bcosθ
Solving (1),(2),(3) and (4) we get
⇒b=3g7
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Single Correct Answer type
491 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type
22 Videos
Similar Questions
If the coefficient of friction between all surface figure is 0.4 then find the minimum force F to have equilibrium of the system.
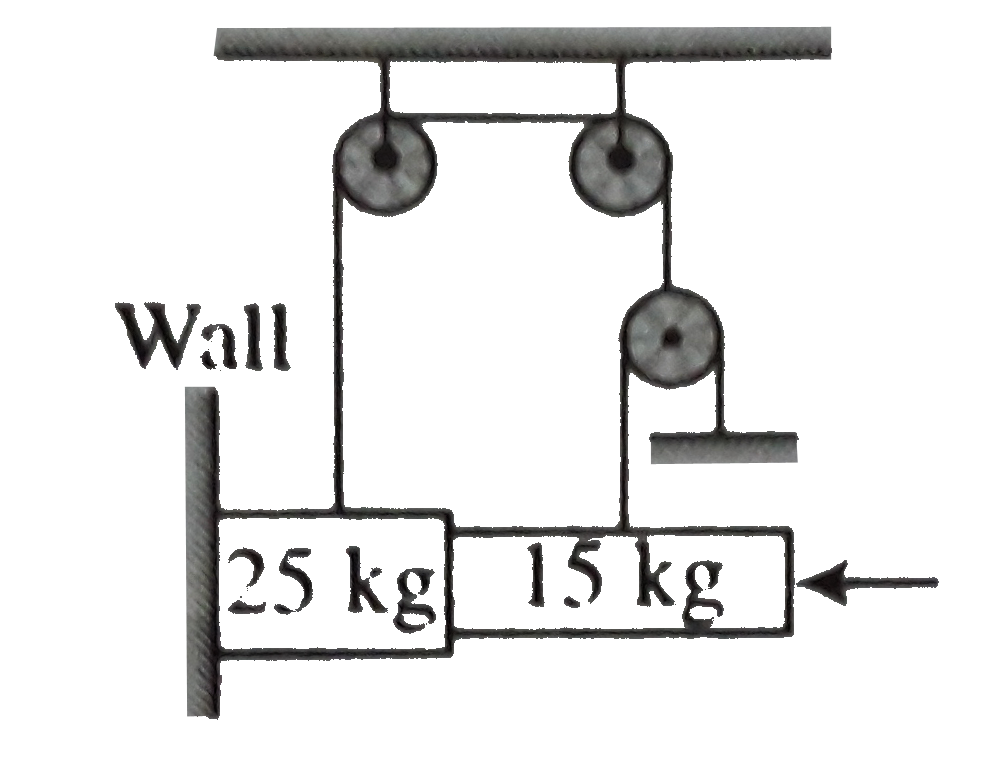
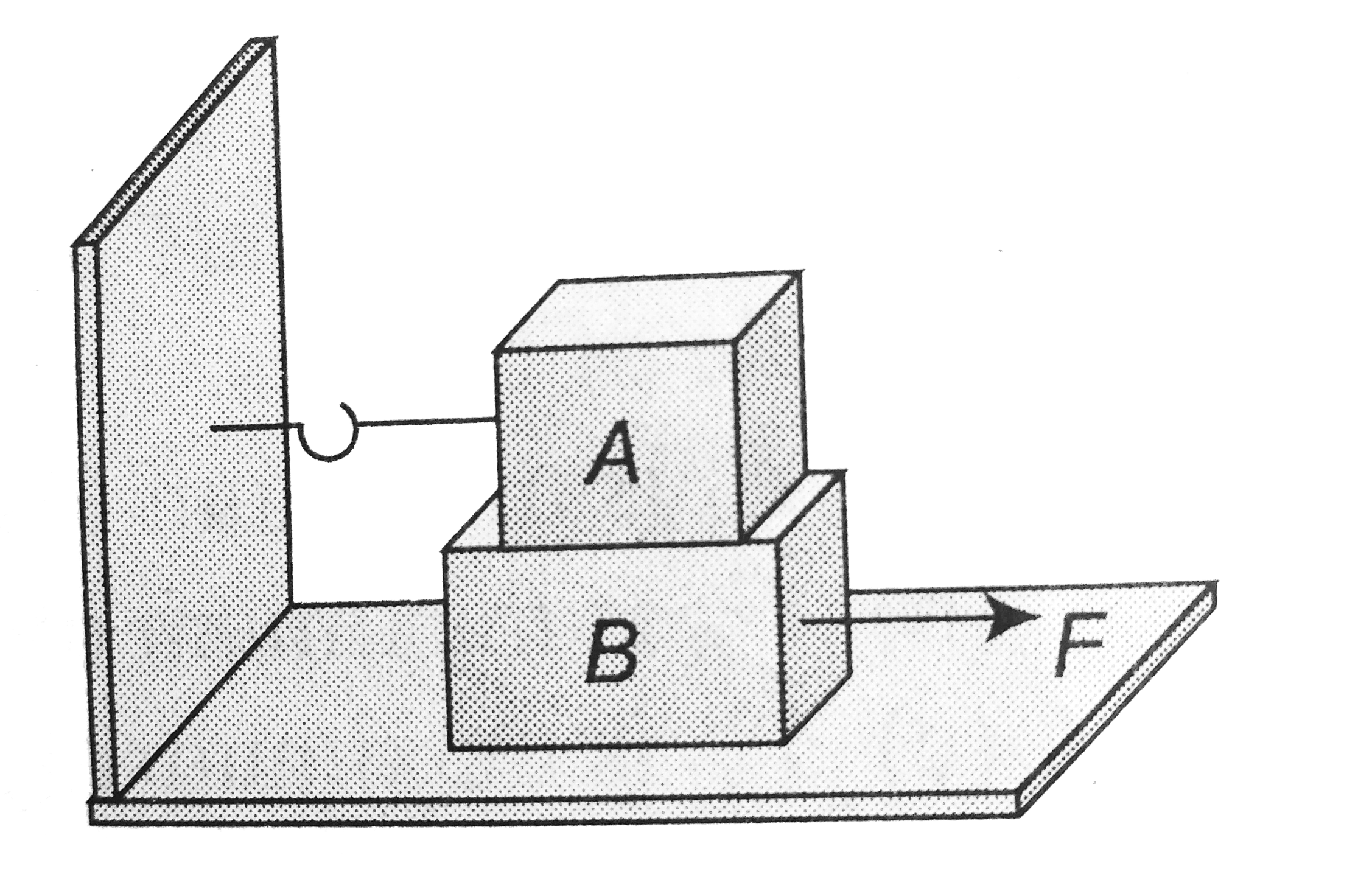
View SolutionA block A with mass 100kg is resting on another block B of mass 200kg. As shown in figure a horizontal rope tied to a wall hold it. The coefficient of friction between A and B is 0.2 while coefficient of friction between B and the ground is 0.3 . the minimum required force F to start moving B will be.
View Solution
Knowledge Check
If the coefficient of friction between all surface figure is 0.4 then find the minimum force F to have equilibrium of the system.
 A62.5NB150NC31.25ND50N
A62.5NB150NC31.25ND50NA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
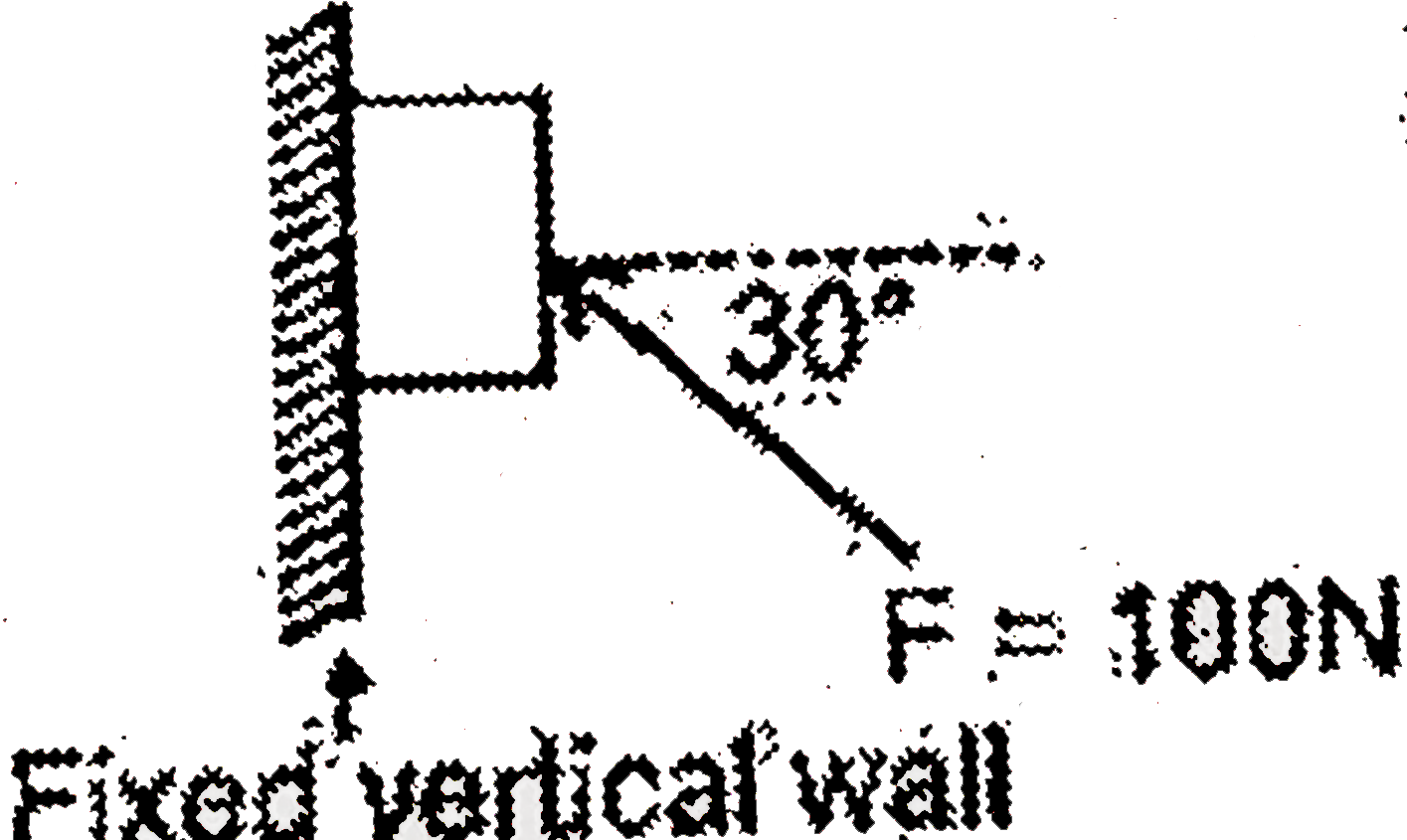 A15 N downwardsB25 N upwardsC20 N downwardsD20 N upwards
A15 N downwardsB25 N upwardsC20 N downwardsD20 N upwards
If the mass of A=10 kg, coefficient of static friction=0.22, coefficient of kinetic friction=0.2, then minimum mass of B to start motion is
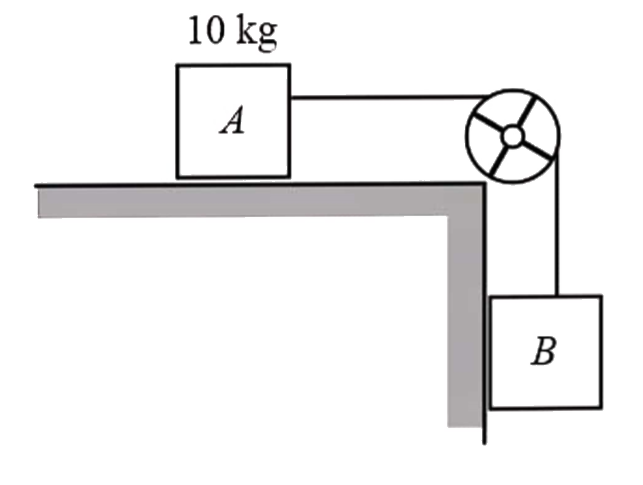
View SolutionIf the mass of A=10 kg, coefficient of static friction=0.22, coefficient of kinetic friction=0.2, then minimum mass of B to start motion is

View SolutionIf the mass of A=10 kg, coefficient of static friction=0.22, coefficient of kinetic friction=0.2, then minimum mass of B to start motion is

View SolutionA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
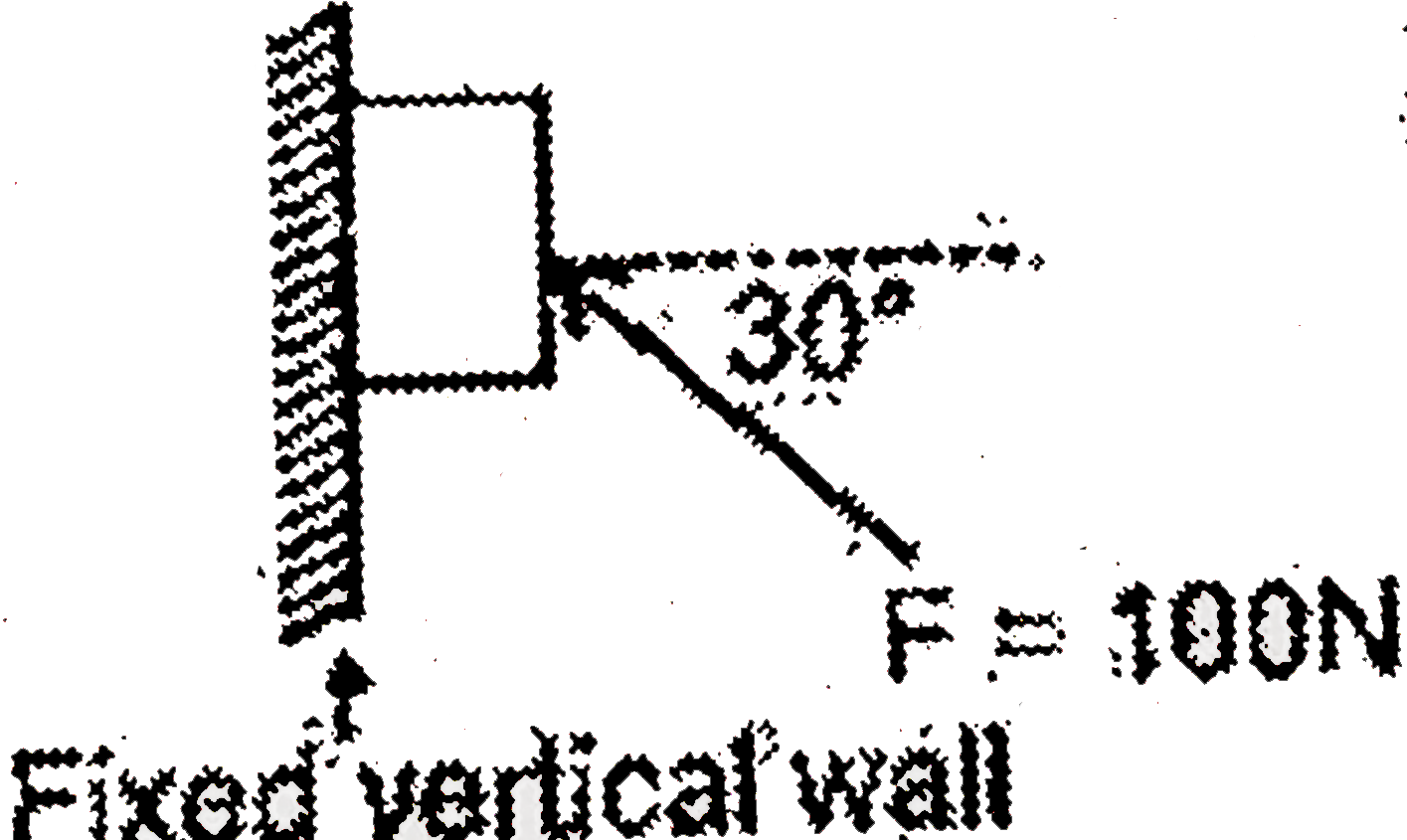
View SolutionA block A with mass 100kg is resting on another block B of mass 200kg. As shown in figure a horizontal rope tied to a wall hold it. The coefficient of friction between A and B is 0.2 while coefficient of friction between B and the ground is 0.3 . the minimum required force F to start moving B will be.

View SolutionA block A with mass 100kg is resting on another block B of mass 200kg. As shown in figure a horizontal rope tied to a wall hold it. The coefficient of friction between A and B is 0.2 while coefficient of friction between B and the ground is 0.3 . the minimum required force F to start moving B will be.

View Solution
