1 kg particle at a height of 4 m has a speed of 2 m/s down a incline making an angle 53∘ with horizontal as shown in figure. It slides on a horizontal section of length 3 m at ground level and then up an incline making an angle 37∘ with horizontal. All surfaces have μk=0.5. How far from point O (bottom of right inclined plane), along the incline making and angle 37∘ with horizontal, does the particle first come to rest?
To solve the problem, we will apply the work-energy theorem and analyze the motion of the particle step by step.
Step 1: Calculate the initial potential energy (PE) and kinetic energy (KE) of the particle at the height of 4 m.
The potential energy at height h is given by:
PE=mgh
where:
- m=1kg
- g=10m/s2 (approximating for simplicity)
- h=4m
Calculating:
PE=1×10×4=40J
The kinetic energy (KE) when the particle has a speed v is given by:
KE=12mv2
where v=2m/s.
Calculating:
KE=12×1×(2)2=12×1×4=2J
Step 2: Calculate the total mechanical energy at the starting point.
Total mechanical energy (TME) at the starting point is:
TME=PE+KE=40+2=42J
Step 3: Determine the work done against friction as the particle slides down the incline.
The distance along the incline can be calculated using the angle θ=53∘. The length of the incline d1 can be derived from the height:
d1=hsin(53∘)=4sin(53∘)≈40.8=5m
The work done against friction Wf while sliding down the incline is:
Wf=μk⋅m⋅g⋅d1⋅cos(53∘)
Calculating:
Wf=0.5⋅1⋅10⋅5⋅0.6=15J
Step 4: Calculate the total energy at the bottom of the incline.
At the bottom of the incline, the energy will be:
Ebottom=TME−Wf=42−15=27J
Step 5: Analyze the motion on the horizontal section.
On the horizontal section of length 3 m, the work done against friction is:
Wf=μk⋅m⋅g⋅d2
where d2=3m.
Calculating:
Wf=0.5⋅1⋅10⋅3=15J
The energy at the end of the horizontal section is:
Ehorizontal=Ebottom−Wf=27−15=12J
Step 6: Determine how far the particle travels up the incline at 37∘.
Let d3 be the distance traveled up the incline. The work done against gravity and friction while moving up the incline is:
W=μk⋅m⋅g⋅d3⋅cos(37∘)+mgh
where h=d3⋅sin(37∘).
Setting the energy at the incline equal to the work done:
12=μk⋅m⋅g⋅d3⋅cos(37∘)+m⋅g⋅d3⋅sin(37∘)
Substituting values:
12=0.5⋅1⋅10⋅d3⋅0.8+1⋅10⋅d3⋅0.6
12=4d3+6d3=10d3
Step 7: Solve for d3.
d3=1210=1.2m
Final Answer:
The particle first comes to rest at a distance of 1.2 m from point O along the incline making an angle of 37∘ with the horizontal.
---
PE=mgh
where:
- m=1kg
- g=10m/s2 (approximating for simplicity)
- h=4m
PE=1×10×4=40J
KE=12mv2
where v=2m/s.
KE=12×1×(2)2=12×1×4=2J
TME=PE+KE=40+2=42J
d1=hsin(53∘)=4sin(53∘)≈40.8=5m
Wf=μk⋅m⋅g⋅d1⋅cos(53∘)
Calculating:
Wf=0.5⋅1⋅10⋅5⋅0.6=15J
Ebottom=TME−Wf=42−15=27J
Wf=μk⋅m⋅g⋅d2
where d2=3m.
Wf=0.5⋅1⋅10⋅3=15J
Ehorizontal=Ebottom−Wf=27−15=12J
W=μk⋅m⋅g⋅d3⋅cos(37∘)+mgh
where h=d3⋅sin(37∘).
12=μk⋅m⋅g⋅d3⋅cos(37∘)+m⋅g⋅d3⋅sin(37∘)
12=0.5⋅1⋅10⋅d3⋅0.8+1⋅10⋅d3⋅0.6
12=4d3+6d3=10d3
The particle first comes to rest at a distance of 1.2 m from point O along the incline making an angle of 37∘ with the horizontal.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Single Correct Answer type491videos
CENGAGE PHYSICS DPP
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Answer Correct Type22videos
COULOMB LAW AND ELECTRIC FIELD
Similar Questions
A brick of mass 2kg begins to slide down on a plane inclined at an angle of 45∘ with the horizontal. The force of friction will be
View SolutionA person pushes a block of mass 4 kg up a frictionless inclined plane 10 m long and that makes an angle of 30∘ with the horizontal . Then the work done is
View Solution
Knowledge Check
- Question 1 - Select One
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.
A1/3 g sinθB2/3 g sinθC2/5 g sinθD2/7 g sinθ
An inclined plane makes an angle of 60∘ with horizontal. A disc rolling down this inclined plane without slipping has a linear acceleration equal to
View SolutionA block placed on an inclined plane making an angle 17∘ with the horizontal slides down without any acceleration. What is the acceleration of the block if the inclimation is increased to 30∘ ?
View SolutionAn inclined plane makes an angle 30° with the horizontal. A solid sphere rolling down this inclined plane from rest without slipping has a linear acceleration equal to
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
 View Solution
View SolutionA block of mass 2 kg rests on a rough inclined plane making an angle of 30° with the horizontal. If μS=0.6 , what is the frictional force on the block ?
View SolutionA ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
View Solution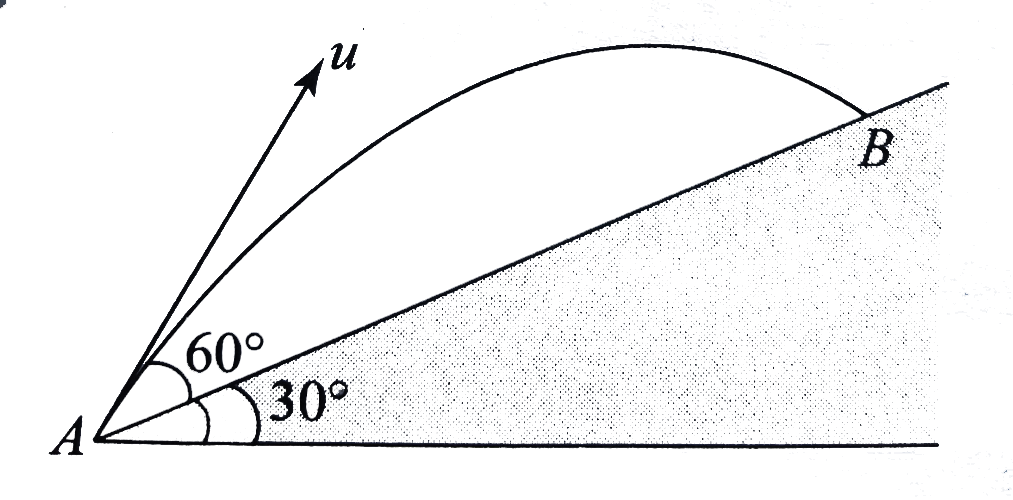
A stone is projected from point A with speed u making an angle 60∘ with horizontal as shown. The fixed inclined suface makes an angle 30∘ with horizontal. The stone lands at B after time t. Then the distance AB is equal toView Solution

