The resistance of a galvanometer is 90 ohm s. If only 10 percent of the main current may flow through the galvanometer, in which way and of what value, a resistor is to be used
10Ω in series
10Ω in parallel
810Ω in series
810Ω in parallel
The correct Answer is:B
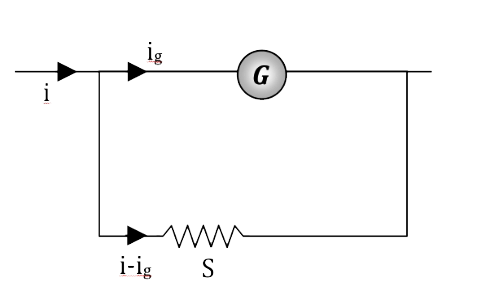
To solve the problem, we need to determine the value and configuration of a resistor (shunt resistor) that should be used with a galvanometer so that only 10% of the main current flows through it. Here’s a step-by-step solution:
Step 1: Understand the Configuration
Since we want only 10% of the main current to flow through the galvanometer, we need to connect a resistor (shunt resistor) in parallel with the galvanometer. In a parallel configuration, the voltage across both components is the same, but the current divides between them.
Hint: Remember that in parallel circuits, the voltage remains constant across all components.
Step 2: Define the Terms
Let:
- I = total (main) current flowing through the circuit.
- Ig = current through the galvanometer = 10% of I = 10100I=0.1I.
- Is = current through the shunt resistor S = 90% of I = 90100I=0.9I.
Hint: Identify the relationship between the currents in the parallel circuit.
Step 3: Apply Ohm’s Law
According to Ohm's Law, the voltage across the galvanometer (V) can be expressed as:
V=Ig×Rg
Where Rg is the resistance of the galvanometer (90 ohms).
Thus,
V=(0.1I)×90=9I
Hint: Use Ohm's Law to relate voltage, current, and resistance.
Step 4: Write the Voltage Equation for the Shunt Resistor
The voltage across the shunt resistor S is also equal to the voltage across the galvanometer:
V=Is×S
Substituting Is=0.9I:
V=(0.9I)×S
Hint: Set the voltage expressions equal since they are in parallel.
Step 5: Equate the Two Voltage Expressions
Now we can set the two voltage expressions equal to each other:
9I=(0.9I)×S
Step 6: Simplify the Equation
Dividing both sides by I (assuming I≠0):
9=0.9S
Step 7: Solve for the Shunt Resistance S
Now, solve for S:
S=90.9=10ohms
Hint: Isolate the variable to find the value of the shunt resistor.
Step 8: Conclusion
The value of the shunt resistor S is 10 ohms, and it should be connected in parallel with the galvanometer.
Final Answer: A 10-ohm resistor should be connected in parallel with the galvanometer.
Since we want only 10% of the main current to flow through the galvanometer, we need to connect a resistor (shunt resistor) in parallel with the galvanometer. In a parallel configuration, the voltage across both components is the same, but the current divides between them.
Let:
- I = total (main) current flowing through the circuit.
- Ig = current through the galvanometer = 10% of I = 10100I=0.1I.
- Is = current through the shunt resistor S = 90% of I = 90100I=0.9I.
According to Ohm's Law, the voltage across the galvanometer (V) can be expressed as:
V=Ig×Rg
Where Rg is the resistance of the galvanometer (90 ohms).
V=(0.1I)×90=9I
The voltage across the shunt resistor S is also equal to the voltage across the galvanometer:
V=Is×S
Substituting Is=0.9I:
V=(0.9I)×S
Now we can set the two voltage expressions equal to each other:
9I=(0.9I)×S
Dividing both sides by I (assuming I≠0):
9=0.9S
Now, solve for S:
S=90.9=10ohms
The value of the shunt resistor S is 10 ohms, and it should be connected in parallel with the galvanometer.
Similar Questions
A galvanometer of resistance 150 ohm is shunted such that only 1/11 of the main current flows through the galvanometer. The resistance of the shunt is, (in ohm)
View SolutionIf 2% of the main current is to be passed through the galvanometer of resistance G, the resistance of shunt required is
View Solution
Knowledge Check
If the galvanometer current is 10 mA, resistance of the galvanometer is 40Ω and shunt of 2Ω is connected to the galvanometer, the maximum current which can be measured by this ammeter is
A0.21AB2.1AC210AD21A
A galvanometer of resistance 20Ω is shunted by a 2Ω resistor. What part of the main current flows through the galvanometer ?
View SolutionA galvanometer has a resistance of 98Ω. If 2% of the main current is to be passed through the meter what should be the value of the shunt?
View SolutionA moving coil galvanometer has a resistance of 990Ω. in order to send only 10% of the main currect through this galvanometer, the resistance of the required shunt is
View SolutionWe have a galvanometer of resistance 25Ω. It is shunted by a 2.5Ω wire. The part of total current that flows through the galvanometer is given as
View SolutionThe resistance of galvanometer is 999Ω. A shunt of 1Ω is connected to it. If the main current is 10−2A, what is the current flowing through the galvanometer.
View SolutionIn a ammeter 0.2% of main current passes through the galvanometer. If resistance of galvanometer is G, the resistance of ammeter will be
View SolutionTo send 10% of the main current through a moving coil galvanometer of resistance 9 Ω, shunt S required is
View Solution
