In meter brigde of Wheatstone bridge for measurment of resistance, the known and the unknown resistance are interchanged. The error so removed is
end correction
index error
due to temperature effect
random error
The correct Answer is:A
To solve the problem regarding the error removed when the known and unknown resistances are interchanged in a meter bridge (Wheatstone bridge), we can follow these steps:
1. Understanding the Meter Bridge:
A meter bridge is a device used to measure unknown resistances by balancing two legs of a bridge circuit. It consists of a wire of uniform cross-section and known length (1 meter) and a galvanometer to detect the null point.
2. Identifying the Error:
In a typical meter bridge setup, it is assumed that the resistance of the connecting wires and the bridge itself is negligible. However, this is not entirely true, and there exists a small resistance in the bridge wire, which leads to an error in the measurement. This error is known as "end error."
3. Interchanging Known and Unknown Resistances:
When the known resistance (R) and the unknown resistance (X) are interchanged, the balance point shifts. By taking readings with both configurations (R in place of X and vice versa), we can average the readings to minimize the effect of the end error.
4. Calculating the Mean Reading:
When the resistances are interchanged, we can denote the balance lengths as L1 (for known resistance) and L2 (for unknown resistance). The mean reading can be calculated as:
Lmean=L1+L22
This mean value helps in compensating for the end error.
5. Conclusion:
By interchanging the known and unknown resistances and taking the mean of the readings, the end error is effectively removed, leading to a more accurate measurement of the unknown resistance.
Final Answer:
The error that is removed by interchanging the known and unknown resistances in a meter bridge is the "end error."
---
A meter bridge is a device used to measure unknown resistances by balancing two legs of a bridge circuit. It consists of a wire of uniform cross-section and known length (1 meter) and a galvanometer to detect the null point.
In a typical meter bridge setup, it is assumed that the resistance of the connecting wires and the bridge itself is negligible. However, this is not entirely true, and there exists a small resistance in the bridge wire, which leads to an error in the measurement. This error is known as "end error."
When the known resistance (R) and the unknown resistance (X) are interchanged, the balance point shifts. By taking readings with both configurations (R in place of X and vice versa), we can average the readings to minimize the effect of the end error.
When the resistances are interchanged, we can denote the balance lengths as L1 (for known resistance) and L2 (for unknown resistance). The mean reading can be calculated as:
Lmean=L1+L22
This mean value helps in compensating for the end error.
By interchanging the known and unknown resistances and taking the mean of the readings, the end error is effectively removed, leading to a more accurate measurement of the unknown resistance.
The error that is removed by interchanging the known and unknown resistances in a meter bridge is the "end error."
Similar Questions
Statement-1 : In a Meter Bridge experiment, null point for an unknown resistance is measured. Now, the unknown resistance is put inside an enclosure maintained at a higher temperature. The null point can be obtained at the same point as before by decreasing the value of the standard resistance.
Statement-2 : Resistance of metal increases with increase in temperature.
View SolutionStatement-1 : In a Meter Bridge experiment, null point for an unknown resistance is measured. Now, the unknown resistance is put inside an enclosure maintained at a higher temperature. The null point can be obtained at the same point as before by decreasing the value of the standard resistance.
Statement-2 : Resistance of metal increases with increase in temperature.
View Solution
Knowledge Check
Four resistances of 3Ω,3Ω,3Ωand4Ω respectively are used to form a Wheatstone bridge. The 4Ω resistance is short circuited with a resistance R in order to get bridge balanced. The value of R will be
A10ΩB11ΩC12ΩD13Ω
In the circuit shown, a meter bridge is in its balanced state. The meter bridge wire has a resistance .1ohm/cm. The value of unknown resistance X and the current drawn from the battery of negligible resistance is
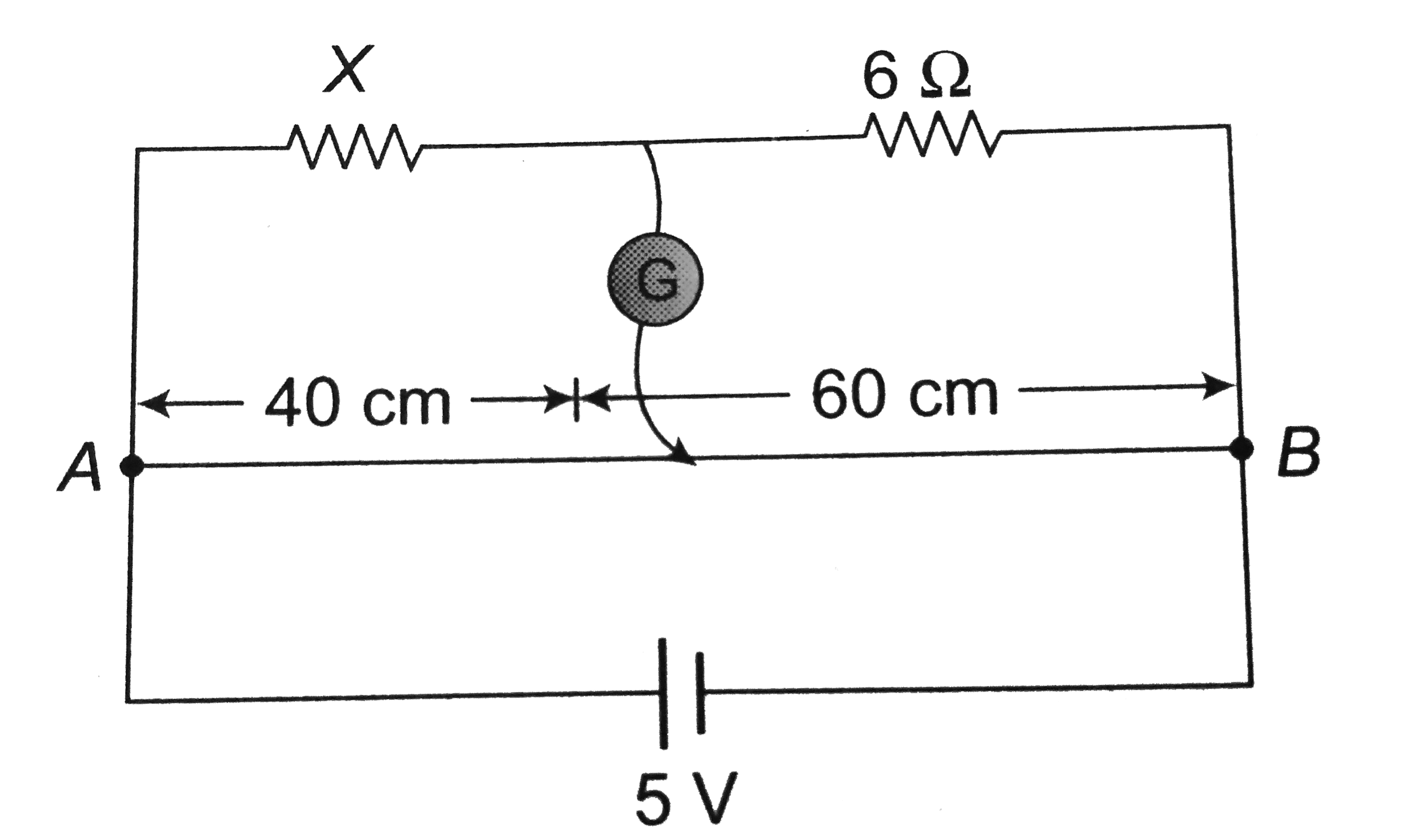
View SolutionIn the circuit shown, a meter bridge is in its balanced state. The meter bridge wire has a resistance .1ohm/cm. The value of unknown resistance X and the current drawn from the battery of negligible resistance is

View SolutionIf in the experiment of Wheatstone's bridge, the positions of cells and galvanometer are interchanged, then balance point will
View SolutionResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged he balance point shifts by
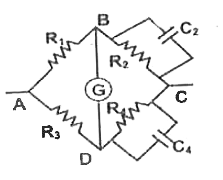
View SolutionConsider a Wheatstone bridge with resistance and capacitance connected as shown Find the condition on the resistance and the capacitance such that the bridge remains balanced at all times.
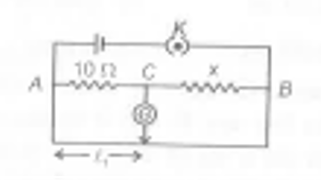
View SolutionThe resistance in two arms of the meter bridge is 10Ω and x respectively. The balance length is l1from left. Now the resistance of 10Ω is shunted with another resistor of resistance equal to unknown resistance x then new balance point is 0.75l1 The value of resistance x is
View SolutionIn a metre bridge when the resistance in the left gap is 2Ω and an unknown resistance in the right gap, the balance point is obtained at 40 cm from the zero end. On shunting the unknown resistance with 2Ω find the shift of the balance point on the bridge wire.
View Solution
