Two identical plates of different metals are joined to form a single plate whose thickness is double the thickness of each plate. If the coefficients of conductivity of each plate are 2 and 3 respectively, Then the conductivity of composite plate will be
The correct Answer is:2.4
To find the conductivity of the composite plate formed by two identical plates of different metals, we can follow these steps:
Step 1: Understand the Setup
We have two identical plates made of different metals, with thermal conductivities k1=2 and k2=3. The thickness of each plate is l, so the total thickness of the composite plate is 2l.
Step 2: Apply the Concept of Thermal Conductivity
The thermal current I through a material can be expressed using Fourier's law of heat conduction:
I=k⋅A⋅(Th−Tc)L
where:
- I is the thermal current,
- k is the thermal conductivity,
- A is the cross-sectional area,
- Th and Tc are the temperatures on either side of the plate,
- L is the thickness of the plate.
Step 3: Calculate Thermal Current for Each Plate
For the first plate (with conductivity k1=2):
I1=2⋅A⋅(Th−Tc)l
For the second plate (with conductivity k2=3):
I2=3⋅A⋅(Th−Tc)l
Step 4: Total Thermal Current
Since the plates are in series, the total thermal current I through the composite plate is the sum of the currents through each plate:
I=I1+I2=2⋅A⋅(Th−Tc)l+3⋅A⋅(Th−Tc)l
I=(2+3)⋅A⋅(Th−Tc)l=5⋅A⋅(Th−Tc)l
Step 5: Equivalent Conductivity of the Composite Plate
For the composite plate, which has an area of 2A and thickness 2l, we can express the thermal current as:
I=keq⋅2A⋅(Th−Tc)2l
Step 6: Set the Two Expressions for Thermal Current Equal
Equating the two expressions for I:
5⋅A⋅(Th−Tc)l=keq⋅2A⋅(Th−Tc)2l
Step 7: Simplify and Solve for keq
Cancelling A and (Th−Tc) from both sides (assuming they are not zero):
5=keq⋅22
5=keq
Final Result
Thus, the equivalent conductivity keq of the composite plate is:
keq=5
We have two identical plates made of different metals, with thermal conductivities k1=2 and k2=3. The thickness of each plate is l, so the total thickness of the composite plate is 2l.
The thermal current I through a material can be expressed using Fourier's law of heat conduction:
I=k⋅A⋅(Th−Tc)L
where:
- I is the thermal current,
- k is the thermal conductivity,
- A is the cross-sectional area,
- Th and Tc are the temperatures on either side of the plate,
- L is the thickness of the plate.
For the first plate (with conductivity k1=2):
I1=2⋅A⋅(Th−Tc)l
I2=3⋅A⋅(Th−Tc)l
Since the plates are in series, the total thermal current I through the composite plate is the sum of the currents through each plate:
I=I1+I2=2⋅A⋅(Th−Tc)l+3⋅A⋅(Th−Tc)l
I=(2+3)⋅A⋅(Th−Tc)l=5⋅A⋅(Th−Tc)l
For the composite plate, which has an area of 2A and thickness 2l, we can express the thermal current as:
I=keq⋅2A⋅(Th−Tc)2l
Equating the two expressions for I:
5⋅A⋅(Th−Tc)l=keq⋅2A⋅(Th−Tc)2l
Cancelling A and (Th−Tc) from both sides (assuming they are not zero):
5=keq⋅22
5=keq
Thus, the equivalent conductivity keq of the composite plate is:
keq=5
Topper's Solved these Questions
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single Corrct Answer
7 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Multiple Correct Answer
2 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Comprehension type
4 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
Similar Questions
Two identical plates with thermal conductivities K and 3K are joined together to form a single plate of double thickness. The equivalent thermal conductivity of one composite plate so formed for the flow of heat through its thickness is.
View SolutionThe length of rivet is slightly smaller than the thickness of metal plates.
View Solution
Knowledge Check
IF two metallic plates of equal thickness and thermal conductivities K1andK2 are put together face to face anda common plate is constructed, then the equivalent thermal conductivity of this plate will be
AK1K2K1+K2B2K1K2K1+K2C(K21_K22)3/2K1K2D(K21+K22)3/22K1K2
Five identical plates each of area a are joined as shown in the figure. The distance between the plates is d. the plates are connected to a potential difference of V volt. The charge on plates 1 and 4 will be

View SolutionTwo plates of equal area are placed in contact with each other. The thickness of the plates are 2.0 cm and 3.0 cm respectively. The outer face of first plate is at −25∘C and that of second plate is at +25∘C. The conductivities of the plates are in the ratio 2:3. Calculate the temperature of the common surface of the plates.
View SolutionTwo parallel plates A and B are joined together to form a compound plate . The thicknesses of the plate are 4.0cm and 2.5cm respectively and the area of cross section is 100cm2 for each plate. The thermal conductivities are KA=200Wm−1C−1 for plate A and KB=400Wm−1C−1 for the plate B. The outer surface of the plate A is maintained at100∘C and the outer surface of the plate B is maintained at 0∘C. Find (a) the rate of heat flow through any cross section, (b) the temperature at the interface and (c) the equivalent thermal conductivity of the compound plate.

View SolutionFind the charge on each plate given area of each plate is A and separation between two consecutive plates is d.

View SolutionA double equatorial plate is formed in
View SolutionTwo plates eachb of area A, thickness L1andL2 thermal conductivities K1andK2 respectively are joined to form a single plate of thickness (L1+L2). If the temperatures of the free surfaces are T1andT2. Calculate.
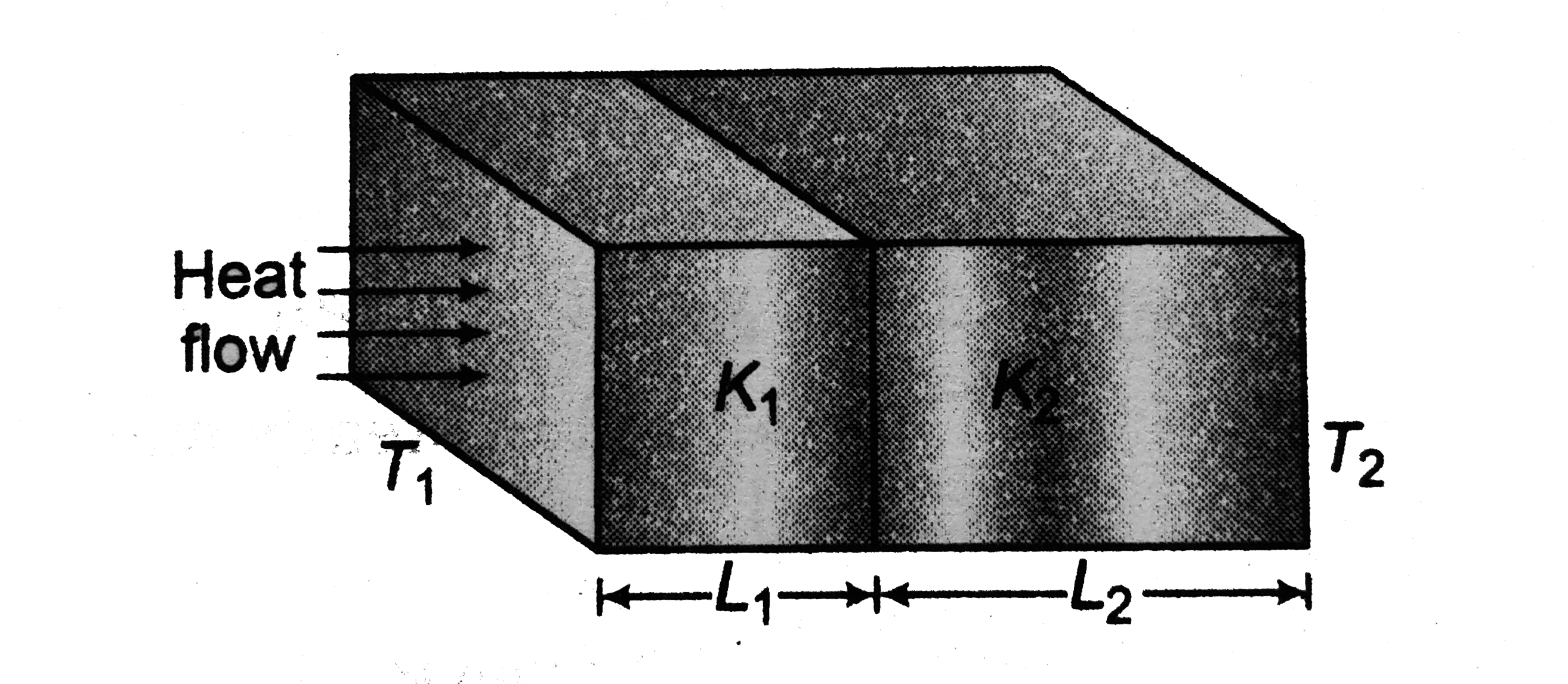
(a) rate of flow of heat
(b) temperature of interface and
(c) equivalent thermal conductivity.
View SolutionA composite slab is prepared by pasting two plates of thickness L1 and L2 and thermal conductivities K1 and K2 . The slab have equal cross-sectional area. Find the equivalent conductivity of the composite slab.
View Solution
