A technician wraps wire around a tube of length 4π2 cm having a diameter of 8.00cm. When the windings are evenly spread over the full length of the tube, the result is a solenoid containing 1000turns of wire. If the current in this solonoid increases at the rate of 4.00A/s
the self-induced emf in the solenoid is
32mV
16mV
64mV
24mV
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Calculate the area of the cross-section of the solenoid.
The diameter of the tube is given as 8.00 cm. To find the radius, we divide the diameter by 2:
Radius(r)=Diameter2=8.00 cm2=4.00 cm=0.04 m
Now, we can calculate the area A of the cross-section using the formula:
A=πr2=π(0.04)2=π(0.0016)≈0.0050265 m2
Step 2: Calculate the inductance L of the solenoid.
The formula for the inductance of a solenoid is given by:
L=μ0n2Al
where:
- μ0=4π×10−7H/m (permeability of free space),
- n=1000 (number of turns),
- A is the area calculated in Step 1,
- l=4π2cm=0.04π2m.
Substituting the values:
L=(4π×10−7)(1000)2(0.0050265)0.04π2
Calculating the values:
L=(4π×10−7)1000000×0.00502650.04π2
L=(4×10−7)5026.50.04π
L=(4×10−7)5026.50.12566≈(4×10−7)×40000≈16×10−3 H=0.016 H
Step 3: Calculate the self-induced emf E.
The self-induced emf can be calculated using the formula:
E=Ldidt
Given that didt=4.00A/s:
E=0.016×4=0.064 V=64 mV
Final Answer:
The self-induced emf in the solenoid is 64mV.
---
The diameter of the tube is given as 8.00 cm. To find the radius, we divide the diameter by 2:
Radius(r)=Diameter2=8.00 cm2=4.00 cm=0.04 m
Now, we can calculate the area A of the cross-section using the formula:
A=πr2=π(0.04)2=π(0.0016)≈0.0050265 m2
The formula for the inductance of a solenoid is given by:
L=μ0n2Al
where:
- μ0=4π×10−7H/m (permeability of free space),
- n=1000 (number of turns),
- A is the area calculated in Step 1,
- l=4π2cm=0.04π2m.
L=(4π×10−7)(1000)2(0.0050265)0.04π2
Calculating the values:
L=(4π×10−7)1000000×0.00502650.04π2
L=(4×10−7)5026.50.04π
L=(4×10−7)5026.50.12566≈(4×10−7)×40000≈16×10−3 H=0.016 H
The self-induced emf can be calculated using the formula:
E=Ldidt
Given that didt=4.00A/s:
E=0.016×4=0.064 V=64 mV
The self-induced emf in the solenoid is 64mV.
Topper's Solved these Questions
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Multiple Correct Answer
3 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
Similar Questions
A technician wraps wire around a tube of length 4π2 cm having a diameter of 8.00cm. When the windings are evenly spread over the full length of the tube, the result is a solenoid containing 1000turns of wire. If the current in this solonoid increases at the rate of 4.00A/s.
the inductance of this solenoid is
View SolutionThe current in a solenoid of 240 turns, having a length of 12 cm and a radius of 2 cm, changes at a rate of 0.8As−. Find the emf induced in it.
View SolutionA solenoid has length 0.4m, radius 1 cm and 400 turns of wire. If a current fo 5 A is passed through this solenoid, then what is the magnetic field inside the solenoid?
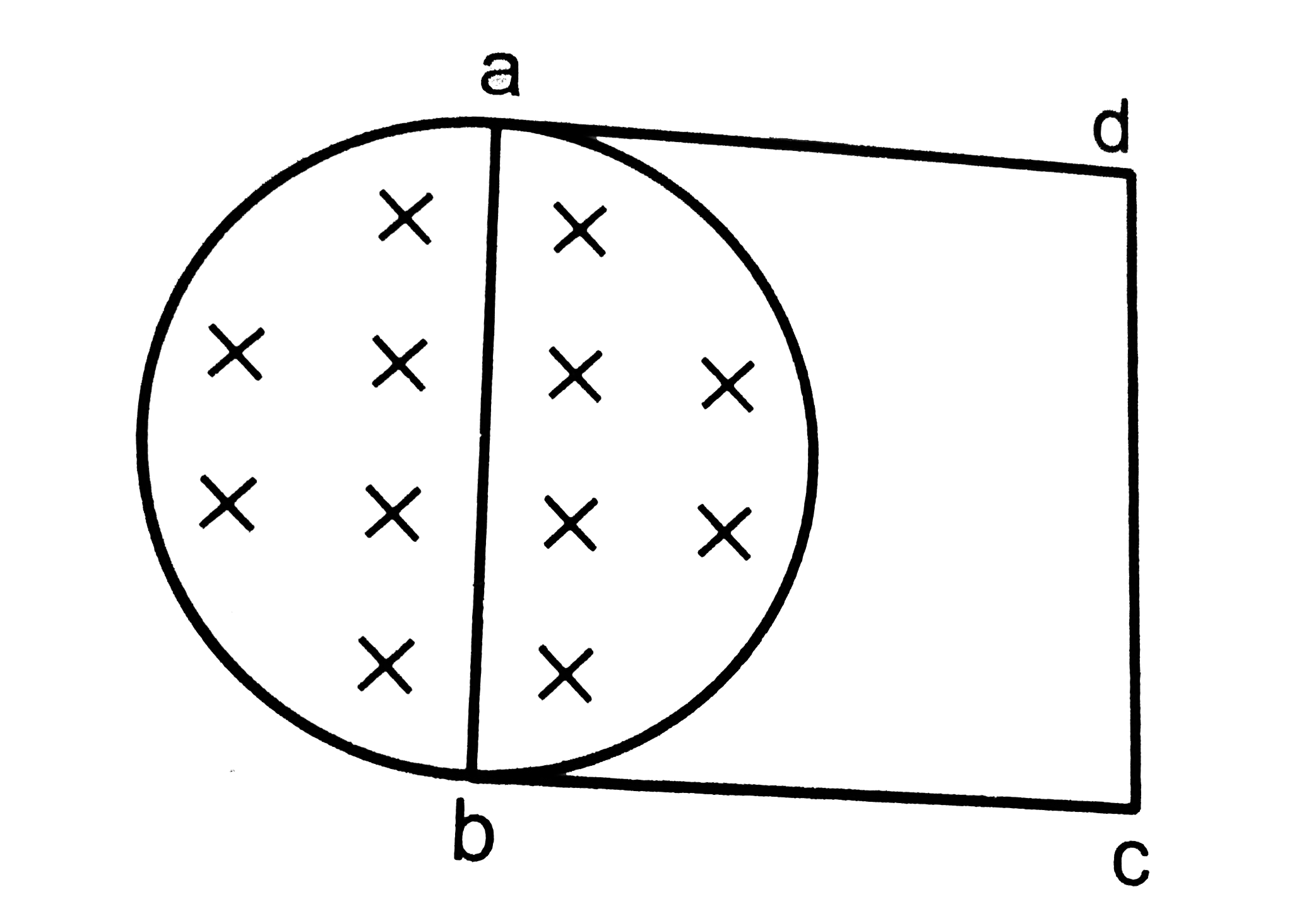
View SolutionA unifrom magnetic field B exists in a cylindrical region of radius 10 cm as shown in. A uniform wire of length 80 cm and resistance 4.0 Omega is bent into a square frame and is placed with one side along a diameter of the cylindrical region. If the magnetic field increases at a constant rate of 0.010 T/s, find the current induced in the frame.
View Solutiona. Calculate the inductance of an air core solenoid containing 300 turns if the length of the solenoid is 25.0cm and its cross -sectional area is 4.00cm2.
b. Calculate the self -induced emf in the solenoid if the current through it is decreasing at the rate of 50.0A/s.
View Solutiona. Calculate the inductance of an air core solenoid containing 300 turns if the length of the solenoid is 25.0cm and its cross -sectional area is 4.00cm2.
b. Calculate the self -induced emf in the solenoid if the current through it is decreasingg at the rate of 50.0A/s.
View SolutionA current l = 1.5 A is flowing through a long solenoid of diameter 3.2 cm, having 220 turns per cm. At its centre, a 130 turn closely packed coil of diameter 2.1 cm is placed such that the coil is coaxial with the long solenoid. The current in the solenoid is reduced to zero at a steady rate in 25 ms. What is the magnitude of emf induced in the coil while the current in the solenoid is changing?
View SolutionA long solenoid of cross-sectional area 5.0cm2 is wound with 25 turns of wire per centimetre. It is placed in the middle of a closely wrapped coil of 10 turns and radius 25 cm as shown.
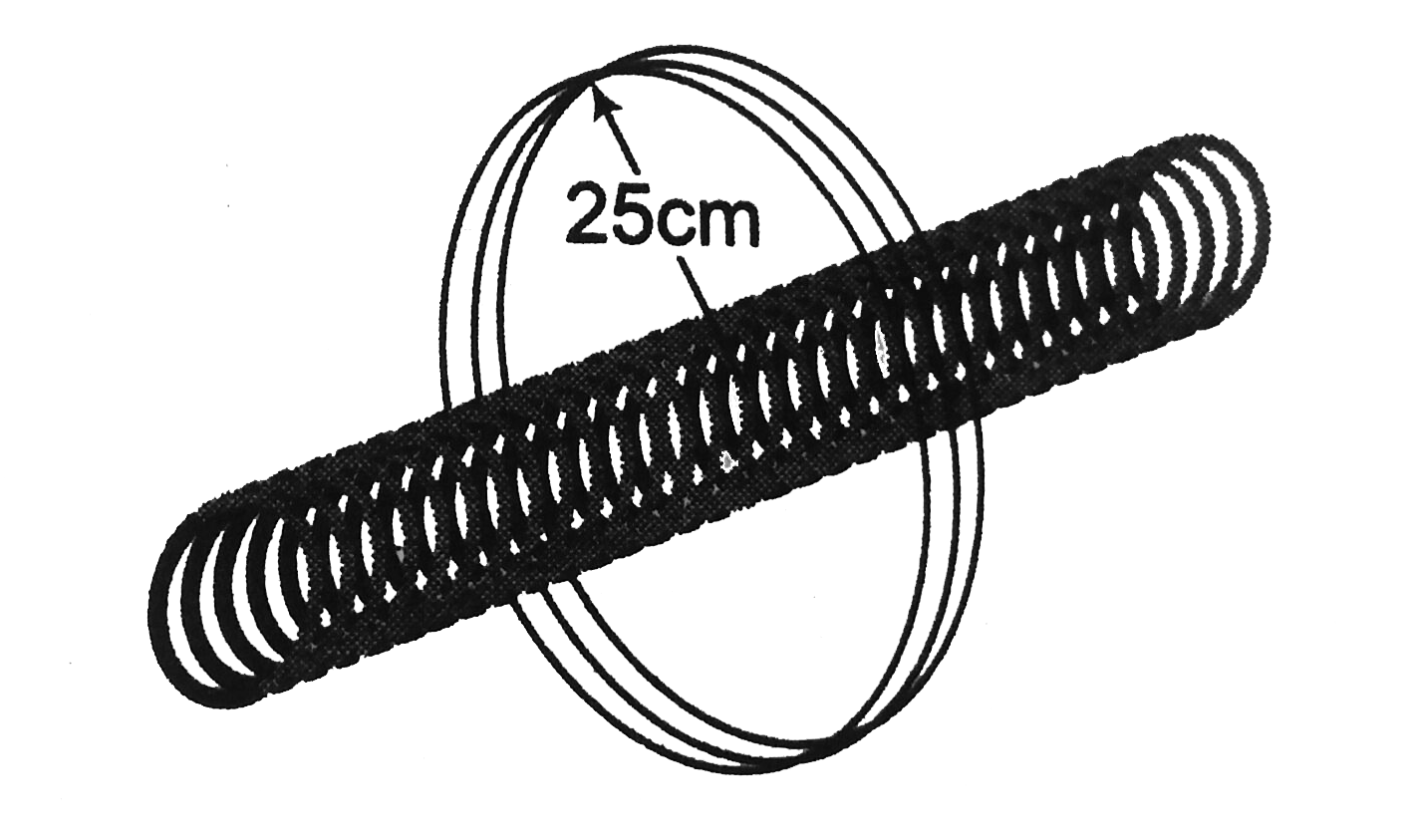
(a) What is the emf induced in the coil when the current through the solenoid is decreasing at a rate −0.20A/s ?
(b) What is the electric field induced in the coil?
View SolutionA long solenoid having 200 turns per centimeter carries a current of 1.5A. At the center of the solenoid is placed a coil of 100 turns of cross-sectional area 3.14×10−4m2 having its axis parallel to the field produced by the solenoid. When the direction of current in teh solenoid is reversed within 0.05s, the induced emf in the coil is
View SolutionFor a toroid N = 500, radius = 40 cm, and area of cross section =10cm2. Find inductance
View Solution
