The current in a solenoid of 240 turns, having a length of 12 cm and a radius of 2 cm, changes at a rate of 0.8As−. Find the emf induced in it.
To find the induced EMF in the solenoid, we will follow these steps:
Step 1: Identify the formula for induced EMF
The induced EMF (ε) in an inductor (or solenoid) is given by the formula:
ϵ=Ldidt
where:
- L is the inductance of the solenoid,
- didt is the rate of change of current.
Step 2: Calculate the inductance L of the solenoid
The inductance L of a solenoid is given by the formula:
L=μ0N2Al
where:
- μ0=4π×10−7T m/A (permeability of free space),
- N is the number of turns (240 turns),
- A is the cross-sectional area of the solenoid,
- l is the length of the solenoid.
Step 3: Calculate the cross-sectional area A
The cross-sectional area A of the solenoid is given by:
A=πr2
where r is the radius of the solenoid. Given that the radius is 2 cm, we convert it to meters:
r=2cm=0.02m
Now, calculate A:
A=π(0.02)2=π(0.0004)≈1.25664×10−3m2
Step 4: Convert the length of the solenoid to meters
The length of the solenoid is given as 12 cm, which we convert to meters:
l=12cm=0.12m
Step 5: Substitute values into the inductance formula
Now we can substitute the values into the inductance formula:
L=(4π×10−7)(2402)(1.25664×10−3)0.12
Calculating 2402=57600:
L=(4π×10−7)(57600)(1.25664×10−3)0.12
Calculating this step-by-step:
1. Calculate 4π≈12.5664,
2. Then 12.5664×10−7×57600≈7.238×10−2,
3. Now multiply by 1.25664×10−3≈9.095×10−5,
4. Finally, divide by 0.12:
L≈9.095×10−50.12≈7.54×10−4H
Step 6: Calculate the induced EMF
Now we can substitute L and didt into the EMF formula:
Given didt=0.8A/s:
ϵ=Ldidt=(7.54×10−4)(0.8)
Calculating this gives:
ϵ≈6.032×10−4V≈6.14×10−4V
Final Answer
The induced EMF in the solenoid is approximately:
ϵ≈6.14×10−4V
---
The induced EMF (ε) in an inductor (or solenoid) is given by the formula:
ϵ=Ldidt
where:
- L is the inductance of the solenoid,
- didt is the rate of change of current.
The inductance L of a solenoid is given by the formula:
L=μ0N2Al
where:
- μ0=4π×10−7T m/A (permeability of free space),
- N is the number of turns (240 turns),
- A is the cross-sectional area of the solenoid,
- l is the length of the solenoid.
The cross-sectional area A of the solenoid is given by:
A=πr2
where r is the radius of the solenoid. Given that the radius is 2 cm, we convert it to meters:
r=2cm=0.02m
Now, calculate A:
A=π(0.02)2=π(0.0004)≈1.25664×10−3m2
The length of the solenoid is given as 12 cm, which we convert to meters:
l=12cm=0.12m
Now we can substitute the values into the inductance formula:
L=(4π×10−7)(2402)(1.25664×10−3)0.12
Calculating 2402=57600:
L=(4π×10−7)(57600)(1.25664×10−3)0.12
Calculating this step-by-step:
1. Calculate 4π≈12.5664,
2. Then 12.5664×10−7×57600≈7.238×10−2,
3. Now multiply by 1.25664×10−3≈9.095×10−5,
4. Finally, divide by 0.12:
L≈9.095×10−50.12≈7.54×10−4H
Now we can substitute L and didt into the EMF formula:
Given didt=0.8A/s:
ϵ=Ldidt=(7.54×10−4)(0.8)
Calculating this gives:
ϵ≈6.032×10−4V≈6.14×10−4V
The induced EMF in the solenoid is approximately:
ϵ≈6.14×10−4V
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer9videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 210videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 27videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise8videos
ELECTROMAGNETIC WAVES
Similar Questions
A technician wraps wire around a tube of length 4π2 cm having a diameter of 8.00cm. When the windings are evenly spread over the full length of the tube, the result is a solenoid containing 1000turns of wire. If the current in this solonoid increases at the rate of 4.00A/s
the self-induced emf in the solenoid isView SolutionA solenoid of length 20cm, area of cross- section 4.0cm2 and having 4000 turns is placed inside another solenoid of 2000 turns having a cross - sectional area 8.0cm2 and length 10cm. Find the mutual inductance between the solenoids.
View Solution
Knowledge Check
- Question 1 - Select One
Find the ara of a rectangle of length 12 cm and breadth 8.5cm.
A109 sq. cmB102 sq. cmC106 sq. cmD108 sq. cm
In Fig (a) and (b), two air-cored solenoids P and Q have been shows. They are placed near each other. In Fig (a), when IP, the current in P, changes at the rate of 5As−1, an emf of 2mV is induced in Q. The current in P is then switched off, and the current changing at 2As−1 is fed through Q as shows in the figure. What emf will be induced in P?

 View Solution
View Solutiona. Calculate the inductance of an air core solenoid containing 300 turns if the length of the solenoid is 25.0cm and its cross -sectional area is 4.00cm2.
b. Calculate the self -induced emf in the solenoid if the current through it is decreasing at the rate of 50.0A/s.View Solutiona. Calculate the inductance of an air core solenoid containing 300 turns if the length of the solenoid is 25.0cm and its cross -sectional area is 4.00cm2.
b. Calculate the self -induced emf in the solenoid if the current through it is decreasingg at the rate of 50.0A/s.View SolutionThe current in a long solenoid of radius R and having n turns per unit length is given by i=i0sinωt. A coil having N turns is wound around it near the centre. Find (a) the induced emf in the coil and (b) the mutual inductance between the solenoid and the coil.
View SolutionA technician wraps wire around a tube of length 4π2 cm having a diameter of 8.00cm. When the windings are evenly spread over the full length of the tube, the result is a solenoid containing 1000turns of wire. If the current in this solonoid increases at the rate of 4.00A/s.
the inductance of this solenoid isView SolutionA long solenoid of cross-sectional area 5.0cm2 is wound with 25 turns of wire per centimetre. It is placed in the middle of a closely wrapped coil of 10 turns and radius 25 cm as shown.
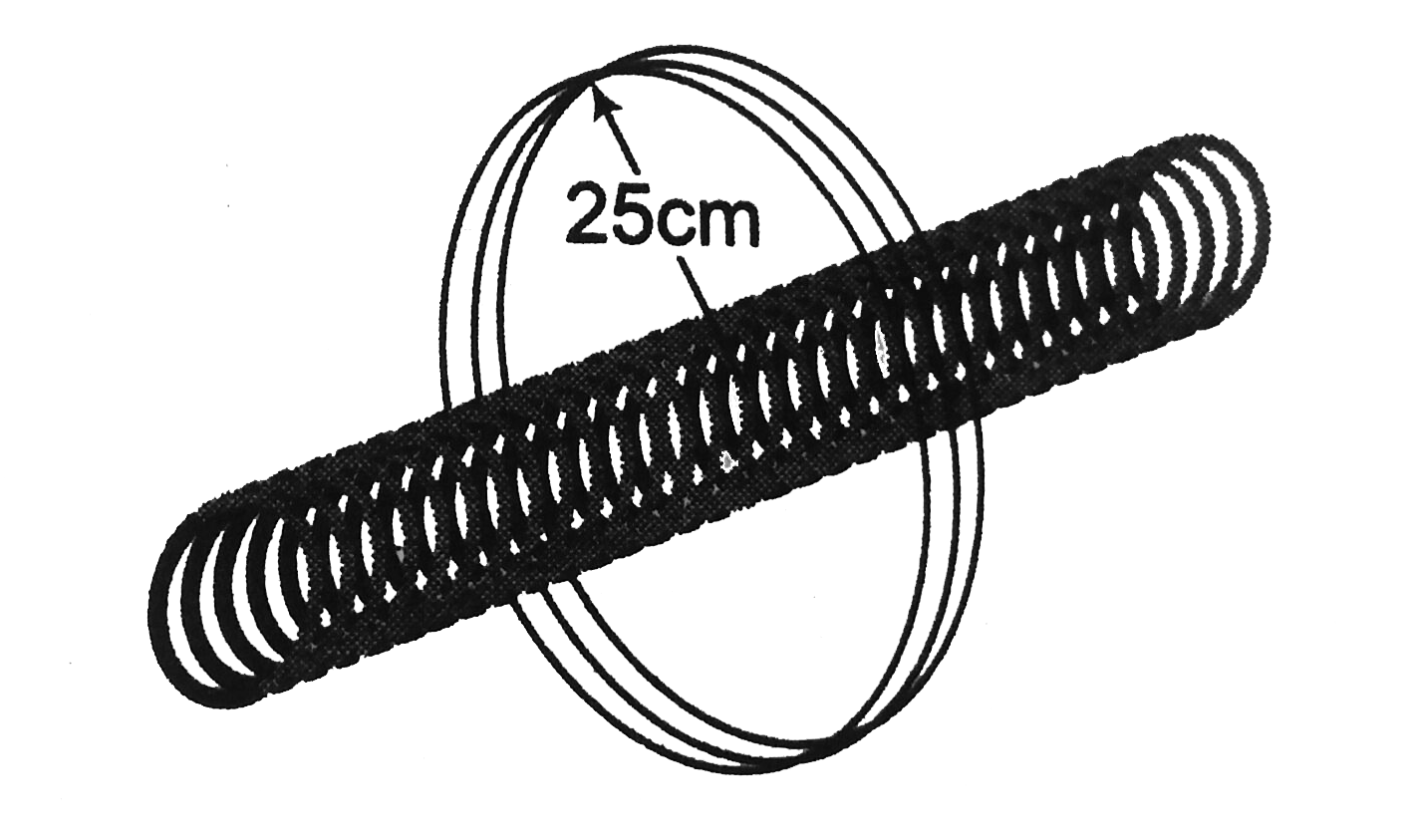
(a) What is the emf induced in the coil when the current through the solenoid is decreasing at a rate −0.20A/s ?
(b) What is the electric field induced in the coil?View SolutionA solenoid of length 10 cm and radius 1 cm contains 200 turns and carries a current of 10 A. Find the magnetic field at a point on the axis at a distance of 10cm from the centre.
View Solution

