A thin mica sheet of thickness 2×10−6m and refractive index (μ=1.5) is introduced in the path of the first wave. The wavelength of the wave used is 5000Å. The central bright maximum will shift
2 fringes upward
2 fringes downward
10 fringes upwards
none of these
The correct Answer is:A
To determine the shift in the central bright maximum when a thin mica sheet is introduced, we can follow these steps:
Step 1: Identify the given values
- Thickness of the mica sheet (t) = 2×10−6m
- Refractive index of mica (μ) = 1.5
- Wavelength of the wave (λ) = 5000 Å = 5000×10−10m
Step 2: Calculate the extra path difference
The extra path difference (Δx) introduced by the mica sheet can be calculated using the formula:
Δx=(μ−1)⋅t
Substituting the values:
Δx=(1.5−1)⋅(2×10−6)=0.5⋅(2×10−6)=1×10−6m
Step 3: Calculate the number of fringes shifted
The number of fringes (n) shifted can be calculated using the formula:
n=Δxλ
Substituting the values:
n=1×10−65000×10−10=1×10−65×10−7=2
Step 4: Interpret the result
Since n=2, this means that the central bright maximum will shift by 2 fringes upwards.
Final Answer
The central bright maximum will shift by 2 fringes upwards.
---
- Thickness of the mica sheet (t) = 2×10−6m
- Refractive index of mica (μ) = 1.5
- Wavelength of the wave (λ) = 5000 Å = 5000×10−10m
The extra path difference (Δx) introduced by the mica sheet can be calculated using the formula:
Δx=(μ−1)⋅t
Substituting the values:
Δx=(1.5−1)⋅(2×10−6)=0.5⋅(2×10−6)=1×10−6m
The number of fringes (n) shifted can be calculated using the formula:
n=Δxλ
Substituting the values:
n=1×10−65000×10−10=1×10−65×10−7=2
Since n=2, this means that the central bright maximum will shift by 2 fringes upwards.
The central bright maximum will shift by 2 fringes upwards.
Similar Questions
A thin mica sheet of thickness 4×10−6 m and refractive index (μ=1.5) is introduced in the path of the light from upper slit. The wavelength of the wave used is 5000Å The central bright maximum will shift
View SolutionA double slit experiment is performed with light of wavelength 500nm. A thin film of thickness 2μm and refractive index 1.5 is introduced in the path of the upper beam. The location of the central maximum will
View SolutionIf a thin mica sheet of thickness 't' and refractive index μ is placed in the path of one of the waves producing interference , then the whole interference pattern shifts towards the side of the sheet by a distance
View SolutionInterference fringes were produced using light in a doulbe-slit experiment. When a mica sheet of uniform thickness and refractive index 1.6 (relative to air) is placed in the path of light from one of the slits, the central fringe moves through some distance. This distance is equal to the width of 30 interference bands if light of wavelength 4800 is used. The thickness (in μm) of mica is
View SolutionIn YDSE using monochromatic light, the fringe pattern shifts by a certain distance on the screen when a mica sheet of refractive index 1.5 and thickness 2 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the distance between the plane of slits and the screen is doubled. It is found that the distance between successive maxima (or minima) now is the same as the observed fringe shift upon the introduction of the mica sheet. Calculate the wavelength of the light.
View SolutionTo make the central fringe at the centerO , mica sheet of refractive index 1.5 is introduced Choose the corect statement.
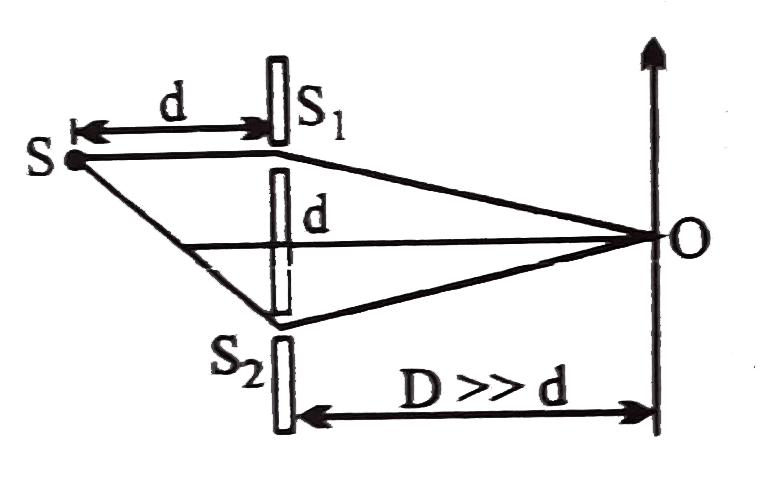
View SolutionIn a doulble slit experiment when a thin film of thickness t having refractive index μ is introduced in from of one of the slits, the maximum at the centre of the fringe pattern shifts by one width. The value of t is (λ is the wavelength of the light used)
View SolutionIn the ideal double-slit experiment, when a glass-plate (refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wavelength λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate is
View SolutionIn the ideal double-slit experiment, when a glass-plate (refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wavelength λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate is
View SolutionThe central fringe shifts to the position of fifth bright fringe, if a thin film of refractive index 1.5 is introduced in the path of light of wavelength 5000Å. The thickness of the glass plate is
View Solution
