The ratio of radii of nuclei .13A127 and .52XA is 3:5. The number of neutrons in the nuclei of X will be
52
73
125
13
The correct Answer is:B
To solve the problem, we need to find the number of neutrons in the nucleus of element X given the ratio of the radii of the nuclei of 2713Al and A52X is 3:5.
1. Understanding the Relationship of Nuclear Radius:
The radius of a nucleus is given by the formula:
R=R0A1/3
where R0 is a constant and A is the atomic mass number.
2. Setting Up the Radii for Both Nuclei:
Let R1 be the radius of the aluminum nucleus and R2 be the radius of the nucleus of element X.
- For aluminum (2713Al):
R1=R0(27)1/3
- For element X:
R2=R0A1/3
3. Taking the Ratio of the Radii:
According to the problem, the ratio of the radii is given as:
R1R2=35
Substituting the expressions for R1 and R2:
R0(27)1/3R0A1/3=35
The R0 cancels out:
(27)1/3A1/3=35
4. Cubing Both Sides:
To eliminate the cube root, we cube both sides:
27A=(35)3
Calculating (35)3:
(35)3=27125
Thus, we have:
27A=27125
5. Cross-Multiplying to Solve for A:
Cross-multiplying gives:
27×125=27×A
Dividing both sides by 27:
A=125
6. Finding the Number of Neutrons:
The atomic mass A is the sum of the number of protons and neutrons:
A=Z+N
where Z is the number of protons and N is the number of neutrons. For element X:
- Z=52 (given)
- A=125 (calculated)
Thus:
N=A−Z=125−52=73
Final Answer:
The number of neutrons in the nucleus of element X is 73.
---
The radius of a nucleus is given by the formula:
R=R0A1/3
where R0 is a constant and A is the atomic mass number.
Let R1 be the radius of the aluminum nucleus and R2 be the radius of the nucleus of element X.
- For aluminum (2713Al):
R1=R0(27)1/3
- For element X:
R2=R0A1/3
According to the problem, the ratio of the radii is given as:
R1R2=35
Substituting the expressions for R1 and R2:
R0(27)1/3R0A1/3=35
The R0 cancels out:
(27)1/3A1/3=35
To eliminate the cube root, we cube both sides:
27A=(35)3
Calculating (35)3:
(35)3=27125
Thus, we have:
27A=27125
Cross-multiplying gives:
27×125=27×A
Dividing both sides by 27:
A=125
The atomic mass A is the sum of the number of protons and neutrons:
A=Z+N
where Z is the number of protons and N is the number of neutrons. For element X:
- Z=52 (given)
- A=125 (calculated)
N=A−Z=125−52=73
The number of neutrons in the nucleus of element X is 73.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.215videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.315videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - Integer type3videos
NUCLEAR PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer12videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Integer Type4videos
PHOTOELECTRIC EFFECT
Similar Questions
The ratio of the radii of the nuclei .2713Al and .52Te125 is approximately-
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
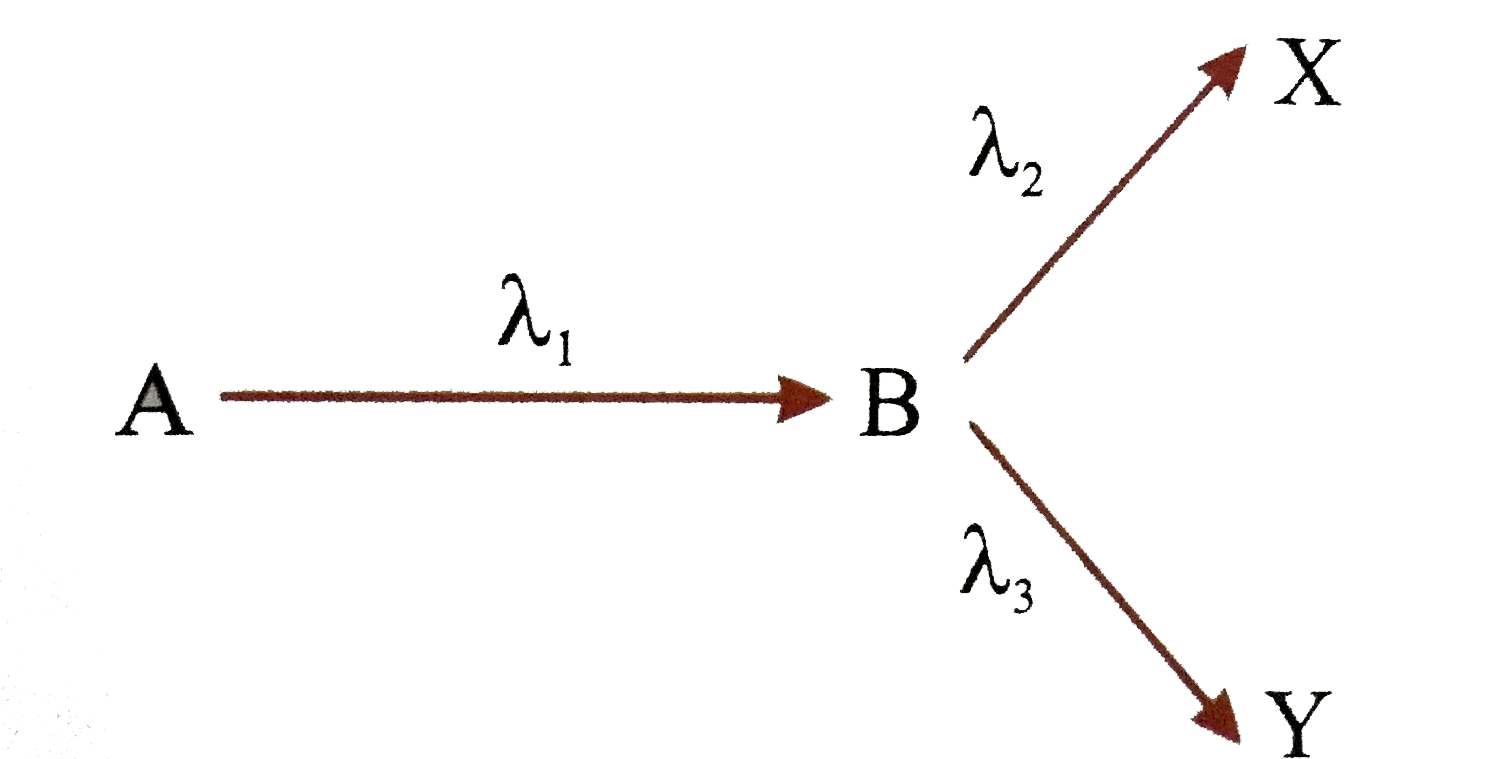
The number of nuclei of B will first increase and then after a maximum value, it decreases forView SolutionA radio nuclide X is produced at constant rate α. At time t=0, number of nuclei of X are zero. Find
(a) the maximum number of nuclei of X.
the number of nuclei at time t.
Decay constant of X is λ.View SolutionStatement : The plot of atomic number ( y -axis ) versus number of neutrons ( x -axis ) for stable nuclei shows a curvature towards x-axis fron the line of 45∘ slope as the atomic number is increased .
Explanation : proton -proton electrostatic repulsions begin to overcome attracive forces involving protons and neutrons in heavier nuclides.View SolutionAssuming the radius of a nucleus to be equal to R=1.3A1/3×10−15m. Where A is its mass number, evaluate the density of nuclei and the number of nucleons per unit volume of the nucleus. Take mass of one nucleon =1.67×10−27kg
View SolutionThe rate of biomass production by consumers is called :
View SolutionUnstable nuclei attain stability through disintegration. The nuclear stability is related to neutron proton ratio (n/p). For stable nuclei n/p ratio lies close to unity for elements with low atmoic numbers (20 or less) but it is more than 1 for nuclei having higher atomic numbers. Nuclei having n/p ratio either very high or low undergo nuclear transformation. When n/p ratio is higher than required for stability, the nuclei have the tendency to emit β-rays. while when n/p ratio is lower than required for stability, the nuclei either emits α-particles or a positron or capture K-electron.
For reaction .92M238→.yNx+2.2He4,.yNx→.BLA+2.−1e0
The number of neutrons in the element L isView SolutionTwo radioactive materials X1 and X2 have decay constants 10λ and λ respectively. If initially they have the same number of nuclei, if the ratio of the number of nuclei of X1 to that of X2 will be 1/e after a time n9λ. Find the value of n?
View SolutionA stable nuclei C is formed from two radioactive nuclei A and B with decay constant of λ1 and λ2 respectively. Initially, the number of nuclei of A is N0 and that of B is zero. Nuclei B are produced at a constant rate of P. Find the number of the nuclei of C after time t.
View SolutionTwo radioactive materials X1 and X2 have decayconstants 10λ and λ respectively. If initially they have the same number of nuclei, then the ratio of the number of nuclei ofX1, to that of X2 will be 1e after a time,
View Solution

