Calculate for the reactions
where is the completely deprotonated anion of EDTA. The formation constant for is and for is .
(a)
(b)
(c)
(d)
The correct Answer is:a
To calculate the standard reduction potential E∘ for the reaction:
ZnY2−⇌Zn(s)+Y4−
we will use the given information about the formation constant and the standard reduction potential of zinc.
Step 1: Identify the given values
- The formation constant Kf for the reaction Zn2++Y4−⇌ZnY2− is given as 3.2×1016.
- The standard reduction potential for the reaction Zn2++2e−⇌Zn(s) is E∘=0.76V.
Step 2: Write the expression for the equilibrium constant
The equilibrium constant K for the reverse reaction (which we need) is the reciprocal of the formation constant:
K=1Kf=13.2×1016
Step 3: Use the Nernst equation
The Nernst equation relates the standard reduction potential to the equilibrium constant:
E∘=E∘Zn2+/Zn+0.059nlogK
Where:
- E∘Zn2+/Zn=0.76V
- n=2 (the number of electrons transferred)
- K=13.2×1016
Step 4: Calculate logK
First, we need to calculate logK:
logK=log(13.2×1016)=−log(3.2×1016)=−(log(3.2)+log(1016))=−(log(3.2)+16)
Using log(3.2)≈0.505:
logK≈−(0.505+16)=−16.505
Step 5: Substitute values into the Nernst equation
Now we can substitute the values into the Nernst equation:
E∘=0.76+0.0592×(−16.505)
Calculating the second term:
0.0592≈0.0295
E∘=0.76−0.0295×16.505
E∘=0.76−0.487
E∘≈0.273V
Step 6: Final adjustment
Since the reaction is written in the form of ZnY2−⇌Zn(s)+Y4−, we need to consider the sign of the potential. The potential for the formation of the complex is negative, thus:
E∘=−1.25V
Conclusion
The calculated standard reduction potential E∘ for the reaction is:
−1.25V
- The formation constant Kf for the reaction Zn2++Y4−⇌ZnY2− is given as 3.2×1016.
- The standard reduction potential for the reaction Zn2++2e−⇌Zn(s) is E∘=0.76V.
The equilibrium constant K for the reverse reaction (which we need) is the reciprocal of the formation constant:
The Nernst equation relates the standard reduction potential to the equilibrium constant:
- E∘Zn2+/Zn=0.76V
- n=2 (the number of electrons transferred)
- K=13.2×1016
First, we need to calculate logK:
Now we can substitute the values into the Nernst equation:
E∘=0.76−0.0295×16.505
E∘=0.76−0.487
E∘≈0.273V
Since the reaction is written in the form of ZnY2−⇌Zn(s)+Y4−, we need to consider the sign of the potential. The potential for the formation of the complex is negative, thus:
The calculated standard reduction potential E∘ for the reaction is:
Topper's Solved these Questions
- ELECTROCHEMISTRYBook:CENGAGE CHEMISTRY ENGLISHChapter:ELECTROCHEMISTRYExercise:Exerciseassertion -Reasoning
Explore 25 Videos
- ELECTROCHEMISTRYBook:CENGAGE CHEMISTRY ENGLISHChapter:ELECTROCHEMISTRYExercise:Exerciseinterger
Explore 8 Videos
- ELECTROCHEMISTRYBook:CENGAGE CHEMISTRY ENGLISHChapter:ELECTROCHEMISTRYExercise:Exercisemultiple Correct Ansers
Explore 53 Videos
- D AND F BLOCK ELEMENTSBook:CENGAGE CHEMISTRY ENGLISHChapter:D AND F BLOCK ELEMENTSExercise:Archives Subjective
Explore 29 Videos
- GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSBook:CENGAGE CHEMISTRY ENGLISHChapter:GENERAL PRINCIPLES AND PROCESS OF ISOLATION OF ELEMENTSExercise:Archives (Subjective)
Explore 14 Videos
Similar Questions
Eo for the reaction Fe+Zn2+→Zn+Fe2+ is –0.35 V. The given cell reaction is :
View Solution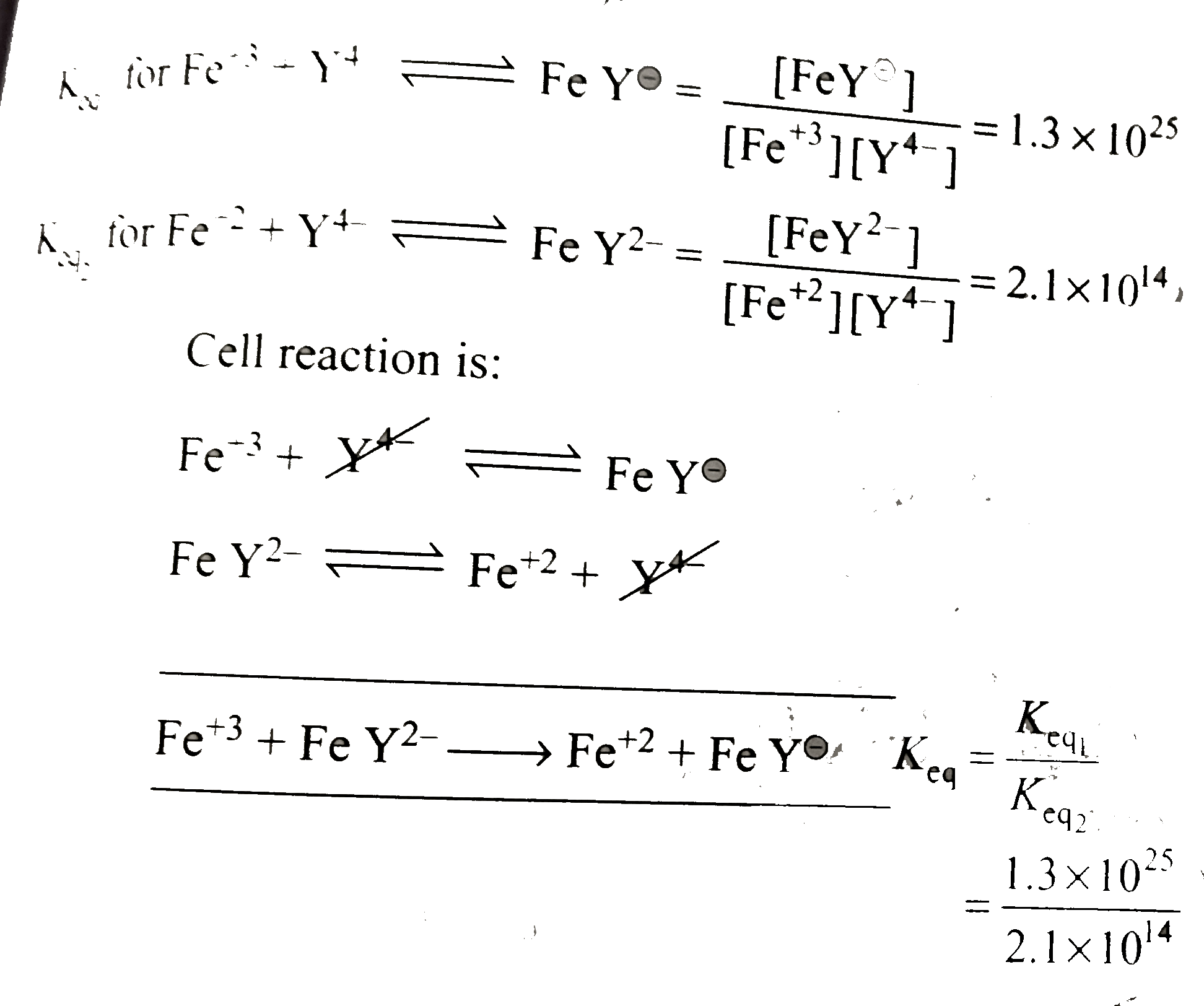
Ec− for FeYc−+ec−→FeY2−
given: Fe+3/+2=0.77V
(a)0.13V
(b)−0.636V
(c)+0.636V
(d)1.41V
View SolutionThe standard potential E∘ for the half reactions are as :
Zn→Zn2++2e−,E∘=0.76V
Cu→Cu2++2e−,E∘=−0.34V
The standard cell voltage for the cell reaction is ?
Zn+Cu2→Zn2++Cu
View SolutionThe reaction Zn2++2e–→Zn(s) has a standard potential of –0.76V. This means:
View SolutionThe electrode potential, E∘, for the reduction of MnO−4 to Mn2+ in acidic medium is +1.51V. Which of the following metal(s) will be oxidised? The reduction reactions and standard electrode potentials for Zn2+,Ag+andAu+ are given as
Zn2+(aq)+2e−→Zn(s),E∘=−0.762V
Ag+(aq)+e−⇔Ag(x),E∘=+0.80V
Au+(aq)+e−⇔Au(s),E∘=+1.69V
View SolutionCalculate Ecell at 25∘C for the reaction :
Zn+Cu2+(0.20M)→Zn2+(0.50M)+Cu
Given EΘ(Zn2+/Zn)=−0.76V,EΘ(Cu2+/Cu)=0.34V.
View SolutionAg++e−→Ag,E0=+0.8VandZn2++2e−→Zn,E0=−076V. Calculate the cell potential for the reaction, 2Ag+Zn2+→Zn+2Ag+
View SolutionFor a reaction A(s)+B2+→B(s)+A2+, at 25∘CEocell=0.2955V. Hence, Keq will be :
View SolutionCalculate the equilibrium constant for the reaction at 298K.
Zn(s)+Cu2+(aq)⇔Zn2+(aq)+Cu(s)
Given, E∘Zn2+/Zn=−0.76V and E∘Cu2+/Cu=+0.34V
View SolutionCalculate the equilibrium constant for the reaction at 298K.
Zn(s)+Cu2+(aq)⇔Zn2+(aq)+Cu(s)
Given, E∘Zn2+/Zn=−0.76V and E∘Cu2+/Cu=+0.34V
View Solution
