Mechanism of the reaction is:
...(i)
...(ii)
...(iii)
What is (a) , (b )
To solve the problem, we will analyze the given reaction mechanism step by step and derive the required expressions for (−d(A))/(dt) and (d(C))/(dt).
Step 1: Understand the Reaction Mechanism
The reaction mechanism consists of two steps:
1. A \xrightleftharpoons(k_2){k_1} B (Equation i)
2. B + A \xrightleftharpoons(k_3){C} (Equation ii)
From these reactions, we can see that A is being converted to B and C through the intermediates.
Step 2: Write the Rate of Change for (−d(A)/dt)
To find the rate of disappearance of A, we need to consider both reactions:
1. In the first reaction (i), A is converted to B:
- The rate of formation of B from A is given by k1(A).
- The rate of disappearance of B back to A is given by k2(B).
2. In the second reaction (ii), A is consumed along with B to form C:
- The rate of this reaction is k3(A)(B).
Combining these, the overall rate of disappearance of A can be expressed as:
−d(A)dt=k1(A)−k2(B)+k3(A)(B)
Step 3: Write the Rate of Change for (d(C)/dt)
To find the rate of formation of C, we focus on the second reaction (ii):
- The formation of C occurs when B and A react together, which is represented by the rate constant k3.
Thus, the rate of formation of C is given by:
d(C)dt=k3(A)(B)
Final Expressions
(a) The expression for the rate of disappearance of A is:
−d(A)dt=k1(A)−k2(B)+k3(A)(B)
(b) The expression for the rate of formation of C is:
d(C)dt=k3(A)(B)
The reaction mechanism consists of two steps:
1. A \xrightleftharpoons(k_2){k_1} B (Equation i)
2. B + A \xrightleftharpoons(k_3){C} (Equation ii)
To find the rate of disappearance of A, we need to consider both reactions:
- The rate of formation of B from A is given by k1(A).
- The rate of disappearance of B back to A is given by k2(B).
- The rate of this reaction is k3(A)(B).
−d(A)dt=k1(A)−k2(B)+k3(A)(B)
To find the rate of formation of C, we focus on the second reaction (ii):
- The formation of C occurs when B and A react together, which is represented by the rate constant k3.
d(C)dt=k3(A)(B)
(a) The expression for the rate of disappearance of A is:
−d(A)dt=k1(A)−k2(B)+k3(A)(B)
d(C)dt=k3(A)(B)
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example
52 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)
9 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Mechanism of the reaction is:
Ak1−−→B,2Ak2−−→C+D
What is −d[A]dt ?
View SolutionMechanism of the reaction is:
A2k⇐⇒k'2A
A+B2k2−−→C+B
B+A2k2−−→C+A
What is−d[A2]dt
View SolutionMechanism of the reaction is:
A2k2⇐⇒2A
A+Bk2−−→C
A2+Ck−−→D+A
What is d[D]dt ?
View SolutionMechanism of the reaction is:
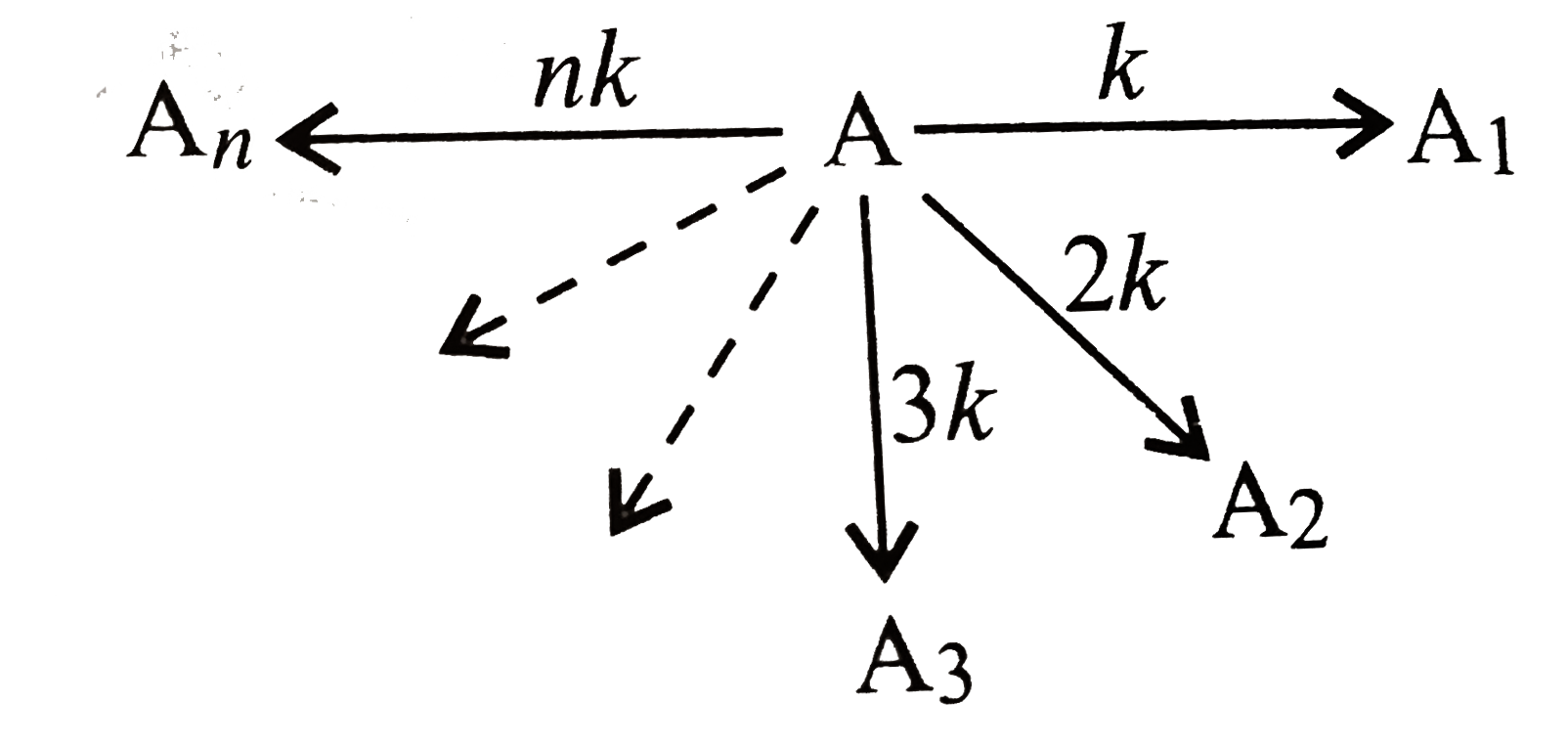
What is (a) −d[A]dt, (b) d[A1]dt
View SolutionConisder the following reactions:
I. A+Bk1⇐⇒k−1C
II. C+Bk2−−→D
Then k1[A][B]−k−1[C]−k2[C][B] is equal to
(a) −d[A]dt
(b)−d[B]dt
(c)d[C]dt
(d)d[D]dt
View SolutionFor an elementary chemical reaction, A2k1⇐===⇒k−12A, the expression for d[A]dt is
View SolutionFor an elementary chemical reaction, A2k1⇐===⇒k−12A, the expression for d[A]dt is
View SolutionA proposed mechanism for a reaction A→B is
Ak1⇐⇒k2C Ck3⇐⇒k4B
Determine the expresison for the equilibrium constant for the overall reaction in terms of the rate constants.
View SolutionThe mechanism of the reaction
2NO+O2→2NO2 is
NO+NOk1⇐⇒k−1N2O2(fast)
N2O2+O2k2−−→2NO2(slow)
The rate constant of the reaction is
View SolutionIn the reaction sequence
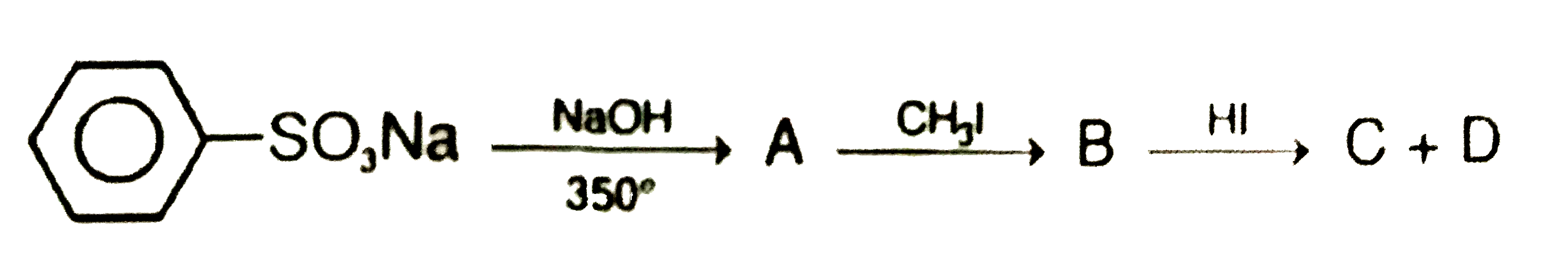 NaOH−−−→350∘ACH3I−−→BHI−−→C+D ltbr> A, B, C, and D are given by the set :
NaOH−−−→350∘ACH3I−−→BHI−−→C+D ltbr> A, B, C, and D are given by the set :
View Solution
