Conisder the following reactions:
I. A+Bk1⇐⇒k−1C
II. C+Bk2−−→D
Then k1[A][B]−k−1[C]−k2[C][B] is equal to
(a) −d[A]dt
(b)−d[B]dt
(c)d[C]dt
(d)d[D]dt
−d[A]dt
−d[B]dt
d[C]dt
d[D]dt
The correct Answer is:c
To solve the problem, we need to analyze the given reactions and their rate expressions.
Step 1: Write the rate expressions for the reactions
We have two reactions:
1. A+Bk1⇌C
2. C+Bk2→D
For the first reaction, the rate of formation of C can be expressed as:
Rate of formation of C=k1(A)(B)−k−1(C)
For the second reaction, the rate of disappearance of C can be expressed as:
Rate of disappearance of C=k2(C)(B)
Step 2: Combine the rate expressions
The net rate of change of concentration of C can be expressed as:
d(C)dt=Rate of formation of C−Rate of disappearance of C
Substituting the expressions from above, we get:
d(C)dt=(k1(A)(B)−k−1(C))−k2(C)(B)
Step 3: Rearranging the expression
Rearranging the equation gives:
d(C)dt=k1(A)(B)−k−1(C)−k2(C)(B)
Step 4: Identify the expression from the options
From the derived expression, we see that:
k1(A)(B)−k−1(C)−k2(C)(B)=d(C)dt
Thus, the expression k1(A)(B)−k−1(C)−k2(C)(B) is equal to d(C)dt.
Conclusion
The correct answer is:
(c) d(C)dt
---
We have two reactions:
1. A+Bk1⇌C
2. C+Bk2→D
Rate of formation of C=k1(A)(B)−k−1(C)
Rate of disappearance of C=k2(C)(B)
The net rate of change of concentration of C can be expressed as:
d(C)dt=Rate of formation of C−Rate of disappearance of C
Substituting the expressions from above, we get:
d(C)dt=(k1(A)(B)−k−1(C))−k2(C)(B)
Rearranging the equation gives:
d(C)dt=k1(A)(B)−k−1(C)−k2(C)(B)
From the derived expression, we see that:
k1(A)(B)−k−1(C)−k2(C)(B)=d(C)dt
The correct answer is:
(c) d(C)dt
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Solved Example
52 Videos
- CHEMICAL KINETICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Ex 4.1 (Objective)
9 Videos
- CARBOXYLIC ACIDS AND THEIR DERIVATIVESBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CARBOXYLIC ACIDS AND THEIR DERIVATIVESEXERCISE - Exercises Archives (Analytical And Descriptive)
34 Videos
- COORDINATION COMPOUNDSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - COORDINATION COMPOUNDSEXERCISE - Archives Subjective
18 Videos
Similar Questions
Mechanism of the reaction is:
Ak2⇐⇒k1B ...(i)
B+Ak3⇐⇒C ...(ii)
¯¯¯¯¯¯¯¯¯¯¯¯¯¯2A→C––––––––– ...(iii)
What is (a) −d[A]dt, (b ) d[C]dt
View SolutionMechanism of the reaction is:
A2k2⇐⇒2A
A+Bk2−−→C
A2+Ck−−→D+A
What is d[D]dt ?
View SolutionMechanism of the reaction is:
A2k⇐⇒k'2A
A+B2k2−−→C+B
B+A2k2−−→C+A
What is−d[A2]dt
View SolutionMechanism of the reaction is:
Ak1−−→B,2Ak2−−→C+D
What is −d[A]dt ?
View SolutionFor an elementary chemical reaction, A2k1⇐===⇒k−12A, the expression for d[A]dt is
View SolutionFor an elementary chemical reaction, A2k1⇐===⇒k−12A, the expression for d[A]dt is
View SolutionMechanism of the reaction is:
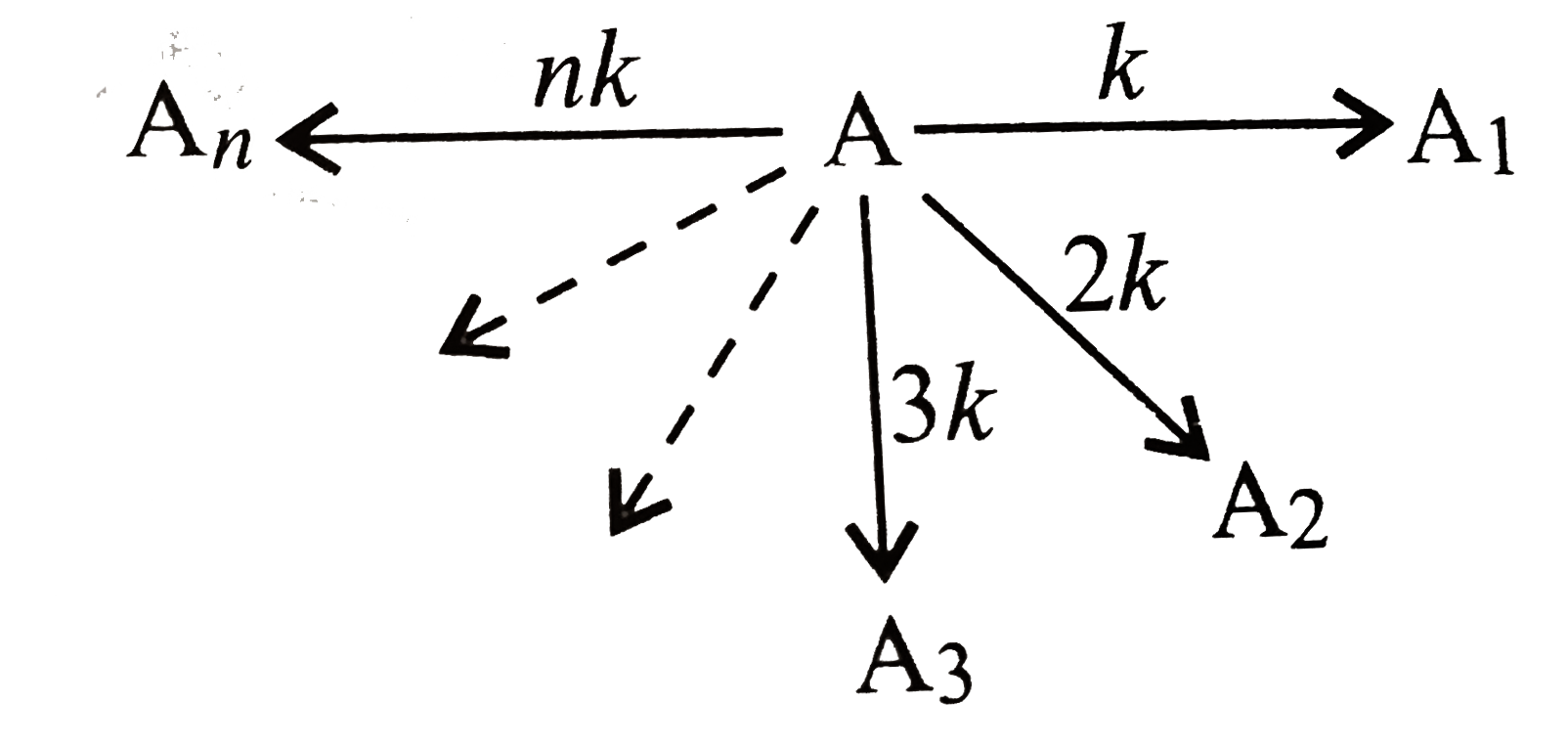
What is (a) −d[A]dt, (b) d[A1]dt
View SolutionFor the following mechanism, P+QKA⇐⇒KBPQ
KC⇐⇒KD R at equilibrium [R][P][Q] is: [K represents rate constant]
View SolutionFor and elementary reaction
2Ak1⇐⇒k2B, the rate of disappearance of A iss equal to
(a) 2k1k2[A]2
(b) −2k1[A]2+2k2[B]
( c) 2k1[A]2−2k2[B]
(d) (2k1−k2)[A]
View SolutionThe overall rate d[P]dt, for the reaction 2AK⇐⇒B,B+Ckf−−→P is given by
View Solution
