If the amount of a radioactive substance is increased three times, the number of atoms disintegrated per unit time would
Be doulbe b.Be triple
c. Remian one-third d. Not change
a. Be double
b.Be triple
c. remain one-third
d. Not change
The correct Answer is:b.Be triple
To solve the question, we need to understand the relationship between the amount of a radioactive substance and its activity, which is defined as the number of atoms disintegrated per unit time.
1. Understanding Activity:
- The activity (A) of a radioactive substance is defined as the number of disintegrations (or decays) per unit time. It is given by the formula:
A=λ×N
where λ is the decay constant and N is the number of radioactive atoms present.
2. Initial Condition:
- Let's denote the initial amount of the radioactive substance as N. The initial activity can be expressed as:
Ainitial=λ×N
3. Increasing the Amount:
- If the amount of the radioactive substance is increased three times, the new amount of the substance becomes:
Nnew=3N
4. New Activity Calculation:
- Using the new amount in the activity formula, the new activity becomes:
Anew=λ×Nnew=λ×(3N)=3(λ×N)=3Ainitial
5. Conclusion:
- Since the new activity is three times the initial activity, we can conclude that the number of atoms disintegrated per unit time would be triple the initial amount. Therefore, the answer to the question is:
b. Be triple
- The activity (A) of a radioactive substance is defined as the number of disintegrations (or decays) per unit time. It is given by the formula:
A=λ×N
where λ is the decay constant and N is the number of radioactive atoms present.
- Let's denote the initial amount of the radioactive substance as N. The initial activity can be expressed as:
Ainitial=λ×N
- If the amount of the radioactive substance is increased three times, the new amount of the substance becomes:
Nnew=3N
- Using the new amount in the activity formula, the new activity becomes:
Anew=λ×Nnew=λ×(3N)=3(λ×N)=3Ainitial
- Since the new activity is three times the initial activity, we can conclude that the number of atoms disintegrated per unit time would be triple the initial amount. Therefore, the answer to the question is:
b. Be triple
Topper's Solved these Questions
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Solved Example
18 Videos
- NUCLEAR CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.1 Objective
15 Videos
- NCERT BASED EXERCISEBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)
29 Videos
- ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive
24 Videos
Similar Questions
When the quantity of a radioactive substance is increased two times, the number of atoms disintegrating per unit time is
View SolutionThe rate of disintegration of a radioactive substance is …………………………… to the number of atoms present at that time .
View Solution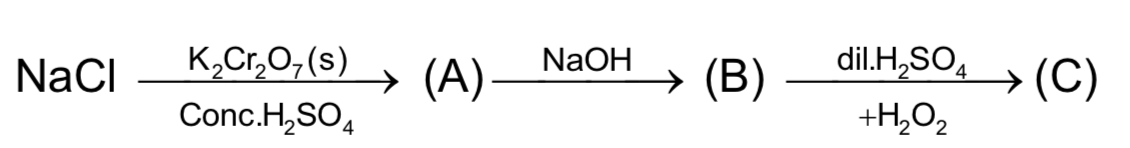
Determine total number of atoms in per unit formula of (A),(B) and (C)
View SolutionIf time t is required for a radioactive substance to become one third of its initial amount, what fraction would be left after 0.5 t ?
View Solution1g of a radioactive substance disintegrates at the rate of 3.7×1010 disintegrations per second. The atomic massof the substance is 226. Calculate its mean life.
View SolutionIn a radioactive substance at t=0, the number of atoms is 8×104. Its half-life period is 3 years. The number of atoms 1×104 will remain after interval.
View SolutionIn a radioactive disintegration, the ratio of initial number of atoms to the number of atoms present at an instant of time equal to its mean life is
View SolutionFind the decay rate of the substance having 4×1015 atoms. Half life of a radioactive substances in α-decay is 1.2×107 is
View SolutionThe length of a rectangle is reduced to its one-third and its breadth is increased three times. Find the new perimeter and the new area.
View SolutionAnswer the following:
(a) For a radioactive substance, show the variation of the total mass disintegrated as a function of time t graphically.
(b) Initial mass of a radioactive substance is 3.2mg. It has a half-life of 4h. find the mass of the substance left undecayed after 8h.
View Solution
