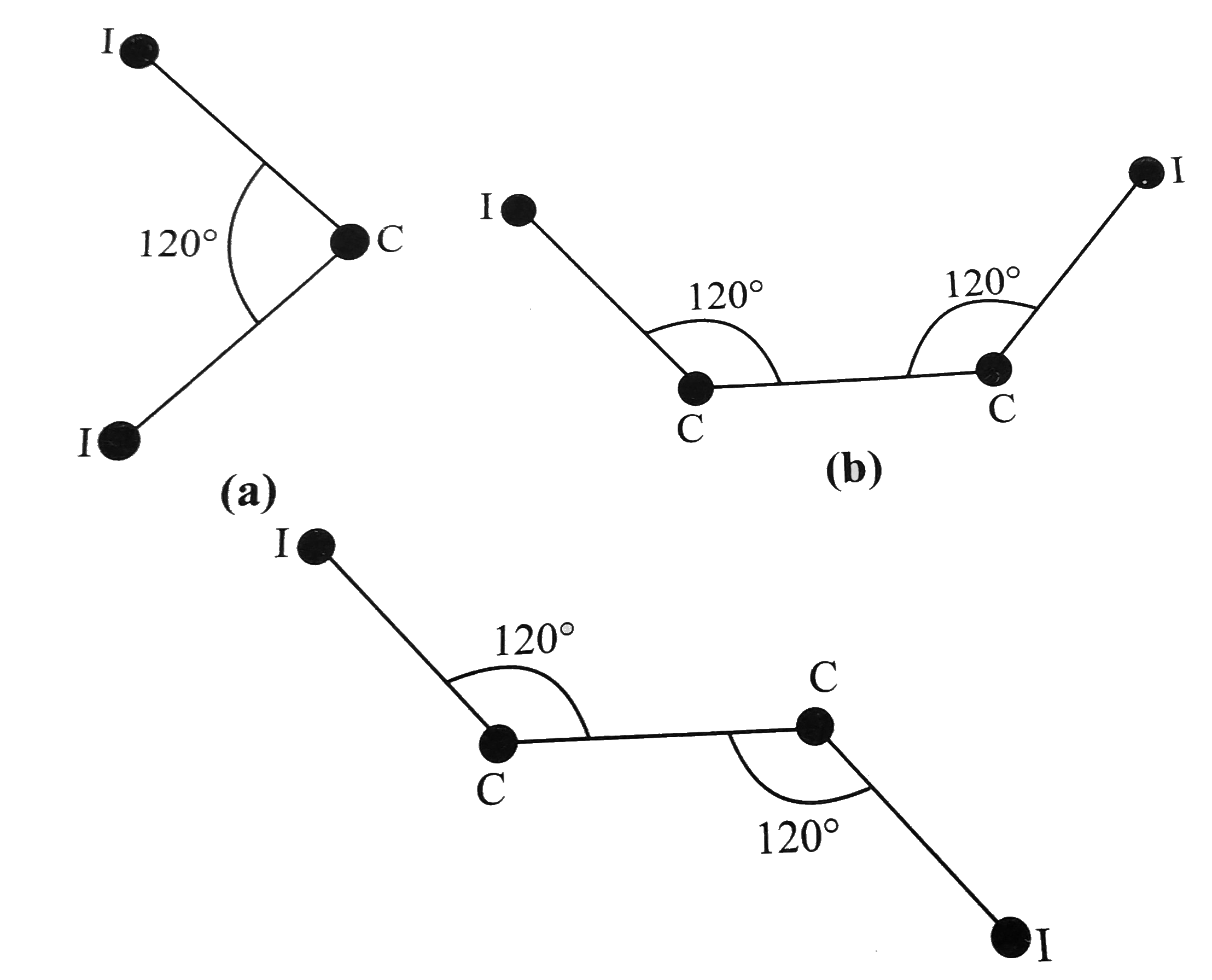
Calculate the I-I distance in each of the isomeric compounds C2H2I2 as shown below
Give Bond length of C−I⇒210 pm
Bond length of C=C⇒133 pm

 .
.
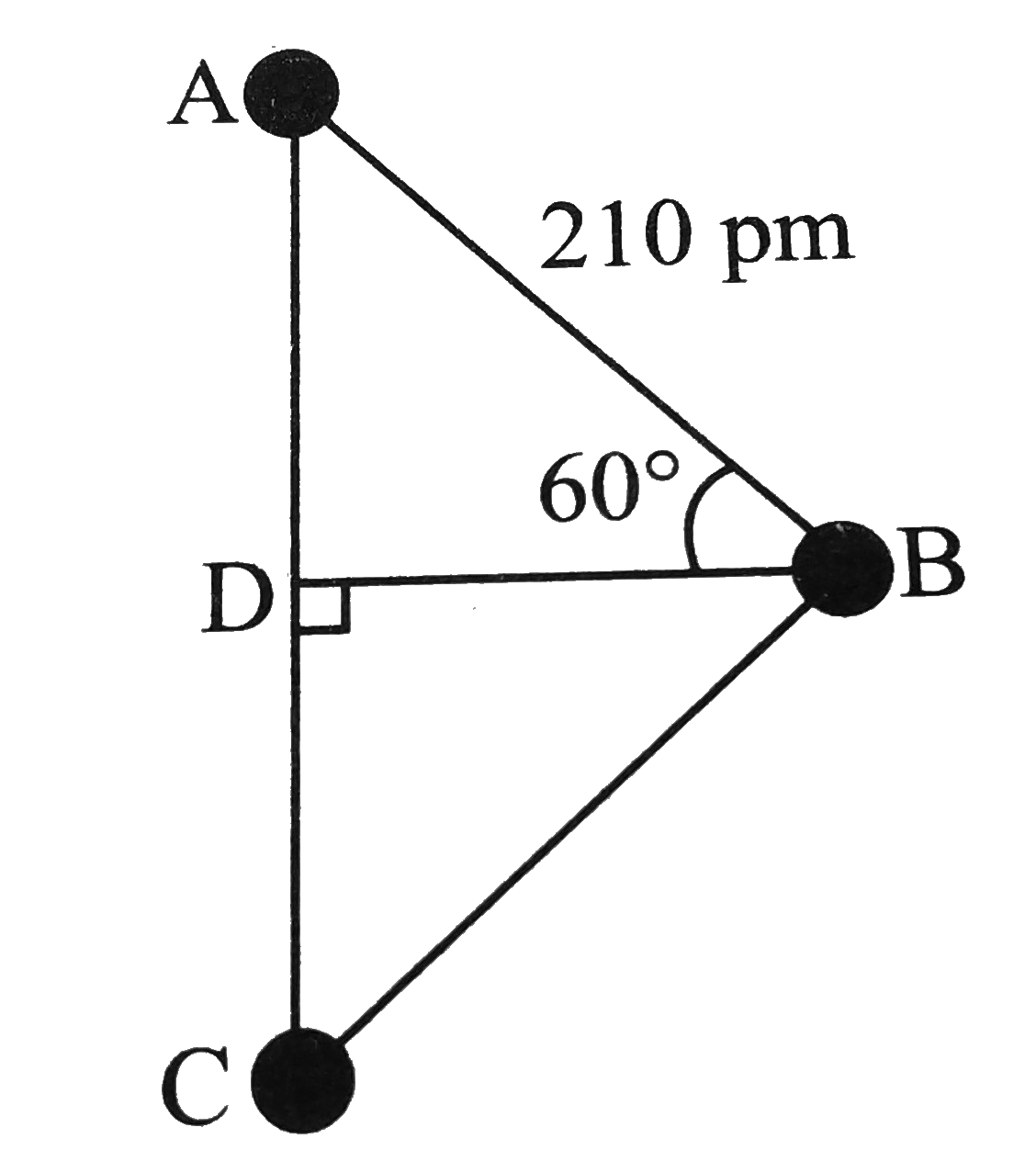
The I -I distance ¯¯¯¯¯¯¯¯¯(AC)=¯¯¯¯¯¯¯¯¯(AD)+¯¯¯¯¯¯¯¯¯(DC)=¯¯¯¯¯¯¯¯¯¯¯(2AD)
C−I bond length ¯¯¯¯¯¯¯¯¯(AB)=210 pm
In the 60∘ right angle triangle (ABD)
2¯¯¯¯¯¯¯¯¯(AD)=2(210sin60∘)=364 pm
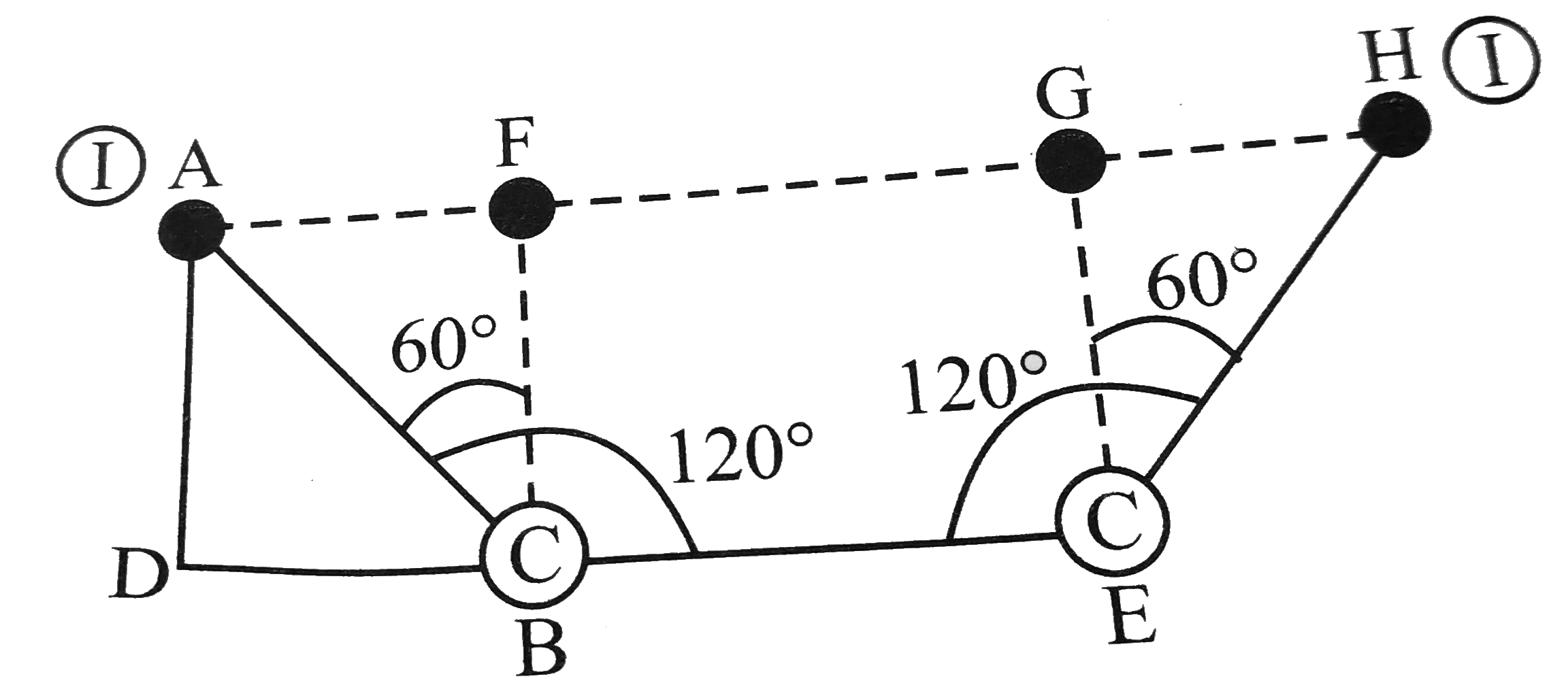
The I-I distance =¯¯¯¯¯¯¯¯¯(AH)=¯¯¯¯¯¯¯¯¯(AF)+¯¯¯¯¯¯¯¯¯¯(GH)+¯¯¯¯¯¯¯¯¯(FG)
Now ¯¯¯¯¯¯AF=¯¯¯¯¯¯¯GH=¯¯¯¯¯¯BD=210cos60∘=105 pm
¯¯¯¯¯¯FG is the C=C double bond distance =133 pm
Thus, ¯¯¯¯¯¯AH=105+133+105=343 pm
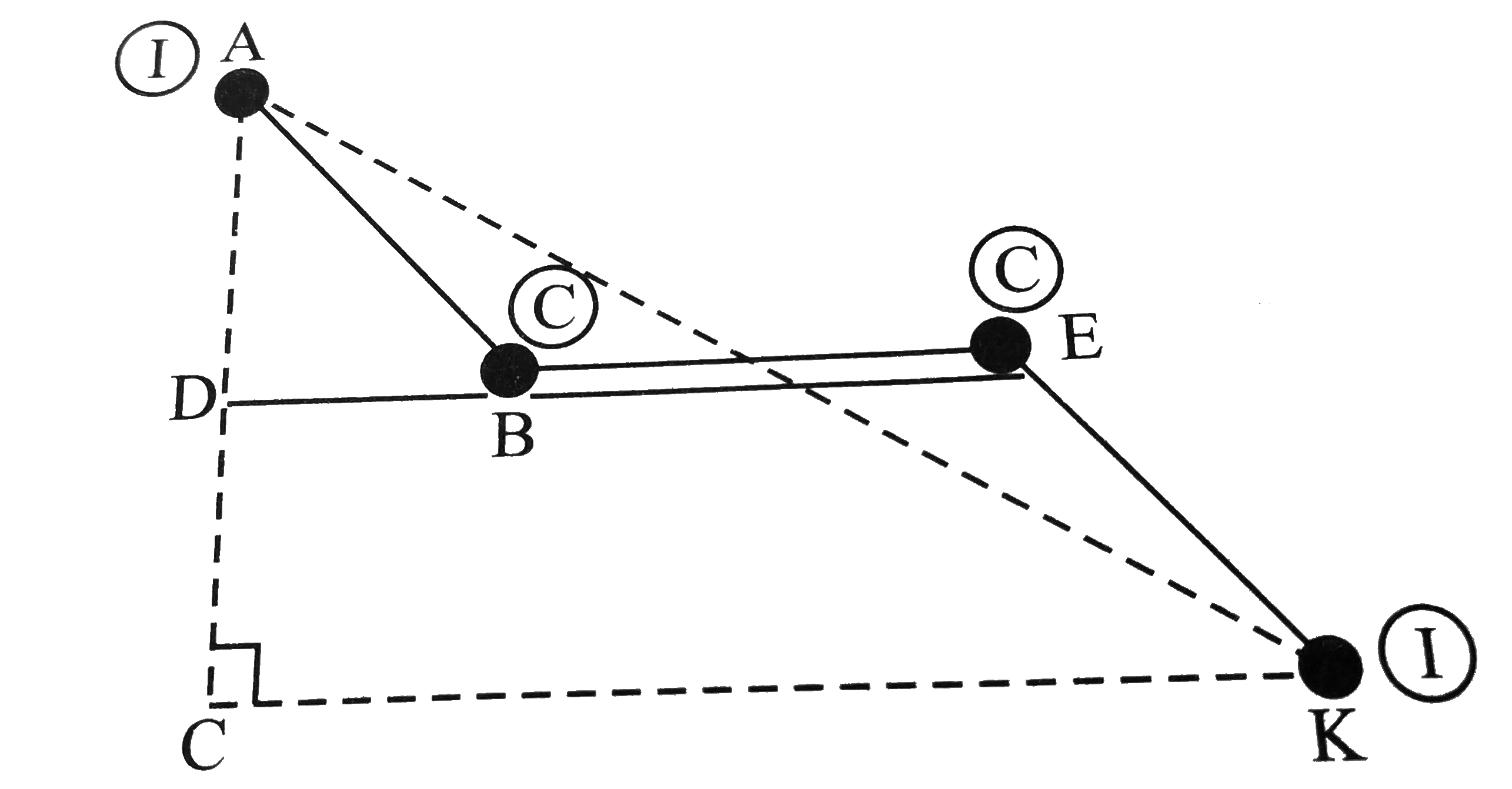
The I-I distance =AK the hypotenuse or right angle triangle ACK
The legs of the triangle ACK are AC are AC and CK
AC=364 pm (as calculated in part (a) above)
¯¯¯¯¯¯CK=¯¯¯¯¯¯AH (as calculated in part (b) above)
=343 pm
Then, ¯¯¯AK=√¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯(CK)2+(¯¯¯¯¯¯AC)2
=√¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯(343)2+(364)2
=500 pm
 .
.
Topper's Solved these Questions
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Ex 2 .2 Subjective (Intermolecular Forces And H-Bonding)
7 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Ex 2 .2 Subjective (Molecular Orbital Theory)
4 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Archives Subjective
15 Videos
- ATOMIC STRUCTUREBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ATOMIC STRUCTUREEXERCISE - Concept Applicationexercise(4.3)
19 Videos
- CHEMICAL EQUILIBRIUMBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Subjective type
1 Videos
Similar Questions
Write a Lewis structure for CCl2F2 one of the compounds indicated in the depletion of stratoshpheric ozone .
View SolutionIdentify which of them are polar and non-polar
(a) HF (b) BeCI2 (c ) HgCI2 (d) NH3
(e) H2O (f) N2 (g) AICI3 (h) CCI4
(i) CI2 (j) SiCI4 .
View SolutionState four major physical properties that can be used to distinguish between covalent and ionic compounds. Mention the distinguishing features in each case.
View SolutionHow many quantum number are needed in designate an orbital ? Name them
View SolutionThe equilibrium constant Kp of the reaction: 2SO2+O2⇔2SO3 is 900atm−1 at 800K. A mixture constaining SO3 and O2 having initial pressure of 1 atm and 2 atm respectively, is heated at constant volume to equilibriate. Calculate the partial pressure of each gas at 800K at equilibrium.
View Solution(a) The EN of cesium (Cs) is 0.7 and that of chlorine (Cl) is 3.5 . Predict tha bond formed between them. (b) The X-X bond length is 100 pm and C-C bond length is 154 pm . If EN of 'X' and 'C' are 3.0 and 2.0 respectively, calculate the C-X bond length. (i) 127 pm , (ii) 118 pm (iii) 108 pm , (iv) 128 pm ( c) Which of the properties can be predicted by EN values? (d) If a,b and c are EN , IE and EA respectively. What is the formula of EA ( c) in the terms of EN (a) and IE (b) ?
View SolutionCalculate the I-I distance in each of the isomeric compounds C_(2)H_(2)I_(2) as shown below Give Bond length of C -I implies 210 pm Bond length of C =C implies 133 pm .
View SolutionCalculate the I-I distance in each of the three isomeric diiodobenzenes Assume that the ring is a regular hexagon and that each C−I bonds lies on a line through the centre of the hexagon .
Given C−I bond length =210 pm
The distance between two adjacent C-atom (i.e C-C)
=140 pm
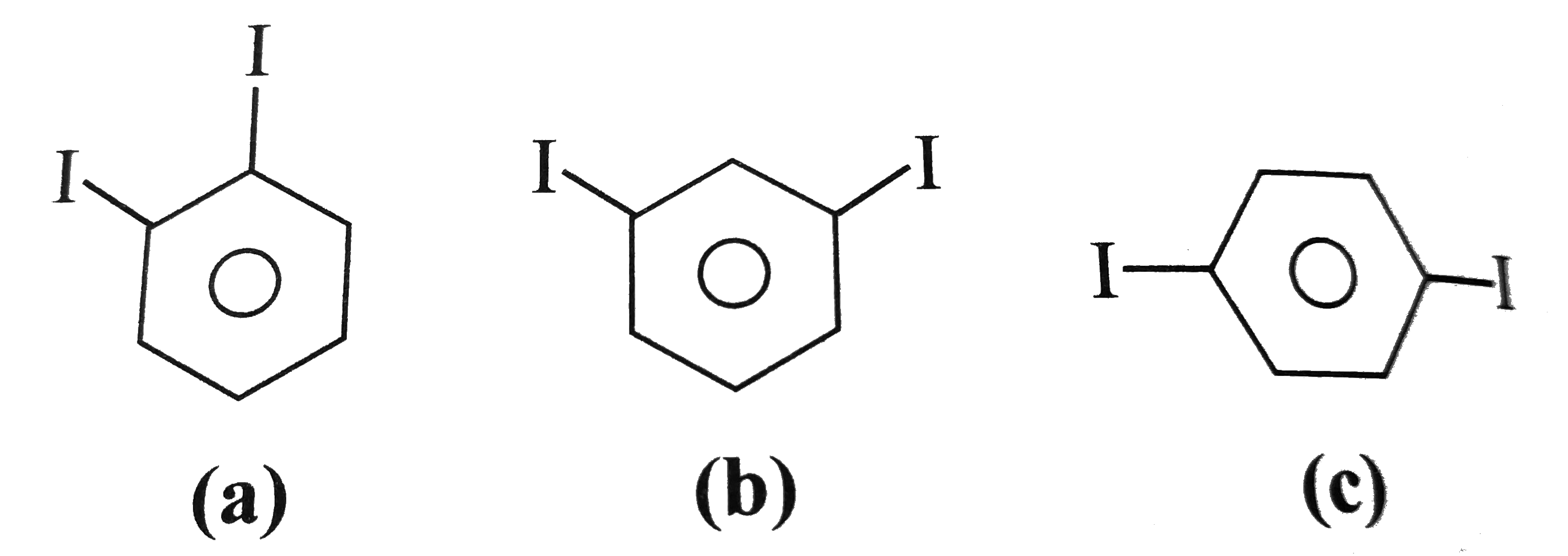 .
.
View SolutionThe interatomic distance in H2 and CI2 molecules are 74 an d198 pm respectively. The bond length of HCI is
View Solutionin isolated condition C−C bond length of C2H4 is x than the bond length ofn C−C bond of C2H4 in Zeise 's salt is .
View SolutionIn diamond, the C - C bond lengths are ______ pm.
View SolutionBond length of A-A bond is 124 pm and bond length of B-B bond is 174 pm. Calculate the bond length (in pm) of A-B bond in AB molecule if percent ionic character of A-B bond is 19.5%
View SolutionThe C-C bond length in propene is little shorter 149 pm than the C-C bond length 154 pm in ethane. This is due to
View SolutionDecreasing order C-C bond length is (I) C_(2)H_(4) , (II) C_(2)H_(2) (III) C_(6)H_(6) , (IV) C_(2)H_(6)
View SolutionComment on the C-C bond length for C2H6 and C2F6 compounds
View SolutionCovalent radius of fluorine is 64 pm but the bond length is not equal to 128 pm and that is 143 pm and bond energy is found to be comparable to I_(2) .
View Solution
