This question has statement and statement II of the four choices given after the statements choose the one that best describes the two statements
Statement-I In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational force. When the particle passes through the centre of the planet sum of kinetic energies of both the bodies is maximum
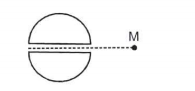
statement-II When the resultant of all forces acting on a particle or a particle like object is constant in direction the kinetic energy of the particle keeps on increasing.
Statement is true Statement-II is true Statement-II is a correct explanation of Statement-I
Statement is true Statement-II is true Statement-II is not a correct explanation of Statement-I
Statement-I is true , Statement-II is false
Statement-I is false , Statement-II is true
The correct Answer is:A
Topper's Solved these Questions
Similar Questions
Statement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.
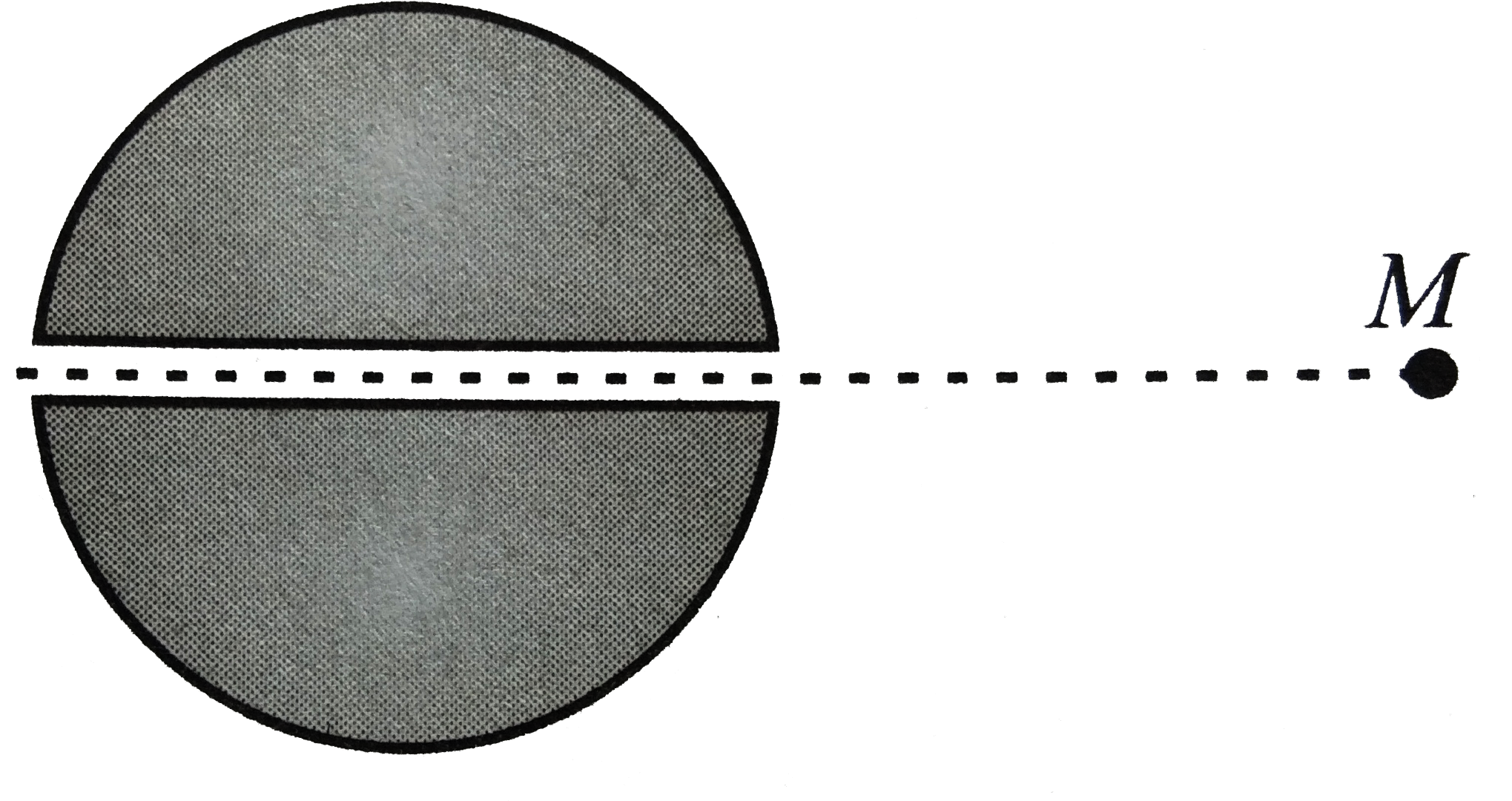
Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.
View SolutionThis questions has Statement I and Statement II. Of the four choices given after the Statements, choose the one that best describes into two Statements.
Statement-I : Higher the range, greater is the resistance of ammeter.
Statement- II : To increase the range of ammeter, additional shunt needs to be used across it.
View Solution
Knowledge Check
Statement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.

Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.AStatement I is True, Statement II is True: Statement II is a correct explanation for Statement I.BStatement I is True, Statement II is True: Statement II is Not a correct explanation for Statement I.CStatement I is True, Statement II is False.DStatement I is False, Statement II is True.This question has statement I and statement II. Of the four choices given after the statements, choose the one that best describes the two statements.
Statement I: A point particle of mass m moving with speed v collides with stationary point particle of mass M. If the maximum energy loss possible glven as f(12mv2) then f=(mM+m)
Statement II: Maximum energy loss occurs when the particles get stuck together as a result of the collision.A(a) Statement -I is true, Statment -II is true, Statement -II is the correct explanation of Statement -I.B(b) Statement-I is true, Statment -II is true, Statement -II is not the correct explanation of Statement -II.C(c) Statement -I is true, Statment -II is false.DStatement -I is false, Statement -II is true.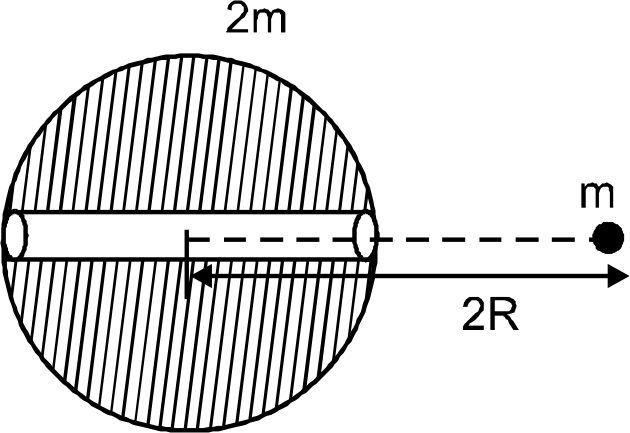
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)A4R3Bvelocity of the cosmic particle at that instant is
√8Gm3RCTotal work done by the gravitational force on both the particle is −2Gm2RDTotal work done by the gravitational force on both the particle is −2Gm2R
This question has statement I and statement II. Of the four choices given after the statements, choose the one that best describes the two statements.
Statement I: A point particle of mass m moving with speed v collides with stationary point particle of mass M. If the maximum energy loss possible glven as f(12mv2) then f=(mM+m)
Statement II: Maximum energy loss occurs when the particles get stuck together as a result of the collision.
View Solution
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View Solution
