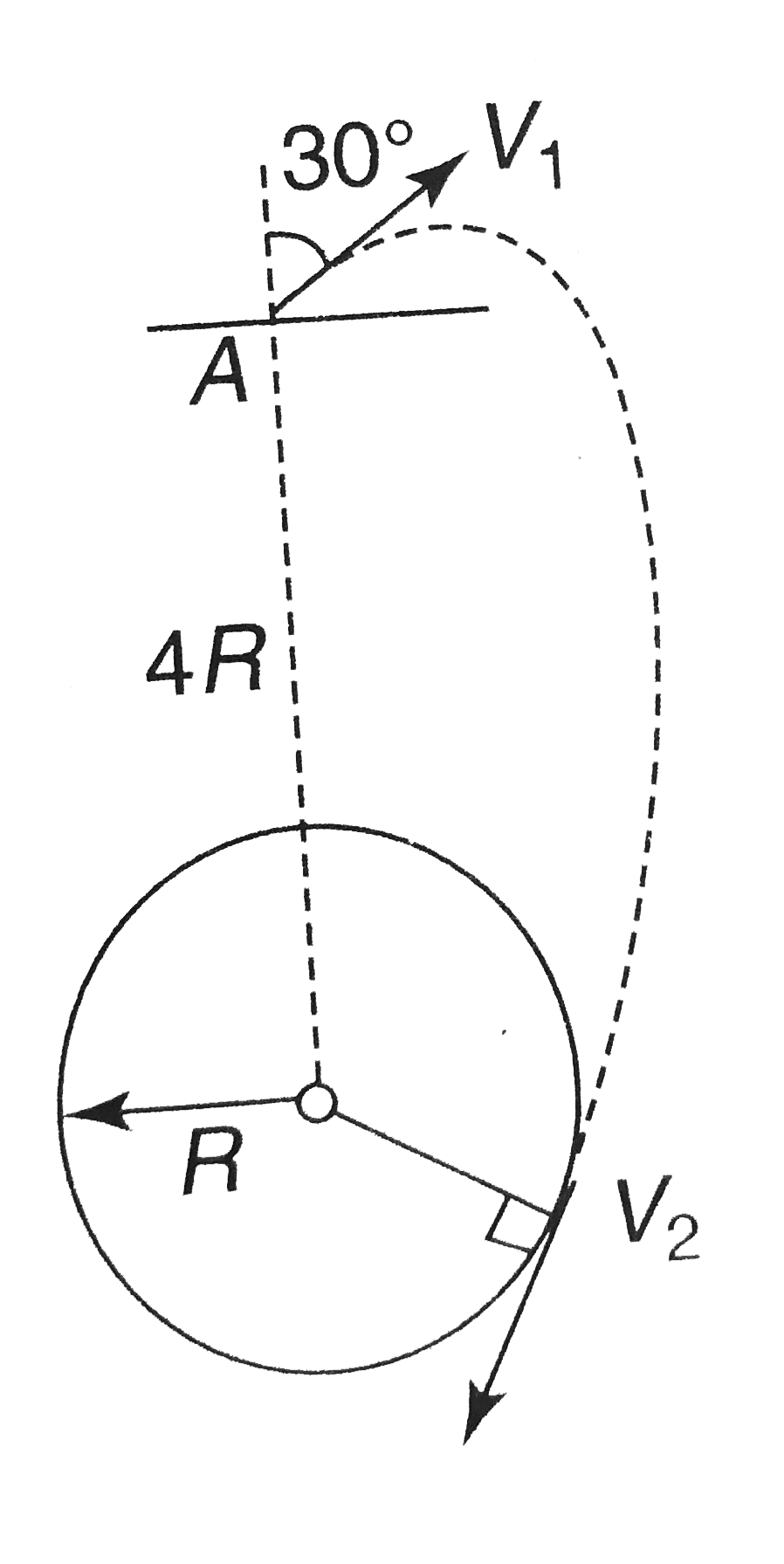
A particle is projected from point A, which is at a distance 4 R from

A particle is projected from point $A$ , that is at a distance $4 R$ form the centre of the earth, with speed $V_{1}$ in a direction making $30^{\circ}$ with the line joining the centre of the earth and point $A$ , as shown. Consider gravitational interaction only between thesetwo. (Use $\frac{G M}{R} = 6.4 \times 10^{7} m^{2} / s^{2}$ ). The speed $V_{1}$ if particle pasess grazing the surface of the earth is

A $2 \sqrt{2} \times 10^{3} m / s$
B $4 \sqrt{2} \times 10^{3} m / s$
C $4 \times 10^{3} m / s$
D $4 \sqrt{3} \times 10^{3} m / s$
A body at rest starts from a point at a distance r (gtR) from the centre of the Earth. If M and R stand for the speed of the body when it reaches the Earth surface is
A $\sqrt{\frac{2 G M}{R}}$
B $\sqrt{\frac{2 G M}{R - r}}$
C $\sqrt{\frac{2 G M (r - R)}{R r}}$
DZero
The distance of the centres of moon the earth is D. The mass of earth is 81 times the mass of the moon. At what distance from the centre of the earth, the gravitational force on a particle will be zero.
A $\frac{D}{2}$
B $\frac{2 D}{3}$
C $\frac{4 D}{3}$
D $\frac{9 D}{10}$

The correct Answer is:8