A uniform magnetic ficld B ы B0j exists in space. A particle of mass m and charge q is projected towards negative x -axis with speed v from the a point (d,0,0). If the maximum value of v for which the particle does not hit y−z plane is 4B0qdαm, then calculate α.
The correct Answer is:4
To solve the problem, we need to analyze the motion of a charged particle in a magnetic field. The particle is projected towards the negative x-axis, and we need to determine the maximum speed v such that the particle does not hit the y−z plane.
1. Understanding the Motion in a Magnetic Field:
When a charged particle moves in a magnetic field, it experiences a magnetic force that acts perpendicular to both the velocity of the particle and the magnetic field direction. This causes the particle to move in a circular path.
2. Magnetic Force:
The magnetic force F acting on the particle is given by:
F=q(v×B)
Since the magnetic field B=B0^j and the velocity v=−v^i, we can compute the cross product:
v×B=(−v^i)×(B0^j)=−vB0(^i×^j)=−vB0^k
Thus, the magnetic force becomes:
F=−qvB0^k
This force acts in the negative z-direction.
3. Radius of the Circular Path:
The radius r of the circular path followed by the particle can be expressed as:
r=mvqB
Substituting B=B0:
r=mvqB0
4. Condition for Not Hitting the y-z Plane:
The particle starts at the point (d,0,0). The particle will not hit the y−z plane if the radius of the circular path is less than or equal to the distance from the x-axis to the y-z plane, which is d:
r≤d
Therefore, we have:
mvqB0≤d
5. Rearranging for Maximum Speed:
Rearranging the inequality gives us:
v≤qB0dm
The maximum value of v for which the particle does not hit the y−z plane is:
vmax=qB0dm
6. Comparing with Given Expression:
According to the problem, the maximum speed is given as:
vmax=4B0qdαm
Setting the two expressions for vmax equal gives:
qB0dm=4B0qdαm
7. Solving for α:
Canceling common terms from both sides (assuming q,B0,d,m≠0):
1=4α
Thus, we find:
α=4
Final Answer:
α=4
When a charged particle moves in a magnetic field, it experiences a magnetic force that acts perpendicular to both the velocity of the particle and the magnetic field direction. This causes the particle to move in a circular path.
The magnetic force F acting on the particle is given by:
F=q(v×B)
Since the magnetic field B=B0^j and the velocity v=−v^i, we can compute the cross product:
v×B=(−v^i)×(B0^j)=−vB0(^i×^j)=−vB0^k
Thus, the magnetic force becomes:
F=−qvB0^k
This force acts in the negative z-direction.
The radius r of the circular path followed by the particle can be expressed as:
r=mvqB
Substituting B=B0:
r=mvqB0
The particle starts at the point (d,0,0). The particle will not hit the y−z plane if the radius of the circular path is less than or equal to the distance from the x-axis to the y-z plane, which is d:
r≤d
Therefore, we have:
mvqB0≤d
Rearranging the inequality gives us:
v≤qB0dm
The maximum value of v for which the particle does not hit the y−z plane is:
vmax=qB0dm
According to the problem, the maximum speed is given as:
vmax=4B0qdαm
Setting the two expressions for vmax equal gives:
qB0dm=4B0qdαm
Canceling common terms from both sides (assuming q,B0,d,m≠0):
1=4α
Thus, we find:
α=4
α=4
Topper's Solved these Questions
Similar Questions
Consider the situation described in the previous example. A particle of mass m having a charge q is placed at a distance d from the metal sheet and is projected towards it. Find the maximum velocity of projection for which the particle does not hit the sheet.
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.View Solution
Knowledge Check
- Question 1 - Select One
A uniform magnetic field B=B0ˆj exists in space. A particle of mass m and charge q is projected towards X-axis with speed v from a point (a,0, 0). The maximum value of v for which the particle does not hit the Y-Z plane is
A2BqdmBBqdmCBq2dmDBqd2m - Question 2 - Select One
A uniform magnetic field B=B0ˆj exists in space. A particle of mass m and charge q is projected towards X-axis with speed v from a point (a,0, 0). The maximum value of v for which the particle does not hit the Y-Z plane is
ABqamBBqa2mCBqamDBq2am - Question 3 - Select One
A plane metallic sheet is placed with its face parallel to lines of magnetic induction B of a uniform field. A particle of mass m and charge q is projected with a velocity v from a distance d from the plane normal to the lines of induction. Then, the maximum velocity of projection for which the particle does not hit the plate is
A2BqdmBBqdmCBqd2mDBqdd
A particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
View SolutionA particle of charge q and mass m is moving with a velocity −vˆi(v≠0) towards a large screen placed int eh Y-Z plane at a distance d. If there is a magnetic field →B=B0ˆk , the minimum value of v for which the particle will not hit the screen is :
View SolutionA charged particle +q of mass m is placed at a distance d from another charge particle −2q of mass 2m in a uniform magnetic field of induction vectro B as shown in the fig.If the particles are projected towards each other with equal speeds v.(Neglect the interaction between the particles)
(a)Find the maximum value of the projection speed Vmax so that the two particles do not collide.
b)Find the time interval after which collision occurs between the particles if projection speed equals 2Vmax
(c)In the previous part,assuming that the particles stick after the collision find the radius of the circular path of the particle in subsequent motion.
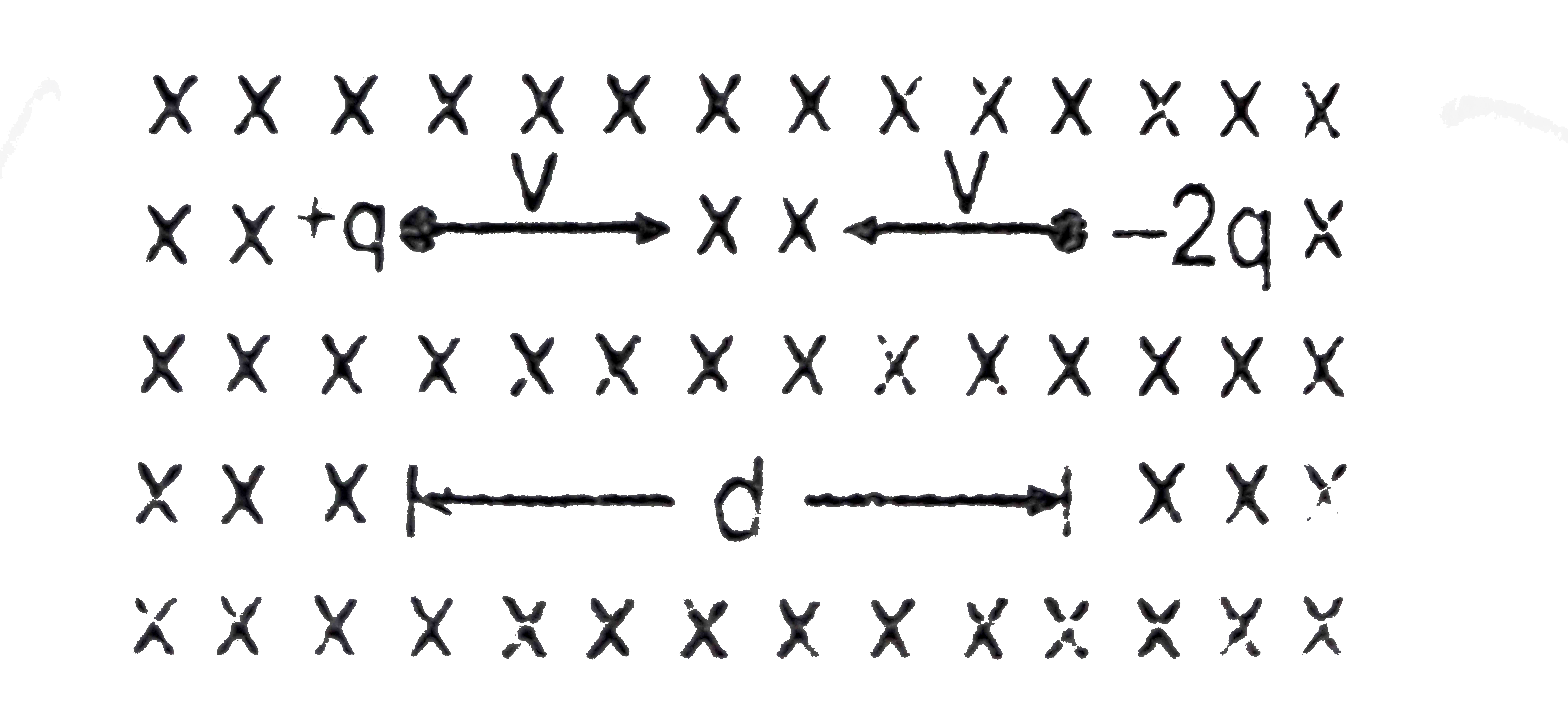 View Solution
View SolutionA uniform electric field →E in the y-direction and uniform magnetic field →B in the x-direction exists in free space. A particle of mass m and carrying charge g is projected from the origin with speed v0 along the y axis. The speed of the particle as a function of its y coordinate will be
View SolutionA particle A of mass m and charge Q moves directly towards a fixed particle B, which has charge Q. The speed of A is a v when it is far away from B. The minimum separtion between the particles is not proportional to
View Solution

