prove by using the principle of similar triangles that:
the line segment drawn parallel to the side of a triangle divides the other sides proportionally. Solution in Assamese
Step by step video & image solution for prove by using the principle of similar triangles that: the line segment drawn parallel to the side of a triangle divides the other sides proportionally. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Express sinθ, cosθ,cotθin terms of secθ .
View SolutionIf the mean of the following data is 18.75,find the value of p.

View SolutionBasic proportionality Theorem or Thales Theorem - If a line is drawn parallel to one side of a triangle intersecting the other two sides; then it divides the two sides in the same ratio.
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
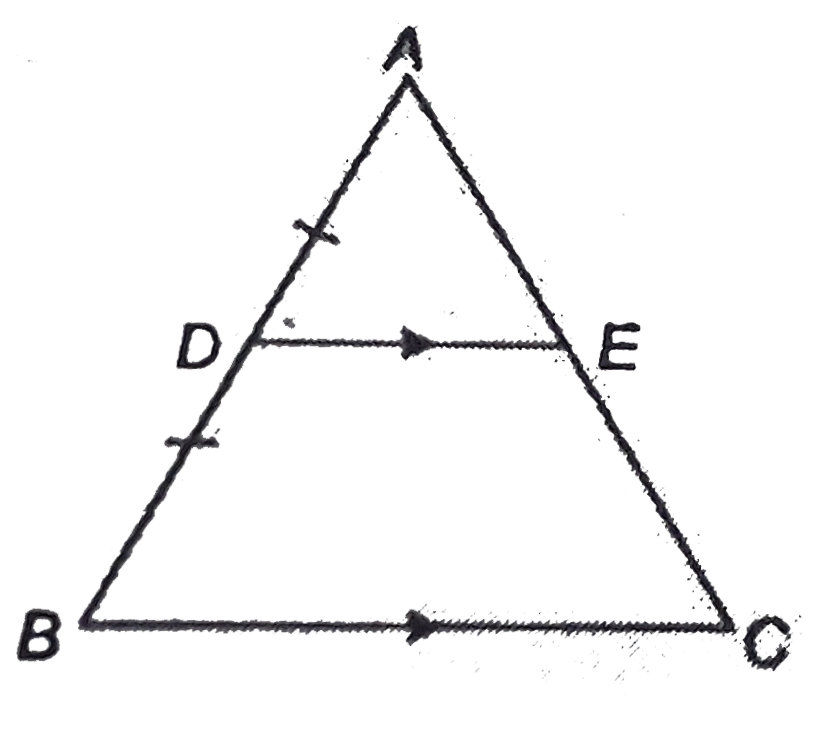
View SolutionProve that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, then the other two sides are divided in the same ratio.
View SolutionProve that a line drawn through the mid-point of one side of a Triangle parallel to another side bisects the third side (Using Basic proportionality theorem).
View SolutionA straight line drawn ____ to a side of a triangle divides the order two sides proportionality.
View SolutionState and prove Thales theorem
Statement
A straight line drawn parallel to a side of triangle intersecting the other two sides , divides the sides in the same ratio.
View SolutionBasic proportionality (Thales' theory): A line drawn parallel to one side of a triangle intersects the other two sides at separate points, and the other two sides are divided by the same ratio.
View SolutionProve that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side (Using basic proportionality theorem).
View SolutionProve that a line joining the midpoints of any two sides of a triangle is parallel to the third side. (Using converse of basic proportionality theorem)
View SolutionProve that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side (Using basic proportionality theorem).
View SolutionProve that a line joining the midpoints of any two sides of a triangle is parallel to the third side. (Using converse of basic proportionality theorem)
View SolutionProve that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side (Using basic proportionality theorem).
View SolutionProve that a line joining the midpoints of any two sides of a triangle is parallel to the third side. (Using converse of basic proportionality theorem)
View SolutionProve that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, then the other two sides are divided in the same ratio.
View Solution
