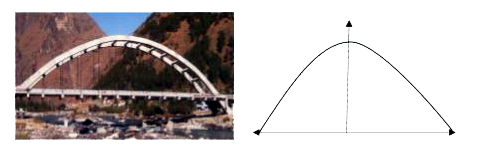
The bridge connects two hills 100 feet apart. The arch on the bridge i

ocr_image
The bridge connects two hills 100 feet apart. The arch on the bridge is in a parabolic form. The highest point on the bridge is 10 feet above the road at the middle of the bridge as seen in the figure.
Based on the information given above, answer the following questions:
The integrand of the integral $\int_{- 50}^{50} x^{2} d x$ is ________ function.

View Solution

ocr_image
The bridge connects two hills 100 feet apart. The arch on the bridge is in a parabolic form. The highest point on the bridge is 10 feet above the road at the middle of the bridge as seen in the figure.
Based on the information given above, answer the following questions:
The equation of the parabola designed on the bridge can be

View Solution

ocr_image
The bridge connects two hills 100 feet apart. The arch on the bridge is in a parabolic form. The highest point on the bridge is 10 feet above the road at the middle of the bridge as seen in the figure.
Based on the information given above, answer the following questions:
The area formed by the curve $x^{2} = 250 y$ , x-axis , y = 0 and y = 10 is

View Solution

ocr_image
The bridge connects two hills 100 feet apart. The arch on the bridge is in a parabolic form. The highest point on the bridge is 10 feet above the road at the middle of the bridge as seen in the figure.
Based on the information given above, answer the following questions:
The area formed between $x^{2} = 250 y$ , y-axis , y = 2 and y = 4 is

View Solution

For the origin O, given two points A and B such that $∠ A O B = 90^{\circ}$ on a parabola $y = x^{2}$ .The line joining A and B has slope 2.On the basis of above information,answer the following questions: The area of $Δ O A B$ is -

View Solution

For the origin O given two points A and B such that $∠ A O B = 90^{\circ}$ on a parabola $y = x^{2}$ .The line joining A and B has slope 2. on the basis of above information answer the following questions: The equation of line AB is

View Solution

Amit is planning to buy a house and the layout is given below. The design and the measurement has been made such that areas of two bedrooms and kitchen together is 95 sq.m.
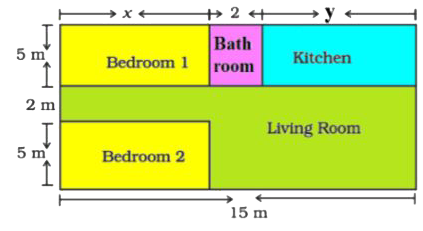
ocr_image
Based on the above information, answer the following questions:
Find the cost of laying tiles in kitchen at the rate of Rs. 50 per sq.m

View Solution

Manjit wants to donate a rectangular plot of land for a school in his village. When he was asked to give dimensions of the plot, he told that if its length is decreased by 50 m and breadth is increased by 50m, then its area will remain same, but if length is decreased by 10m and breadth is decreased by 20m, then its area will decrease by $5300 m^{2}$
Based on the information given above, answer the following questions:
ocr_image
The value of x (length of rectangular field) is

View Solution

Manjit wants to donate a rectangular plot of land for a school in his village. When he was asked to give dimensions of the plot, he told that if its length is decreased by 50 m and breadth is increased by 50m, then its area will remain same, but if length is decreased by 10m and breadth is decreased by 20m, then its area will decrease by $5300 m^{2}$
Based on the information given above, answer the following questions:
ocr_image
The value of y (breadth of rectangular field) is

View Solution

For the origin $O$ , given two points $A$ and $B$ such that $∠ A O B = 90^{\circ}$ on a parabola $y = x^{2}$ . The line joining $A$ and $B$ has slope $2 .$ On the basis of above information, answer the following question: The equation of line AB is

View Solution

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

CBSE MODEL PAPER-QUESTION BANK 2021-QUESTIONS