Three point masses of 1kg, 2kg and 3kg lie at (0,0), (1,2), (3,−1) respectively. Calculate the coordinates of the centre of mass of the system.
To find the coordinates of the center of mass of the system of three point masses, we can use the formula for the center of mass (CM) in two dimensions. The coordinates of the center of mass (X_cm, Y_cm) can be calculated using the following formulas:
1. Identify the masses and their coordinates:
- Mass m1=1kg at coordinates (x1,y1)=(0,0)
- Mass m2=2kg at coordinates (x2,y2)=(1,2)
- Mass m3=3kg at coordinates (x3,y3)=(3,−1)
2. Calculate the total mass:
M=m1+m2+m3=1+2+3=6kg
3. Calculate the x-coordinate of the center of mass:
Xcm=m1x1+m2x2+m3x3M
Substituting the values:
Xcm=1⋅0+2⋅1+3⋅36=0+2+96=116
4. Calculate the y-coordinate of the center of mass:
Ycm=m1y1+m2y2+m3y3M
Substituting the values:
Ycm=1⋅0+2⋅2+3⋅(−1)6=0+4−36=16
5. Final coordinates of the center of mass:
The coordinates of the center of mass are:
(Xcm,Ycm)=(116,16)
Final Answer:
The coordinates of the center of mass are (116,16).
- Mass m1=1kg at coordinates (x1,y1)=(0,0)
- Mass m2=2kg at coordinates (x2,y2)=(1,2)
- Mass m3=3kg at coordinates (x3,y3)=(3,−1)
M=m1+m2+m3=1+2+3=6kg
Xcm=m1x1+m2x2+m3x3M
Substituting the values:
Xcm=1⋅0+2⋅1+3⋅36=0+2+96=116
Ycm=m1y1+m2y2+m3y3M
Substituting the values:
Ycm=1⋅0+2⋅2+3⋅(−1)6=0+4−36=16
The coordinates of the center of mass are:
(Xcm,Ycm)=(116,16)
The coordinates of the center of mass are (116,16).
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions
13 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
Two bodies of masses 1 kg and 3 kg are lying in xy plane at (0, 0) and (2, -1) respectively. What are the coordinates of the centre of mass ?
View SolutionTwo bodies of masses 1 kg and 2 kg are located at (1,2) and (-1,3), respectively. Calculate the coordinates of center of mass.
View SolutionTwo bodies of masses 1kg and 2kg are lying in xy plane at (-1,2) and (2,4) respectively. What are the coordinates of the center of mass?
View SolutionTwo bodies of masses 1kg and 3kg are lying in xy plane at (0,0) and (2,−1) respectively. What are the coordinates of the centre of mass ?
Hint. xcm=m1x1+m2x2m1+x2, ycm=m1y1+m2y2m1+m2
View SolutionThree point masses of 1 kg, 2kg and 3 kg lie at (0, 0), (1, 2), (3, -1), respectively. Calculate the coordinates of the centre of mass of the system.
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo bodies of masses 0.5 kg and 1 kg are lying in the X-Y plane at points (-1, 2) and (3, 4) respectively. Locate the centre of mass of the system.
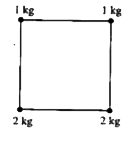
View SolutionFour point masses 1 kg, 1 kg, 2 kg and 2 kg are placed at the corners of a square of side a m as shown in Fig. Find the centre of mass of the system.
View SolutionFour particles of mass 5,3,2,4kg are at the points (1,6),(−1,5),(2,−3),(−1,−4) . Find the coordinates of their centre of mass.
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View Solution