Calculate the moment of inertia of a disc about its any diameter ?
To calculate the moment of inertia of a disc about its diameter, we can follow these steps:
Step 1: Understand the Geometry of the Disc
Consider a disc of mass m and radius r. The disc lies in the xy-plane, with the z-axis perpendicular to the plane of the disc and passing through its center.
Step 2: Use the Known Moment of Inertia
The moment of inertia of the disc about an axis perpendicular to the plane (the z-axis) is given by the formula:
Iz=12mr2
Step 3: Apply the Perpendicular Axis Theorem
The perpendicular axis theorem states that for a planar body (like our disc), the moment of inertia about an axis perpendicular to the plane (z-axis) is equal to the sum of the moments of inertia about two perpendicular axes lying in the plane (x-axis and y-axis):
Iz=Ix+Iy
Step 4: Recognize Symmetry
Since the disc is symmetric about the x-axis and y-axis, we can say:
Ix=Iy
Let’s denote the moment of inertia about the x-axis (or y-axis) as Ix. Therefore, we can rewrite the equation from the perpendicular axis theorem as:
Iz=Ix+Ix=2Ix
Step 5: Substitute the Known Value
Now, substituting the known value of Iz:
12mr2=2Ix
Step 6: Solve for Ix
To find Ix, we rearrange the equation:
Ix=12(12mr2)=14mr2
Conclusion
Thus, the moment of inertia of the disc about its diameter (either x-axis or y-axis) is:
Ix=14mr2
Consider a disc of mass m and radius r. The disc lies in the xy-plane, with the z-axis perpendicular to the plane of the disc and passing through its center.
The moment of inertia of the disc about an axis perpendicular to the plane (the z-axis) is given by the formula:
Iz=12mr2
The perpendicular axis theorem states that for a planar body (like our disc), the moment of inertia about an axis perpendicular to the plane (z-axis) is equal to the sum of the moments of inertia about two perpendicular axes lying in the plane (x-axis and y-axis):
Iz=Ix+Iy
Since the disc is symmetric about the x-axis and y-axis, we can say:
Ix=Iy
Let’s denote the moment of inertia about the x-axis (or y-axis) as Ix. Therefore, we can rewrite the equation from the perpendicular axis theorem as:
Iz=Ix+Ix=2Ix
Now, substituting the known value of Iz:
12mr2=2Ix
To find Ix, we rearrange the equation:
Ix=12(12mr2)=14mr2
Thus, the moment of inertia of the disc about its diameter (either x-axis or y-axis) is:
Ix=14mr2
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions13videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))5videos
SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE21videos
TEST 1
Similar Questions
Moment of inetia of a disc about OO' is
 View Solution
View SolutionThe moment of inertia of a uniform ring about an axis passing through its centre and perpendicular to its plane is 100kgm2. What is the moment of inertia of the ring about its diameter ?
View SolutionThe moment of inertia of a uniform ring about an axis passing through its centre and perpendicular to its plane is 100kgm2. What is the moment of inertia of the ring about its diameter ?
View SolutionCalculate the moment of inertia of a : (a)Disc about an axis passing through its edge and perpendicular to the circular base of the disc. (b) Solid sphere about an axis touching the sphere at its surface?
View SolutionCalculate the moment of inertia of a ring about a tangent to the circle of the ring normal to its plane.
View SolutionCalculate the moment of inertia of a ring about a tangent to the circle of the ring normal to its plane.
View SolutionTwo spheres of same mass and radius are in contact with each other. If the moment of inertia of a sphere about its diameter is I, then the moment of inertia of both the spheres about the tangent at their common point would be
View SolutionWhat is the moment of inertia of a circular disc about one of its diameters ?
View SolutionGiven a uniform disc of mass M and radius R. A small disc of radius R/2 is cut from this disc in such a way that the distance between the centres of the two discs is R/2. Find the moment of inertia of the remaining disc about a diameter of the original disc perpendicular to the line connecting the centres of the two discs
View SolutionCalculate the moment of inertia of the given rod about an axis CD
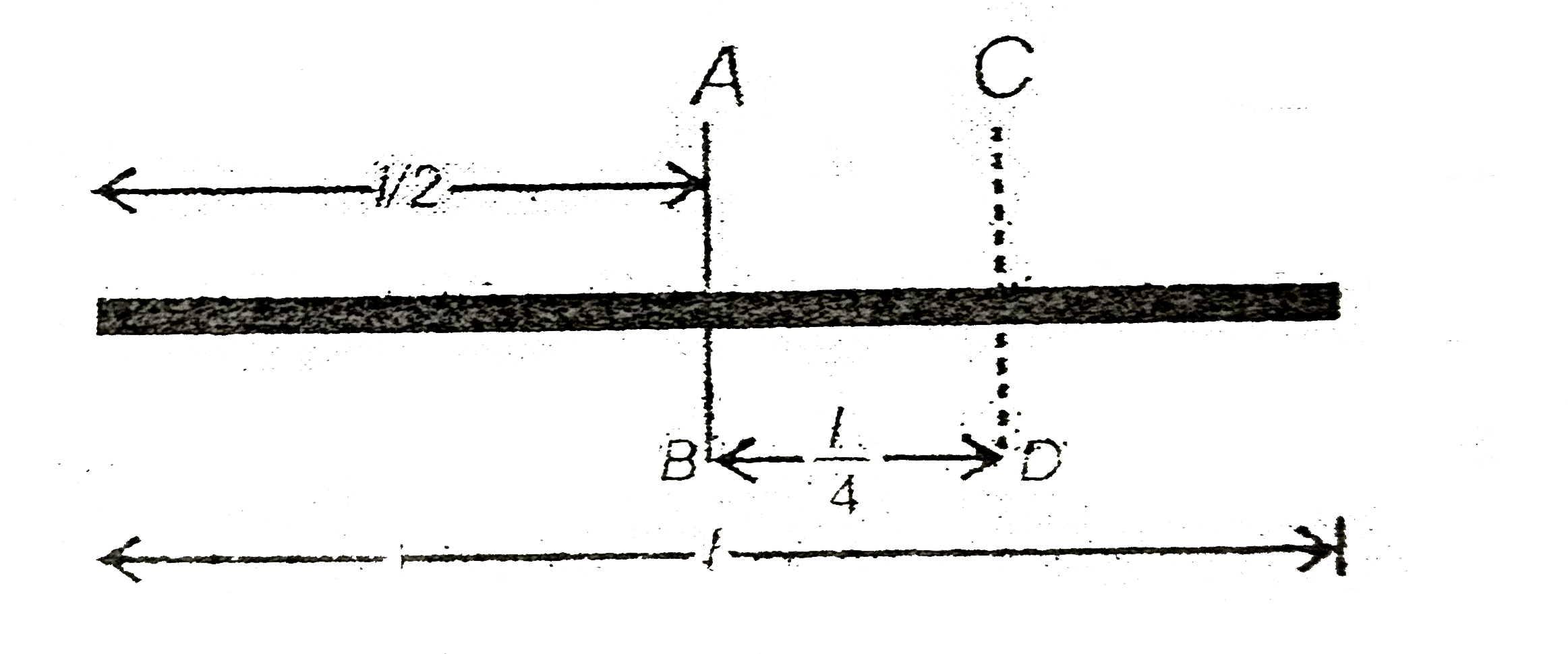 View Solution
View Solution

