A spring of spring constant k is cut in three equal pieces. The spring constant of each part will be
k3
3k
k
k6
The correct Answer is:2
To solve the problem of finding the spring constant of each part when a spring of spring constant k is cut into three equal pieces, we can follow these steps:
1. Understand the Initial Condition:
- We have a spring of length L and spring constant k.
- The relationship between the spring constant k and the length L is given by the formula:
k⋅L=constant
2. Cut the Spring:
- The spring is cut into three equal pieces. Therefore, the length of each piece becomes:
Length of each piece=L3
3. Define the New Spring Constant:
- Let the new spring constant of each piece be k′.
- According to the spring constant relationship, for each piece, we have:
k′⋅(L3)=constant
4. Set Up the Equation:
- For the original spring, the relationship can be expressed as:
k⋅L=k′⋅(L3)
5. Solve for the New Spring Constant:
- Rearranging the equation gives:
k′=k⋅LL3=k⋅3=3k
6. Conclusion:
- The spring constant of each of the three equal pieces is:
k′=3k
Final Answer:
The spring constant of each part will be 3k.
- We have a spring of length L and spring constant k.
- The relationship between the spring constant k and the length L is given by the formula:
k⋅L=constant
- The spring is cut into three equal pieces. Therefore, the length of each piece becomes:
Length of each piece=L3
- Let the new spring constant of each piece be k′.
- According to the spring constant relationship, for each piece, we have:
k′⋅(L3)=constant
- For the original spring, the relationship can be expressed as:
k⋅L=k′⋅(L3)
- Rearranging the equation gives:
k′=k⋅LL3=k⋅3=3k
- The spring constant of each of the three equal pieces is:
k′=3k
The spring constant of each part will be 3k.
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION-B )
25 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION-C )
11 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Try Yourself
86 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
A light spring of spring constant k is cut into two equal halves. Each half is connected in parallel then net spring constant of the combination is :
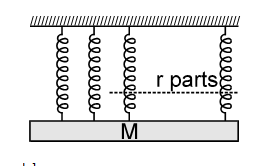
View SolutionA spring of spring constant k is cut into n equal parts, out of which r parts are placed in parallel and connected with mass M as shown in figure. The time period of oscillatory motion of mass M is:
View SolutionA spring of certain length and having spring constant k is cut into two pieces of length in a ratio 1:2. The spring constants of the two pieces are in a ratio :
View SolutionTwo springs of spring constantsK1 and K2 are joined in series. The effective spring constant of the combination is given by
View SolutionTwo springs of spring constantsK1 and K2 are joined in series. The effective spring constant of the combination is given by
View SolutionThe periodic time of a mass suspended by a spring (force constant k) is T. The spring be cut into three equal pieces. If the same mass be suspended from one piece, what will be the periodic time ?
View SolutionIf a spring has time period T, and is cut into (n) equal parts, then the time period of each part will be.
View SolutionA spring of force constant 'k' is cut into four equal parts one part is attached with a mass m. The time period of oscillation will be
View SolutionA solid sphere rolls without slipping and presses a spring of spring constant k as shown in figure. Then, the compression in the spring will be
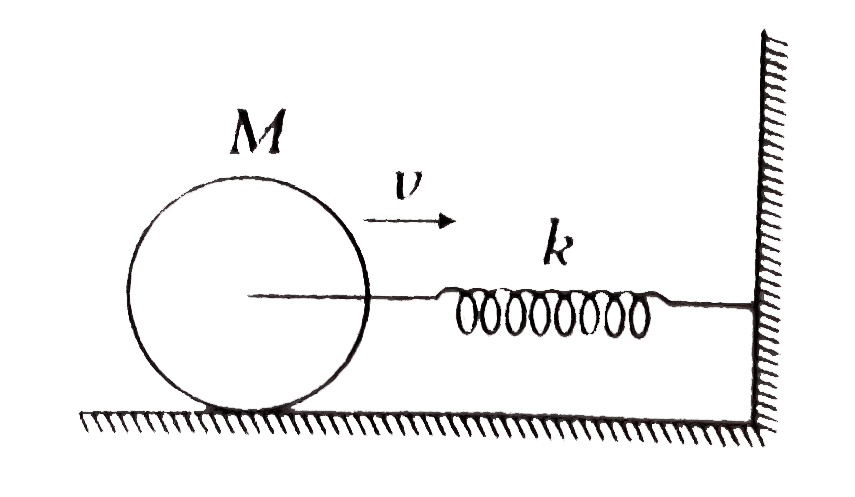
View SolutionA spring of force constant k is cut into two pieces such that one piece is double the length of the other. Then the long piece will have a force constant of
View Solution
