A particle of mass 4 kg moves along x axis, potential energy ( U ) varies with respect to x as U=20+(x−4)2 , maximum speed of paritcle is at
x=4
x=2
x=0
x=2.5
The correct Answer is:1
To solve the problem, we need to determine the position x at which the potential energy U is minimized, as this will correspond to the maximum speed of the particle.
1. Identify the potential energy function:
The potential energy U is given by the equation:
U=20+(x−4)2
2. Find the derivative of the potential energy:
To find the minimum potential energy, we need to take the derivative of U with respect to x and set it to zero:
dUdx=ddx(20+(x−4)2)
Using the chain rule, we get:
dUdx=2(x−4)
3. Set the derivative equal to zero:
To find the critical points, we set the derivative equal to zero:
2(x−4)=0
Solving for x:
x−4=0⟹x=4
4. Determine if this point is a minimum:
To confirm that this point is a minimum, we can take the second derivative of U:
d2Udx2=ddx(2(x−4))=2
Since d2Udx2=2>0, this indicates that U has a minimum at x=4.
5. Conclusion:
Since the potential energy is minimized at x=4, the kinetic energy will be maximized at this point, and therefore, the maximum speed of the particle occurs at:
x=4
Final Answer:
The maximum speed of the particle is at x=4.
The potential energy U is given by the equation:
U=20+(x−4)2
To find the minimum potential energy, we need to take the derivative of U with respect to x and set it to zero:
dUdx=ddx(20+(x−4)2)
Using the chain rule, we get:
dUdx=2(x−4)
To find the critical points, we set the derivative equal to zero:
2(x−4)=0
Solving for x:
x−4=0⟹x=4
To confirm that this point is a minimum, we can take the second derivative of U:
d2Udx2=ddx(2(x−4))=2
Since d2Udx2=2>0, this indicates that U has a minimum at x=4.
Since the potential energy is minimized at x=4, the kinetic energy will be maximized at this point, and therefore, the maximum speed of the particle occurs at:
x=4
The maximum speed of the particle is at x=4.
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION-C )
11 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION -D( Comprehension) )
3 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION -A)
58 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
A single conservative force F(x) acts on a on a (1.0kg ) particle that moves along the x-axis. The potential energy U(x) is given by:
U(x)=20+(x−2)2
where, x is meters. At x=5.0m the particle has a kinetic energy of 20J
(a) What is the mechanical energy of the system?
(b) Make a plot of U(x) as a function of x for −10m≤x≤10m, and on the same graph draw the line that represents the mechanical energy of the system. Use part (b) to determine.
(c) The least value of x and
(d) the greatest value of x between which the particle can move.
(e) The maximum kinetic energy of the particle and
(f) the value of x at which it occurs.
(g) Datermine the equation for F(x) as a function of x.
(h) For what (finite ) value of x does F(x)=0?.
View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View SolutionA particle of mass = 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is nπ5 second. Find value of n.
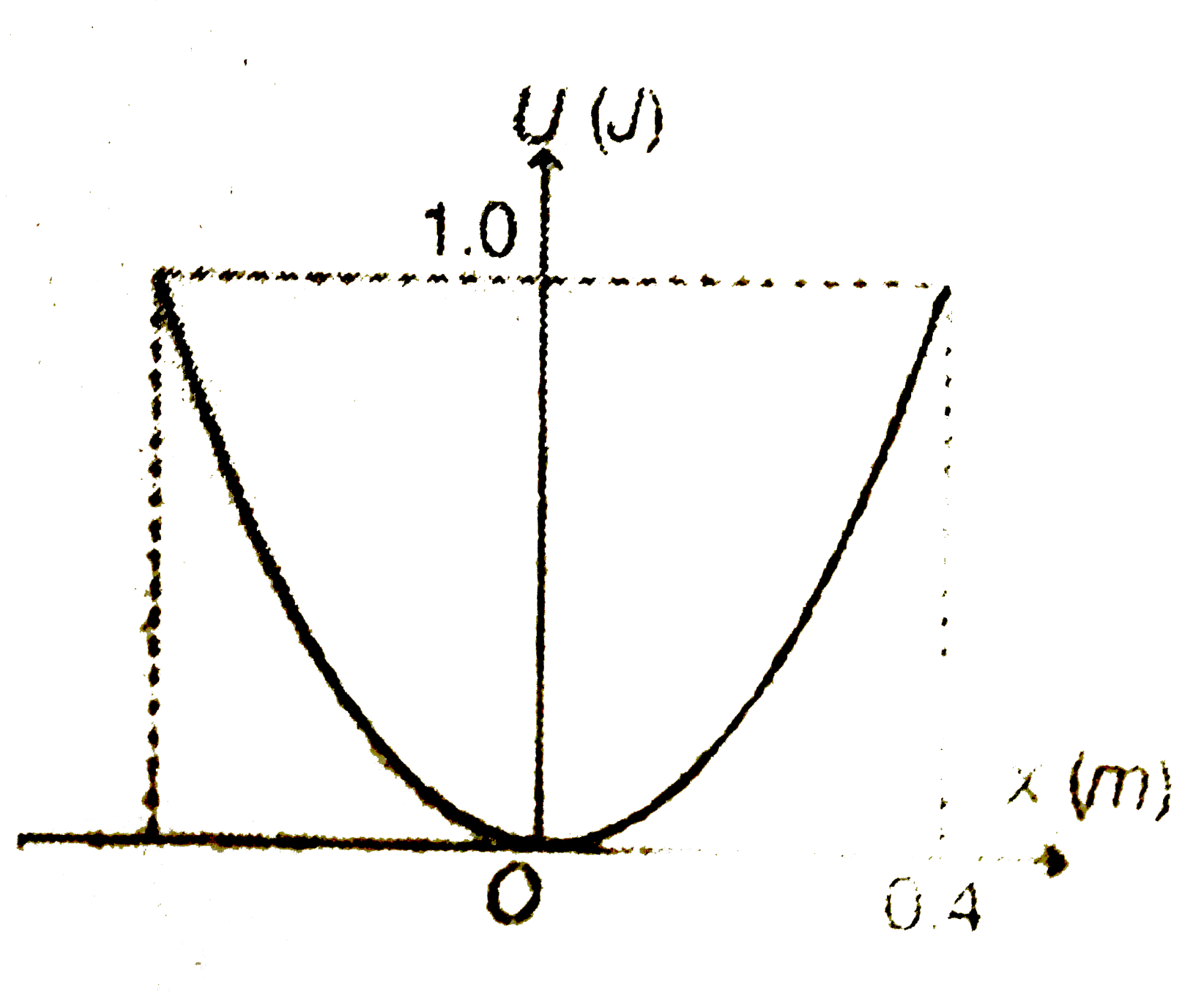
View SolutionA single conservative force F(x) acts on a 1.0−kg particle that moves along the x-axis. The potential energy U(x) is given by U(x)=20+(x−2)2 where x is in meters. At x=5.0m, the particle has a kinetic energy of 20J.
What is the mechanical energy of a system?
View SolutionThere identical point masses, each of mass 1 kg lie in the xy-plane at points (0, 0), (0, 0.2 m) and (0, 2m, 0). The net gravitational force on the mass at the origin is:
View SolutionA particle of mass m moving along x-axis has a potential energy U(x)=a+bx2 where a and b are positive constant. It will execute simple harmonic motion with a frequency determined by the value of
View SolutionA particle of mass m moving along x-axis has a potential energy U(x)=a+bx2 where a and b are positive constant. It will execute simple harmonic motion with a frequency determined by the value of
View SolutionA partical of mass 2kg is moving on the x−axis with a constant mechanical energy 20J. Its potential energy at any x is U=(16−x2)J where x is in metre. The minimum velocity of particle is :−
View SolutionA single conservative fore Fx acts on a 2kg particle that moves along the x-axis. The potential energy is given by.
U=(x−4)2−16
Here, x is in metre and U in joule. At x=6.0m kinetic energy of particle is 8J find .
(a) total mechanical energy
(b) maximum kinetic energy
(c) values of x between which particle moves
(d) the equation of Fx as a funcation is zero
(e) the value of x at which Fx is zero .
View Solution
