A uniform string of length 'L' is suspended from a point on the ceiling. A small wave pulse is now introduced at its lowest end and it starts moving up towards the ceiling.
(i) What will be the speed of the wave pulse when it has moved up a distance x?
(ii) How much time does this wave pulse take to reach the ceiling?
To solve the problem step by step, we will address each part of the question separately.
Part (i): Speed of the Wave Pulse at Distance x
1. Understanding the Setup:
- We have a uniform string of length L suspended from a ceiling. A wave pulse is introduced at the lowest end and travels upwards.
2. Identifying Relevant Variables:
- Let μ be the mass per unit length of the string.
- Let g be the acceleration due to gravity.
- The tension T in the string at a distance x from the bottom is due to the weight of the string below that point.
3. Calculating the Tension:
- The mass of the string segment below the distance x is μ⋅x.
- Therefore, the tension T at distance x is given by:
T=mass×g=μ⋅x⋅g
4. Using the Wave Speed Formula:
- The speed v of a wave on a string is given by the formula:
v=√Tμ
- Substituting the expression for tension:
v=√μ⋅x⋅gμ=√xg
5. Final Answer for Part (i):
- The speed of the wave pulse when it has moved up a distance x is:
v=√xg
Part (ii): Time Taken to Reach the Ceiling
1. Understanding the Motion:
- We need to find the time t it takes for the wave pulse to travel from the bottom of the string to the top (ceiling) over a distance L.
2. Relating Speed and Time:
- We know that speed is defined as:
v=dxdt
- From Part (i), we have v=√xg.
3. Rearranging the Equation:
- Rearranging gives:
dt=dx√xg
4. Integrating to Find Total Time:
- We need to integrate from x=0 to x=L:
t=∫L0dx√xg=1√g∫L0dx√x
- The integral ∫dx√x evaluates to 2√x:
t=1√g(2√x)L0=2√g(√L−0)=2√L√g
5. Final Answer for Part (ii):
- The time taken by the wave pulse to reach the ceiling is:
t=2√Lg
Summary of Answers:
- (i) Speed of the wave pulse when it has moved up a distance x: v=√xg
- (ii) Time taken to reach the ceiling: t=2√Lg
- We have a uniform string of length L suspended from a ceiling. A wave pulse is introduced at the lowest end and travels upwards.
- Let μ be the mass per unit length of the string.
- Let g be the acceleration due to gravity.
- The tension T in the string at a distance x from the bottom is due to the weight of the string below that point.
- The mass of the string segment below the distance x is μ⋅x.
- Therefore, the tension T at distance x is given by:
T=mass×g=μ⋅x⋅g
- The speed v of a wave on a string is given by the formula:
v=√Tμ
- Substituting the expression for tension:
v=√μ⋅x⋅gμ=√xg
- The speed of the wave pulse when it has moved up a distance x is:
v=√xg
- We need to find the time t it takes for the wave pulse to travel from the bottom of the string to the top (ceiling) over a distance L.
- We know that speed is defined as:
v=dxdt
- From Part (i), we have v=√xg.
- Rearranging gives:
dt=dx√xg
- We need to integrate from x=0 to x=L:
t=∫L0dx√xg=1√g∫L0dx√x
- The integral ∫dx√x evaluates to 2√x:
t=1√g(2√x)L0=2√g(√L−0)=2√L√g
- The time taken by the wave pulse to reach the ceiling is:
t=2√Lg
- (i) Speed of the wave pulse when it has moved up a distance x: v=√xg
- (ii) Time taken to reach the ceiling: t=2√Lg
Topper's Solved these Questions
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Try Yourself
65 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-A)
55 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Video
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)
13 Videos
Similar Questions
A uniform string of length 20m is suspended from a rigid support. A short wave pulse is introduced at its lowest end. It starts moving up the string. The time taken to reach the support is :
(take g=10ms−2)
View SolutionA uniform string of length 10 m is suspended from a rigid support A short wave pulse is introduced at its lowest end it starts moving up the string the time taken to reach the support is (take g=10m/sec2)
View SolutionA heavy but unifrom rope of length L is suspended from a celling . A particle is dropped from the celling at the instant when the bottom end is given a transverse wave pulse. Where will the particle meet the pulse.
View SolutionA heavy but uniform rope of lenth L is suspended from a ceiling. (a) Write the velocity of a transverse wave travelling on the string as a function of the distance from the lower end. (b) If the rope is given a sudden sideways jerk at the bottom, how long will it take for the pulse to reach teh celling ? (c ) A particle is dropped from the ceiling at the instant the bottom end is given the jerk where will the particle meet the pulse ?
View SolutionA wave train has the equation y=4sin(30πt+0.1x) where x is in cm is the frequency of the source? How much time does a wave-púlse take to reach a point 30 cm from it ?
View SolutionA perfectly elastic uniform string is suspended vertically with its upper end fixed to the ceiling and the lower end loaded with the weight. If a transverse wave is imparted to the lower end of the string, the pulse will
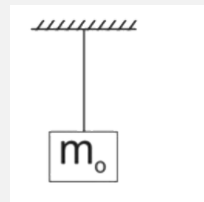
View SolutionThe block of mass m0=μL is attached to a uniform string of mass M=μL and length L as shown in the figure. If a wave pulse is produced near the block, then the time it taken to reach the ceiling is
View SolutionA uniform rope of length 12 m and mass 4 kg is hung vertically from a rigid support transverse wave pulse is produced at lower end. The speed of the wave pulse al x = 2.5 m from lower and will be kg=10 m/s)
View SolutionA uniform rope of mass M length L hangs vertically from the ceiling, with lower end free. A distbance on the rope trvelling upwards starting from the lower end has a velocity v. At a point P at distance x from the lower end.
View SolutionA uniform rope having linear density λ hangs vertically from the ceiling and its lower end is free. A disturbance produced at the free end has a speed v0 at point P midway on the rope . Then the time taken by the disturbance pulse to reach the ceiling is
View Solution