A uniform rope having linear density λ hangs vertically from the ceiling and its lower end is free. A disturbance produced at the free end has a speed v0 at point P midway on the rope . Then the time taken by the disturbance pulse to reach the ceiling is
4μ0g
2√2V0g
2√2V20λg
2√2V0λg
The correct Answer is:B
To solve the problem of determining the time taken by a disturbance pulse to reach the ceiling of a vertically hanging rope, we can follow these steps:
1. Understanding the Setup:
- We have a uniform rope with linear density λ hanging vertically from the ceiling. The lower end of the rope is free.
- A disturbance is produced at the free end of the rope, and it travels upward.
2. Identifying the Point of Interest:
- Let point P be the midpoint of the rope. The total length of the rope is L, so the distance from the free end to point P is L2.
3. Calculating Tension in the Rope:
- Consider a small segment of the rope at a distance x from the free end. The mass of this segment is λ⋅x.
- The tension T at this point due to the weight of the rope below it is given by:
T=λ⋅x⋅g
- Here, g is the acceleration due to gravity.
4. Finding the Wave Speed:
- The speed of a transverse wave in the rope is given by:
v=√Tμ
- Where μ is the linear mass density of the rope, which is λ.
- Substituting for T:
v=√λ⋅x⋅gλ=√x⋅g
5. Setting the Speed at Midpoint:
- At point P (the midpoint), the distance x is L2. The speed of the wave at this point is given as v0:
v0=√L2⋅g
- Squaring both sides:
v20=L⋅g2
- Rearranging gives:
L=2v20g
6. Setting Up the Time Calculation:
- The speed of the wave can also be expressed as:
v=dxdt
- Thus, we can write:
dt=dx√x⋅g
7. Integrating to Find Total Time:
- We need to integrate from 0 to L:
t=∫L0dx√x⋅g=1√g∫L0dx√x
- The integral ∫dx√x evaluates to 2√x:
t=1√g(2√x)L0=2√L√g
8. Substituting for L:
- Now substituting L=2v20g:
t=2√2v20g√g=2⋅√2⋅v0g
9. Final Result:
- Thus, the time taken by the disturbance pulse to reach the ceiling is:
t=2√2v0g
- We have a uniform rope with linear density λ hanging vertically from the ceiling. The lower end of the rope is free.
- A disturbance is produced at the free end of the rope, and it travels upward.
- Let point P be the midpoint of the rope. The total length of the rope is L, so the distance from the free end to point P is L2.
- Consider a small segment of the rope at a distance x from the free end. The mass of this segment is λ⋅x.
- The tension T at this point due to the weight of the rope below it is given by:
T=λ⋅x⋅g
- Here, g is the acceleration due to gravity.
- The speed of a transverse wave in the rope is given by:
v=√Tμ
- Where μ is the linear mass density of the rope, which is λ.
- Substituting for T:
v=√λ⋅x⋅gλ=√x⋅g
- At point P (the midpoint), the distance x is L2. The speed of the wave at this point is given as v0:
v0=√L2⋅g
- Squaring both sides:
v20=L⋅g2
- Rearranging gives:
L=2v20g
- The speed of the wave can also be expressed as:
v=dxdt
- Thus, we can write:
dt=dx√x⋅g
- We need to integrate from 0 to L:
t=∫L0dx√x⋅g=1√g∫L0dx√x
- The integral ∫dx√x evaluates to 2√x:
t=1√g(2√x)L0=2√L√g
- Now substituting L=2v20g:
t=2√2v20g√g=2⋅√2⋅v0g
- Thus, the time taken by the disturbance pulse to reach the ceiling is:
t=2√2v0g
Similar Questions
A uniform rope of mass M length L hangs vertically from the ceiling, with lower end free. A distbance on the rope trvelling upwards starting from the lower end has a velocity v. At a point P at distance x from the lower end.
View SolutionA Uniform rope having mass m hags vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed v of wave pulse varies with height h from the lower end as
View SolutionA uniform rope of mass m per unit length, hangs vertically from a support so that the lower end just touches the table top shown in figure. If it is released, show that at the time a length y of the rope has fallen, the force on the table is equivalent to the weight of the length 3y of the rope.
View SolutionA uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA uniform rope of mass 0.1kg and length 2.45m hangs from a ceiling.
(a) Find the speed of transverse wave in the rope at a point 0.5m distant from the lower end.
(b) Calculate the time taken by a transverse wave to travel the full length of the rope.

View SolutionA wire of variable mass per unit length μ=μ0x, is hanging from the ceiling as shown in figure. The length of wire is l0 . A small transverse disturbance is produced at its lower end. Find the time after which the disturbance will reach to the other ends.
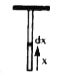
View SolutionA uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
View Solutionidentical wires A and B of different materials are hung from then ceiling of a room . The density of wire A is greater than the density of wire B but their lengths are same. Identical wave pulses are produced at the bottom of respective wires. The time taken by the pulse to reach the top is
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View Solution
