A galvanometer can withstand safely a maximum current of 5 mA. If is converted into voltmeter readding upto 20 V by connecting in series an external resistance of 3960Ω. The resistance of galvanometer is
48Ω
44Ω
36Ω
40Ω
The correct Answer is:(iv)
To find the resistance of the galvanometer (G), we can use the relationship between the total resistance in the circuit, the voltage across the voltmeter (V), and the maximum current (I_G) that the galvanometer can handle. Here are the steps to solve the problem:
1. Identify the given values:
- Maximum current (I_G) = 5 mA = 5×10−3 A
- Voltage (V) = 20 V
- External resistance (R) = 3960 Ω
2. Use the formula for total resistance in a voltmeter setup:
The total resistance (R_total) in the circuit when the galvanometer is converted into a voltmeter is given by:
Rtotal=R+G
where G is the resistance of the galvanometer.
3. Relate voltage, current, and resistance:
From Ohm's law, we know that:
V=IG×Rtotal
Rearranging gives:
Rtotal=VIG
4. Substitute the values into the equation:
Substitute V and I_G into the equation:
Rtotal=20 V5×10−3 A=200.005=4000Ω
5. Set up the equation for G:
Now we can substitute R_total back into the equation for total resistance:
Rtotal=R+G⟹4000=3960+G
6. Solve for G:
Rearranging the equation gives:
G=4000−3960=40Ω
Final Answer:
The resistance of the galvanometer (G) is 40 Ω.
---
- Maximum current (I_G) = 5 mA = 5×10−3 A
- Voltage (V) = 20 V
- External resistance (R) = 3960 Ω
The total resistance (R_total) in the circuit when the galvanometer is converted into a voltmeter is given by:
Rtotal=R+G
where G is the resistance of the galvanometer.
From Ohm's law, we know that:
V=IG×Rtotal
Rearranging gives:
Rtotal=VIG
Substitute V and I_G into the equation:
Rtotal=20 V5×10−3 A=200.005=4000Ω
Now we can substitute R_total back into the equation for total resistance:
Rtotal=R+G⟹4000=3960+G
Rearranging the equation gives:
G=4000−3960=40Ω
The resistance of the galvanometer (G) is 40 Ω.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment (Section A) Objective Type Questions (One option is correct)
55 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment (Section B) Objective Type Questions (One option is correct)
35 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Illustration
14 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
When 0.005A current flows through a moving coil galvanometer, it gives fullscale deflection. It is converted into a voltmeter to read 5 Volt, using an external resistance of 975Ω. The resistance of galvanometer in ohms is
View SolutionA galvanometer has 30 divisions and a sensitivity 16μA/div. It can be converted into a voltmeter to read 3V by connecting
View Solution
Knowledge Check
A galvanometer has a current sensitivity of 1 mA per division. A variable shunt is connected across the galvanometer and the combination is put in series with a resistance of 500Ω and cell of internal resistance 1Ω. It gives a deflection of 5 division for shunt of 5 ohm and 20 division for shunt of 25 ohm. The emf of cell is
A47.1 VB57.1 VC67.1 VD77.1 V
A moving coil galvanometer can be converted into a voltmeter by connecting :
View SolutionA galvanometer of 25 ohm resistance can read a maximum current of 6mA. It can be used as a voltmeter to measure maximum potential difference of 6V by connecting a resistance to galvanometer. Identify the correct choice from the following
View SolutionA galvanometer of resistance 25Ω gives full scale deflection for a current of 10 milliampere , is to be changed into a voltmeter of range 100 V by connecting a resistance of ‘ R ’ in series with galvanometer. The value of resistance R in Ω is
View SolutionA galvanometer of resistance 40Ω can measure a current of 10mA To convert it into a voltmeter of range 8V the required resistance is ___________ Ω.
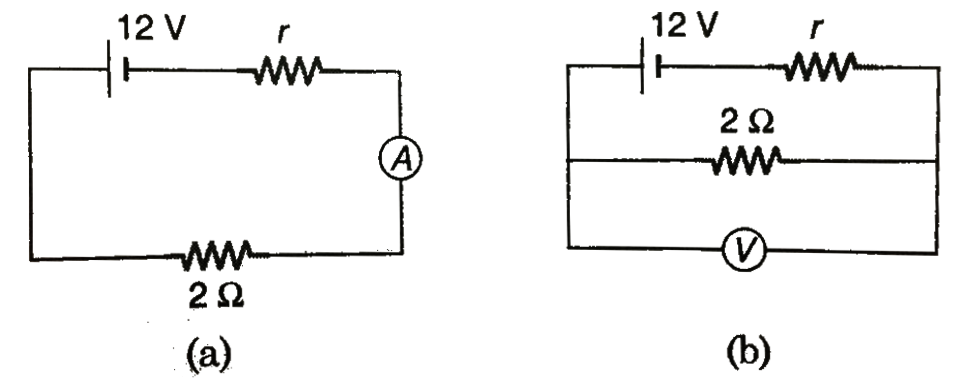
View SolutionA galvanometer (coil resistance 99Ω.) is converted into an ammeter using a shunt of 1Ω and connected as shown in figure (a). The ammeter reads 3A. The same galvanometer is convened into a voltmeter by connecting a resistance of 101Ω in series. This voltmeter is connected at, shown in figure (b). Its reading is found to be 45 of the full scale reading. Find :
(a) internal resistance r of the cell
(b) range of the ammeter and voltmeter
(c) full scale deflection current of the galvanometer.
View SolutionA galvanometer having 50 divisions provided with a variable shunt S is used to measure the current when connected in series with a resistance of 90Ω and a battery of internal resistance 10Ω. It is observed that when the shunt resistances are 10Oemga and 50Ω, the deflections are, respectively, 9 and 30 divisions. What is the resistance of the galvanometer
View SolutionA galvanometer of resistance 50Ω is converted into an ammeter by connecting a low resistance (shunt) of value 1Ω in parallel to the galvanometer, S. If full - scale deflection current of the galvanometer is 10 mA, then the maximum current that can be measured by the ammeter is -
View Solution
